Chứng minh:
1 x x + 1 + 1 x + 1 x + 2 + 1 x + 2 x + 3 + 1 x + 3 x + 4 + 1 x + 4 x + 5 + 1 x + 5
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
CM
Cao Minh Tâm
7 tháng 11 2018
Đúng(0)
Những câu hỏi liên quan
NN
1
28 tháng 2 2021
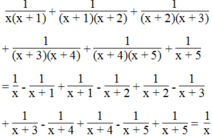
`1/(x+1)-1/(x+2)`
`=(x+2-x-1)/((x+1)(x+2))`
`=1/((x+1)(x+2))(ĐPCM)`
28 tháng 2 2021
\(\dfrac{1}{x+1}-\dfrac{1}{x+2}=\dfrac{1}{\left(x+1\right)\left(x+2\right)}\)
\(\Leftrightarrow\dfrac{x+2-x-1}{\left(x+1\right)\left(x+2\right)}=\dfrac{1}{\left(x+1\right)\left(x+2\right)}\)
\(\Leftrightarrow\dfrac{1}{\left(x+1\right)\left(x+2\right)}=\dfrac{1}{\left(x+1\right)\left(x+2\right)}\left(đpcm\right)\)
NT
1
19 tháng 6 2019
Bạn ơi đề bài sai nha mik sửa lại đề bài
\(\left(x^3-1\right)\left(x^3+1\right)=\left(x^2-1\right)\left(x^2+x+1\right)\)
VT = \(\left(x^3-1\right)\left(x^3+1\right)=\left(x^3\right)^2-1=x^6-1\)
VP = \(\left(x^2-1\right)\left(x^2+x+1\right)=\left(x^2\right)^3-1=x^6-1\)
Ta thấy VT = VP
=> \(\left(x^3-1\right)\left(x^3+1\right)=\left(x^2-1\right)\left(x^2+x+1\right)\) (đpcm)
BH
1
27 tháng 7 2017
(x^2+x+1)^2+(x-1)^2-2(x^2+x+1)(x-1)
=(x^2+x+1)^2-2(x^2+x+1)(x-1)+(x-1)^2
=[(x^2+x+1)-(x-1)]^2
=(x^2+2)^2.
TA
1
DD
Đoàn Đức Hà
Giáo viên
13 tháng 8 2021
\(\left(1-x\right)\left(1+x+x^2+...+x^{31}\right)=1-x^{32}\)
\(\left(1-x\right)\left(1+x\right)\left(1+x^2\right)\left(1+x^4\right)\left(1+x^8\right)\left(1+x^{16}\right)\)
\(=\left(1-x^2\right)\left(1+x^2\right)\left(1+x^4\right)\left(1+x^8\right)\left(1+x^{16}\right)\)
\(=\left(1-x^4\right)\left(1+x^4\right)\left(1+x^8\right)\left(1+x^{16}\right)\)
\(=\left(1-x^8\right)\left(1+x^8\right)\left(1+x^{16}\right)\)
\(=\left(1-x^{16}\right)\left(1+x^{16}\right)\)
\(=1-x^{32}\)
Ta có đpcm.
N
0
28 tháng 3 2021
Ta có: (x-x2+1)/(x-x2-1) - 1
= (x-x2+1)/(x-x2-1) - (x-x2-1)/(x-x2-1)
= (x-x2+1-x+x2+1)/(x-x2-1) = 2/(x-x2-1) = -2/(x2-x+1)
Ta có: x2-x+1 = x2-x+1/4+3/4 = (x - 1/2)2 + 3/4 > 0 với mọi x
Nên (x-x2+1)/(x-x2-1) < 1 (đpcm)
IL
1
