Cho sinx + 2cosx = -1 (π/2<x<π)
Tính sin2x
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


2.1
a.
\(\Leftrightarrow sinx-cosx=\dfrac{\sqrt{2}}{2}\)
\(\Leftrightarrow\sqrt{2}sin\left(x-\dfrac{\pi}{4}\right)=\dfrac{\sqrt{2}}{2}\)
\(\Leftrightarrow sin\left(x-\dfrac{\pi}{4}\right)=\dfrac{1}{2}\)
\(\Leftrightarrow\left[{}\begin{matrix}x-\dfrac{\pi}{4}=\dfrac{\pi}{6}+k2\pi\\x-\dfrac{\pi}{4}=\dfrac{5\pi}{6}+k2\pi\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{5\pi}{12}+k2\pi\\x=\dfrac{13\pi}{12}+k2\pi\end{matrix}\right.\)
b.
\(cosx-\sqrt{3}sinx=1\)
\(\Leftrightarrow\dfrac{1}{2}cosx-\dfrac{\sqrt{3}}{2}sinx=\dfrac{1}{2}\)
\(\Leftrightarrow cos\left(x+\dfrac{\pi}{3}\right)=\dfrac{1}{2}\)
\(\Leftrightarrow\left[{}\begin{matrix}x+\dfrac{\pi}{3}=\dfrac{\pi}{3}+k2\pi\\x+\dfrac{\pi}{3}=-\dfrac{\pi}{3}+k2\pi\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=k2\pi\\x=-\dfrac{2\pi}{3}+k2\pi\end{matrix}\right.\)

Đk: \(\left\{{}\begin{matrix}x\ne\dfrac{\pi}{2}+m2\pi\\x\ne\dfrac{\pi}{4}+n\pi\end{matrix}\right.\left(m,n\in Z\right)\)
PT \(\Leftrightarrow1=2\sqrt{2}sinx.cosx\left(sinx-cosx\right)+2cos^2x\)
\(\Leftrightarrow\sqrt{2}.2sinx.cosx\left(sinx-cosx\right)+\left(2cos^2x-1\right)=0\)
\(\Leftrightarrow\sqrt{2}sin2x\left(sinx-cosx\right)+\left(cosx-sinx\right)\left(cosx+sinx\right)=0\)
\(\Leftrightarrow\sqrt{2}sin2x=sinx+cosx\)
\(\Leftrightarrow\sqrt{2}sin2x=\sqrt{2}sin\left(x+\dfrac{\pi}{4}\right)\)
\(\Leftrightarrow\left[{}\begin{matrix}2x=x+\dfrac{\pi}{4}+k2\pi\\2x=\pi-x-\dfrac{\pi}{4}+k2\pi\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{\pi}{4}+k2\pi\\x=\dfrac{\pi}{4}+k\dfrac{2\pi}{3}\end{matrix}\right.\left(k\in Z\right)\)

Chọn A.
Ta có:
sinx + cosx = ½ nên ( sinx + cosx)2 = ¼
Do đó sinx. cosx = -3/8
Khi đó sinx; cosx là nghiệm của phương trình
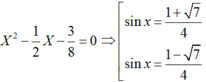
Ta có sinx + cos x = ½ nên 2( sinx + cosx) = 1
+) Với 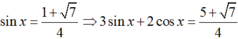
+) Với 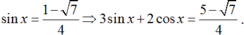

Chọn A.
Từ giả thiết ta suy ra: (sinx+ cosx) 2 = ¼
Suy ra: 2sinx.cosx = -3/4 hay sinx.cosx = -3/8
Khi đó sinx; cosx là nghiệm của phương trình
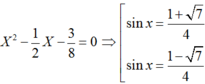
Do sinx + cosx = ½ nên 2(sinx + cosx) = 1
+) Với 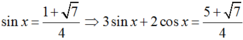
+) Với 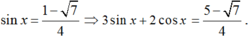

\(1+2\sin x.\cos x=\sin x+2\cos x\)
\(\Leftrightarrow\left(2\sin x.\cos x-\sin x\right)-\left(2\cos x-1\right)=0\)
\(\Leftrightarrow\left(2\cos x-1\right)\left(\sin x-1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}\cos x=\dfrac{1}{2}\\\sin x=1\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=\pm\dfrac{\pi}{3}+k2\pi\\x=\dfrac{\pi}{2}+k2\pi\end{matrix}\right.\left(k\in Z\right)\)

\(sin\left(x-\dfrac{\pi}{2}\right)+cos\left(x-\pi\right)+tan\left(\dfrac{5\pi}{2}-x\right)+tan\left(x-\dfrac{\pi}{2}\right)\)
\(=-sin\left(\dfrac{\pi}{2}-x\right)+cos\left(\pi-x\right)+tan\left(2\pi+\dfrac{\pi}{2}-x\right)-tan\left(\dfrac{\pi}{2}-x\right)\)
\(=-cosx-cosx+tan\left(\dfrac{\pi}{2}-x\right)-cotx\)
\(=-2cosx+cotx-cotx=-2cosx\)

ta có : \(\left(2cosx-1\right)\left(sinx+cosx\right)=1\)
\(\Leftrightarrow2cosx.sinx+2cos^2x-1=sinx+cosx\)
\(\Leftrightarrow sin2x+cos2x=sinx+cosx\)
\(\Rightarrow2x=x\Leftrightarrow x=0\) vậy \(x=0\)
\(\frac{\pi}{2}< x< \pi\Rightarrow cosx\ne0\)
\(\left(sinx+2cosx\right)^2=1\Rightarrow sin^2x+4cos^2x+4sinx.cosx=1\)
\(\Rightarrow3cos^2x+4sinx.cosx=0\)
\(\Rightarrow3cosx+4sinx=0\)
Kết hợp điều kiện ban đầu ta được:
\(\left\{{}\begin{matrix}sinx+2cosx=-1\\4sinx+3cosx=0\end{matrix}\right.\) \(\Rightarrow\left\{{}\begin{matrix}sinx=\frac{3}{5}\\cosx=-\frac{4}{5}\end{matrix}\right.\)
\(\Rightarrow sin2x=2sinx.cosx=-\frac{24}{25}\)