K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
TK
2
PN
4
PL
4 tháng 8 2021
a) |x - 1,7| = 2,3
=> x - 1,7 = 2,3 hoặc x - 1,7 = -2,3
=> x = 4 hoặc x = -0,6
b) |x + 3/4| - 1/3 = 0
=> |x + 3/4| = 1/3
=> x + 3/4 = 1/3 hoặc x + 3/4 = -1/3
=> x = -5/12 hoặc x = -13/12
Chúc e học tốt !
V
1
19 tháng 1 2020
Đặt: \(A=|x-a|+|x-b|+|x-c|+|x-d|\)
Đặt: \(B=|x-a|+|x-d|\)
Ta có: \(B=|x-a|+|x-d|=|x-a|+|d-x|\)
Và: \(B\ge|x-a+d-x|=d-a\)
\(\Rightarrow Min_B=d-a\)
Đạt được \(\Leftrightarrow\left(x-a\right)\left(d-x\right)\ge0\)
Giải ta được: \(a\le x\le d\left(1\right)\)
Đặt \(C=|x-b|+|x-c|\)
\(C=|x-b|+|c-x|\ge|x-b+c-x|\)
\(\Rightarrow C\ge c-b\)
\(\Rightarrow Min_C=c-b\Leftrightarrow\left(x-b\right)\left(c-x\right)\ge0\)
Giải ra được: \(b\le x\le x\left(2\right)\)
Từ \(\left(1\right)\left(2\right)\Rightarrow Min_A=d-a+c-b\)
Dấu " = " xảy ra \(\Leftrightarrow b\le x\le c\)
20 tháng 7 2021
a, Ta có
\(\left|x-1,7\right|=2,3\\ \Rightarrow\left[{}\begin{matrix}x-1,7=2.3\\x-1.7=-2,3\end{matrix}\right.\Rightarrow\left[{}\begin{matrix}x=4\\x=-0,6\end{matrix}\right.\)
Vậy....
b, Ta có :
\(\left|x+\dfrac{3}{4}\right|-\dfrac{1}{3}=0\\ \Rightarrow\left|x+\dfrac{3}{4}\right|=\dfrac{1}{3}\\ \Rightarrow\left[{}\begin{matrix}x+\dfrac{3}{4}=\dfrac{1}{3}\\x+\dfrac{3}{4}=-\dfrac{1}{3}\end{matrix}\right.\Rightarrow\left[{}\begin{matrix}x=-\dfrac{5}{12}\\x=-\dfrac{13}{12}\end{matrix}\right.\)
Vậy...
LM
2
YN
20 tháng 9 2021
\(B=\left|2x+3,5\right|+\left|2x+\frac{7}{2}\right|\)
\(=\left|3,5-2x\right|+\left|2x+3,5\right|\ge\left|3,5-2x+2x+3,5\right|=7\)
Dấu '' = '' xảy ra khi \(\left(3,5-2x\right)\left(2x+3,5\right)\ge0\)
\(\Rightarrow\orbr{\begin{cases}3,5-2x\ge0;2x+3,5\ge0\\3,5-2x\le0;2x+3,5\le0\end{cases}}\)
\(\Rightarrow\orbr{\begin{cases}2x\le3,5;2x\ge-3,5\\2x\ge3,5;2x\le-3,5\end{cases}}\)
\(\Rightarrow\orbr{\begin{cases}x\le1,75;x\ge-1,75\Rightarrow-1,75\le x\le1,75\\x\ge1,75;x\le-1,75\text{(Vô lý)}\end{cases}}\)
Vậy \(MinB=7\Leftrightarrow-1,75\le x\le1,75\)
10 tháng 10 2021
\(A=\dfrac{1}{2}\left(x-3\right)^2+10\ge10\\ A_{min}=10\Leftrightarrow x-3=0\Leftrightarrow x=3\)
10 tháng 10 2021
\(A=\dfrac{1}{2}\left(x-3\right)^2+10\ge10\forall x\)
Dấu '=' xảy ra khi x=3
15 tháng 2 2019
a) |x - 1,7| = 2,3
Xét 2 trường hợp:
TH1: x - 1,7 = -2,3
x = -2,3 +1,7
x = -0,6
TH2: x - 1,7 = 2,3
x = 2,3 + 1,7
x = 4
Vậy: Tự kl :<
25 tháng 7 2021
a, \(-\dfrac{2}{3}+\left|\dfrac{1}{2}x-3\right|\ge-\dfrac{2}{3}\)
Dấu ''='' xảy ra khi x = 6
Vậy GTNN biểu thức trên là -2/3 khi x = 6
b, \(1,6-\left|2x-1\right|\le1,6\)
Dấu ''='' xảy ra khi x = 1/2
Vậy GTLN biểu thức trên là 1,6 khi x = 1/2
25 tháng 7 2021
a) Ta có: \(\left|\dfrac{1}{2}x-3\right|\ge0\forall x\)
\(\Leftrightarrow\left|\dfrac{1}{2}x-3\right|-\dfrac{2}{3}\ge-\dfrac{2}{3}\forall x\)
Dấu '=' xảy ra khi x=6
b) Ta có: \(\left|2x-1\right|\ge0\)
\(\Leftrightarrow-\left|2x-1\right|\le0\forall x\)
\(\Leftrightarrow-\left|2x-1\right|+1.6\le1.6\forall x\)
Dấu '=' xảy ra khi \(x=\dfrac{1}{2}\)
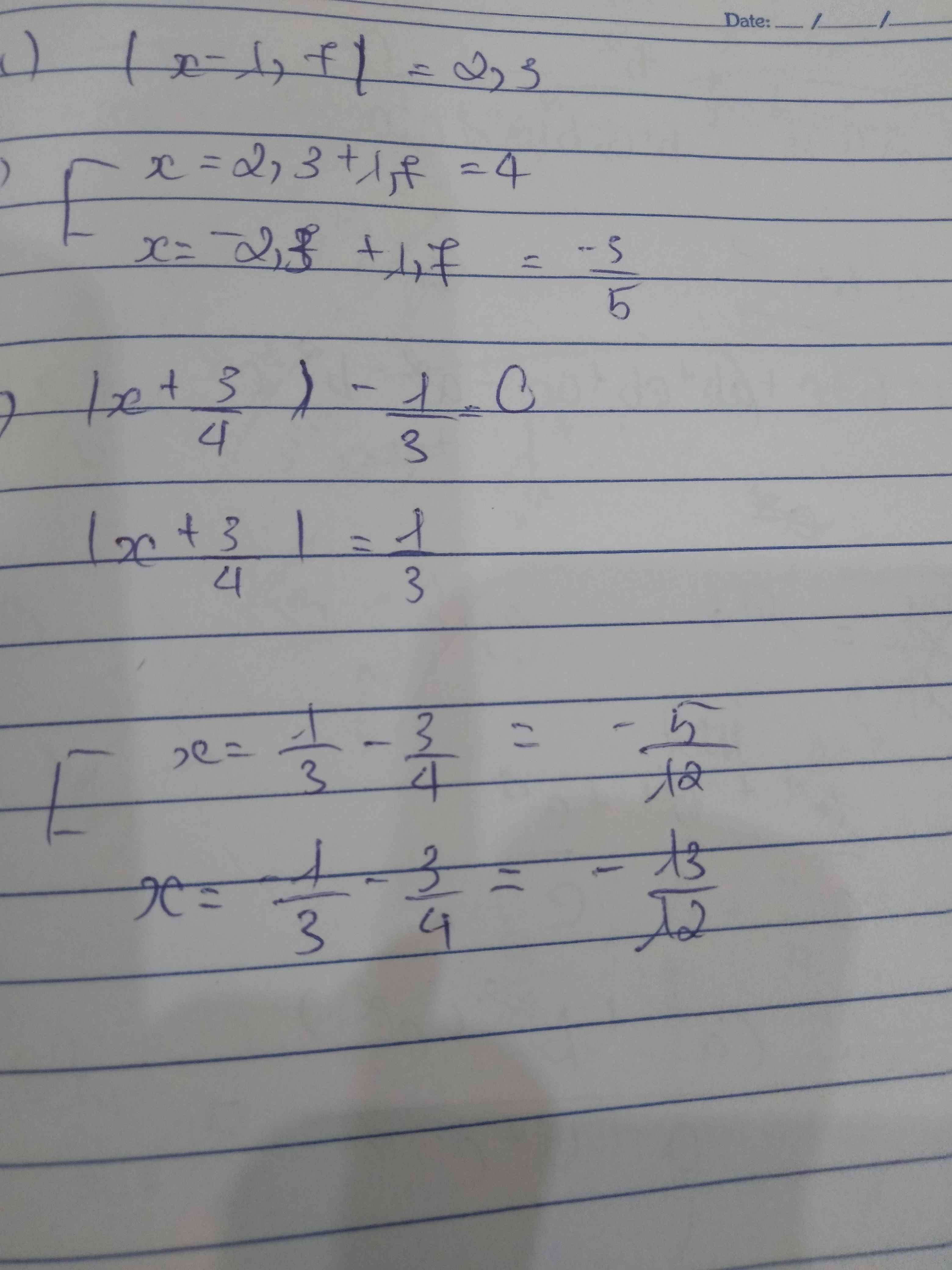
Cái này phải là GTLN
\(A=2,3-\left|x-1,7\right|\le2,3\)
\(maxA=2,3\Leftrightarrow x=1,7\)