Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

![]() xác định khi:
xác định khi:
(x + y)(6x – 6y)
≠
0 ⇒ 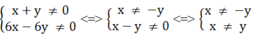
Điều kiện x ≠ ± y
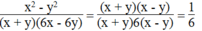
Vậy biểu thức không phụ thuộc vào x, y.

a)\(\left(x+4\right)\left(x^2-4x+16\right)-x^3+5=x^3+64-x^3+5=69\)
Vậy biểu thức trên ko phụ thuộc vào biến x .
b)\(y\left(x^2-y^2\right)\left(x^2+y^2\right)-y\left(x^4-y^4\right)=y\left(x^4-y^4\right)-y\left(x^4-y^4\right)=0\)
Vậy biểu thức trên ko phụ thuộc vào biến x .

\(a,-x^3+\left(x-3\right)\left[\left(2x+1\right)^2-2\left(\dfrac{3}{2}x^2+\dfrac{1}{2}x-4\right)\right]\\ =-x^3+\left(x-3\right)\left(4x^2+4x+1-3x^2-x+8\right)\\ =-x^3+\left(x-3\right)\left(x^2+3x+9\right)\\ =-x^3+\left(x^3-27\right)=-27\)
\(b,\left(x+2y\right)^3-\left(x-3y\right)\left(x^2+3xy+9y^2\right)-6y\left(x^2+2xy-\dfrac{35}{6}y^2\right)\\ =x^3+6x^2y+12xy^2+8y^3-x^3+27y^3-6x^2y-12xy^2+35y^3\\ =0\)

Bài 2:
\(A=\left(x+y\right)^3-3xy\left(x+y\right)+3xy=1^3-3xy+3xy=1\)
Bài 3:
\(M=x^6-x^4-x^4+x^2+x^3-x\)
\(=x^3\left(x^3-x\right)-x\left(x^3-x\right)+\left(x^3-x\right)\)
\(=8x^3-8x+8\)
\(=8\cdot8+8=72\)

⇒ 2x(2a + 3) + 3y(2a + 3) = (2a + 3)(2x + 3y) ≠ 0
Ta có: 2a + 3 ≠ 0 ⇒ a ≠ - 3/2 ; 2x + 3y ≠ 0 ⇒ x ≠ - 3/2 y
Điều kiện: x ≠ - 3/2 y và a ≠ - 3/2
Vậy biểu thức không phụ thuộc vào x, y.