Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

a: Ta có: ΔABC vuông tại A
nên \(\widehat{B}+\widehat{C}=90^0\)
hay \(\widehat{B}=55^0\)
Xét ΔABC vuông tại A có
\(AC=BC\cdot\sin55^0\)
\(\Leftrightarrow AC\simeq3.69\left(cm\right)\)
\(\Leftrightarrow AB\simeq2.58\left(cm\right)\)

a: Xét ΔABC vuông tại A có
\(BC^2=AB^2+AC^2\)
hay \(BC=\dfrac{9\sqrt{34}}{10}\left(cm\right)\)
Xét ΔABC vuông tại A có
\(\sin\widehat{B}=\dfrac{AC}{BC}=\dfrac{5\sqrt{34}}{34}\)
\(\Leftrightarrow\widehat{B}\simeq59^0\)
\(\Leftrightarrow\widehat{C}=21^0\)

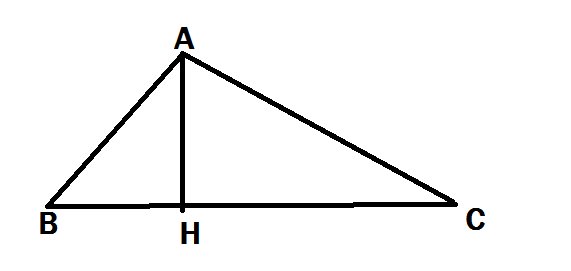
\(\dfrac{1}{AH^2}=\dfrac{1}{AB^2}+\dfrac{1}{AC^2}\)
\(\Leftrightarrow\dfrac{1}{9}=\dfrac{1}{AB^2}+\dfrac{1}{25}\)
\(\Leftrightarrow\dfrac{1}{AB^2}=\dfrac{16}{225}\)
\(\Leftrightarrow AB=\dfrac{15}{4}\)
- \(AH.BC=AB.AC\)
\(3.BC=\dfrac{15}{4}.5\)
\(BC=6,25\)
- \(CH=\dfrac{AC^2}{BC}=4\)
=> BH = 6,25 - 4 = 2,25

Ta có:
\(AB+BC=AC\sqrt{3}\)
=> \(\frac{AC}{BC}\sqrt{3}-\frac{AB}{BC}=1\)
=> \(\sqrt{3}\cos\widehat{C}-\sin\widehat{C}=1\)
=> \(\sqrt{3}\cos\widehat{C}-1=\sin\widehat{C}\)
Mặt khác: \(\sin^2\widehat{C}+\cos^2\widehat{C}=1\)
<=> \(\left(\sqrt{3}\cos\widehat{C}-1\right)^2+\cos^2\widehat{C}=1\)
<=> \(4\cos^2\widehat{C}-2\sqrt{3}\cos\widehat{C}=0\)( \(0^o< \widehat{C}< 90^o\))
<=> \(2\cos\widehat{C}-\sqrt{3}=0\)
<=> \(\cos\widehat{C}=\frac{\sqrt{3}}{2}\)
<=> \(\widehat{C}=30^o\)=> \(\widehat{B}=60^o\)