
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.




Vì (2x-4). F(x) = (x-1).F(x+1) với mọi x nên
+) Khi x=2 thì 0.F(2) = 1.F(3) => F(3) = 0
Vậy x=3 là một nghiệm của F(x).
+) Khi x = 1 thì -2F(1) = 0.F(2) => F(1) = 0
Vậy x = 1 là một nghiệm của F(x)
Do đó F (x) có ít nhất hai nghiệm là 3 và 1.
~ Chúc b học tốt nhaa~

\(81^7-27^9-9^{13}=\left(3^4\right)^7-\left(3^3\right)^9-\left(3^2\right)^{13}=3^{28}-3^{27}-3^{26}=3^{26}\left(3^2-3-1\right)=3^{24}.3^2.5=3^{24}.45⋮45\)

a) \(f\left(x\right)-g\left(x\right)=\left[x\left(x^2-2x+7\right)-1\right]-\left[x\left(x^2-2x-1\right)-1\right]\)
\(f\left(x\right)-g\left(x\right)=x^3-2x^2+7x-1-x^3+2x^2+x+1\)
\(f\left(x\right)-g\left(x\right)=8x\)
\(f\left(x\right)+g\left(x\right)=x\left(x^2-2x+7\right)-1+x\left(x^2-2x-1\right)-1\)
\(f\left(x\right)+g\left(x\right)=x^3-2x^2+7x-1+x^3-2x^2-x-1\)
\(f\left(x\right)+g\left(x\right)=2x^3-4x^2+6x-2\)
b) 8x=0
=> x=0
=> Nghiệm đa thức f(x)-g(x)
c) Thay \(x=-\frac{3}{2}\)vào BT f(x)+g(x) ta được :
\(2.\left(-\frac{3}{2}\right)^3-4\left(-\frac{3}{2}\right)^2+6\left(-\frac{3}{2}\right)-2\)
\(=6,75+9-9-2\)
\(=4,75\)
#H

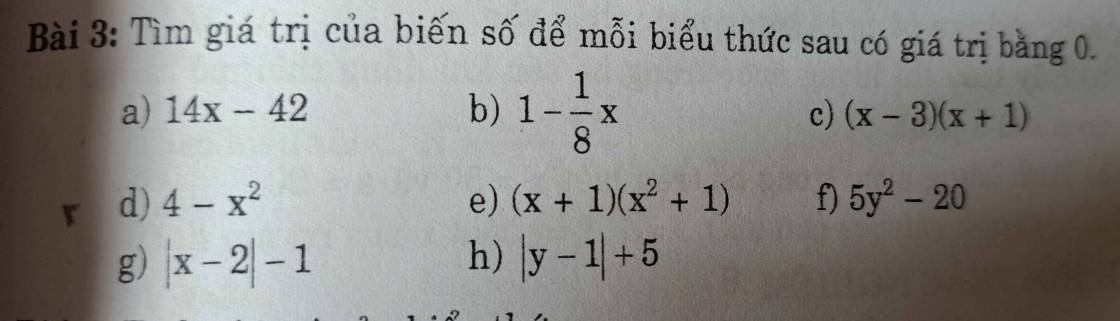




f: 5y^2-20=0
=>y^2-4=0
=>(y-2)(y+2)=0
=>y=2 hoặc y=-2
g: |x-2|-1=0
=>|x-2|=1
=>x-2=1 hoặc x-2=-1
=>x=3 hoặc x=1
Em cảm ơn ạ