Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

\(\Leftrightarrow2y^3-6y^2+7y-3=-2x\sqrt{1-x}+2\sqrt{1-x}+\sqrt{1-x}\)
\(\Leftrightarrow2\left(y^3-3y^2+3y+1\right)+y-1=2\left(1-x\right)\sqrt{1-x}+\sqrt{1-x}\)
\(\Leftrightarrow2\left(y-1\right)^3+y-1=2\left(\sqrt{1-x}\right)^3+\sqrt{1-x}\) (1)
Xét hàm \(f\left(t\right)=2t^3+t\)
\(f'\left(t\right)=6t^2+1>0\Rightarrow f\left(t\right)\) đồng biến
Nên (1) tương đương: \(y-1=\sqrt{1-x}\Rightarrow y=1+\sqrt{1-x}\)
\(\Rightarrow P=x+2\sqrt{1-x}+2=-\left(1-x-2\sqrt{1-x}+1\right)+4=-\left(\sqrt{1-x}-1\right)^2+4\le4\)
⇒ P = x + 2 √ 1 − x + 2
= − ( 1 − x − 2 √ 1 − x + 1 ) + 4
= − ( √ 1 − x − 1 ) 2 + 4 ≤ 4
Cho xin một like đi các dân chơi à.


\(4=2^x+2^y\ge2\sqrt{2^{x+y}}\Rightarrow2^{x+y}\le4\Rightarrow x+y\le2\)
\(\Rightarrow xy\le1\)
\(P=4x^2y^2+2x^3+2y^3+10xy\)
\(P=4x^2y^2+10xy+2\left(x+y\right)\left[\left(x+y\right)^2-3xy\right]\)
\(P\le4x^2y^2+10xy+4\left(4-3xy\right)=4x^2y^2-2xy+16\)
Đặt \(xy=t\Rightarrow0< t\le1\)
Xét hàm \(f\left(t\right)=4t^2-2t+16\) trên \((0;1]\)
\(\Rightarrow...\)

Đáp án C.
Ta có: GT
<=> 5x+2y + x + 2y – 3–x–2y = 5xy–1 – 31–xy + xy – 1.
X é t h à m s ố f t = 5 t + t - 3 - t
⇒ f t = 5 t ln 5 + 1 + 3 - t ln 3 > 0 ∀ t ∈ ℝ
Do đó hàm số đồng biến trên ℝ suy ra
f(x+2y) = f(xy – 1) <=> x+ 2y = xy – 1
⇔ x = 2 y + 1 y - 1 ⇒ T = 2 y + 1 y - 1 + y .
Do x > 0 => y > 1.
Ta có:
T = 2 + y + 3 y - 1 = 3 + y - 1 + 3 y - 1 ≥ 3 + 2 3 .

Do \(x;y\in\left[0;2\right]\Rightarrow\left\{{}\begin{matrix}x\left(2-x\right)\ge0\\y\left(2-y\right)\ge0\end{matrix}\right.\) \(\Rightarrow2x^2+4y^2\le4x+8y\)
\(P\le3^0+5^0+3^z+4\left(x+2y\right)=2+3^z+4\left(6-z\right)=3^z-4z+26\)
Xét hàm \(f\left(z\right)=3^z-4z+26\) trên \(\left[0;2\right]\)
\(f'\left(z\right)=3^z.ln3-4=0\Rightarrow z=log_3\left(\dfrac{4}{ln3}\right)=a\)
\(f\left(0\right)=27\) ; \(f\left(2\right)=27\); \(f\left(a\right)\approx-1,1\)
\(\Rightarrow f\left(z\right)\le27\Rightarrow maxP=27\)
(Dấu "=" xảy ra khi \(\left(x;y;z\right)=\left(0;2;2\right)\))
Ồ mà khoan, bài trước bị nhầm lẫn ở chỗ \(3^{2x-x^2}+5^{2y-y^2}\ge3^0+5^0\) mới đúng, ko để ý bị ngược dấu đoạn này
Vậy giải cách khác:
\(0\le x;y;z\le2\Rightarrow x\left(2-x\right)\ge0\Rightarrow2x-x^2\ge0\)
Lại có: \(2x-x^2=1-\left(x-1\right)^2\le1\)
\(\Rightarrow0\le2x-x^2\le1\)
Tương tự ta có: \(0\le2y-y^2\le1\)
Xét hàm: \(f\left(t\right)=3^t-2t\) trên \(\left[0;1\right]\)
\(f'\left(t\right)=3^t.ln3-2=0\Rightarrow t=log_3\left(\dfrac{2}{ln3}\right)=a\)
\(f\left(0\right)=1;\) \(f\left(1\right)=1\) ; \(f\left(a\right)\approx0,73\)
\(\Rightarrow f\left(t\right)\le1\Rightarrow3^t-2t\le1\Rightarrow3^t\le2t+1\)
\(\Rightarrow3^{2x-x^2}\le2\left(2x-x^2\right)+1\)
Hoàn toàn tương tự, ta chứng minh được:
\(5^t\le4t+1\) với \(t\in\left[0;1\right]\Rightarrow5^{2y-y^2}\le4\left(2y-y^2\right)+1\)
\(3^t\le4t+1\) với \(t\in\left[0;2\right]\Rightarrow3^z\le4z+1\)
\(\Rightarrow P\le2\left(2x-x^2\right)+4\left(2y-y^2\right)+4z+3+2x^2+4y^2=4\left(x+2y+z\right)+3=27\)
Lần này thì ko sai được rồi

\(a^2+b^2+6ab+2=2a+3b\Rightarrow\left(a+b\right)^2-3\left(a+b\right)+2=-a\left(4b+1\right)\le0\)
\(\Rightarrow\left(a+b-1\right)\left(a+b-2\right)\le0\Rightarrow1\le a+b\le2\)
\(a^2+b^2+6ab+2=2a+3b\Rightarrow4ab=-\left(a+b\right)^2+2a+3b-2\)
\(-P=\dfrac{6a+5b+4ab+7}{a+b+1}=\dfrac{6a+5a+7-\left(a+b\right)^2+2a+3b-2}{a+b+1}\)
\(=\dfrac{-\left(a+b\right)^2+8\left(a+b\right)+5}{a+b+1}\)
Tới đây có thể giải theo lớp 9 (tách thành tích hoặc bình phương) hoặc làm theo lớp 12 (khảo sát hàm \(f\left(x\right)=\dfrac{-x^2+8x+5}{x+1}\) trên \(\left[1;2\right]\)). Cả 2 việc đều dễ dàng cả
\(-P=6-\dfrac{\left(x-1\right)^2}{x+1}=\dfrac{17}{3}+\dfrac{\left(3x-1\right)\left(2-x\right)}{3\left(x+1\right)}\)

Đáp án B.
Từ giả thiết, suy ra
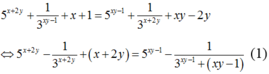
Xét hàm số f ( t ) = 5 t - 1 3 t + t trên ℝ .
Đạo hàm f ' ( t ) = 5 t . ln 5 - ln 3 3 t + 1 > 0 , ∀ t ∈ ℝ ⇒ hàm số f ( t ) luôn đồng biến trên ℝ .
Suy ra
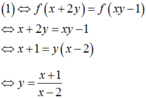
Do y > 0 nên x + 1 x - 2 > 0 ⇔ [ x > 2 x < - 1 . Mà x > 0 nên x > 2 .
Từ đó T = x + y = x + x + 1 x - 2 . Xét hàm số g ( x ) = x + x + 1 x - 2 trên 2 ; + ∞ .
Đạo hàm
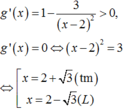
Lập bảng biến thiên của hàm số trên 2 ; + ∞ , ta thấy min g ( x ) = g ( 2 + 3 ) = 3 + 2 3 .
Vậy T m i n = 3 + 2 3 khi x = 2 + 3 và x = 1 + 3 .
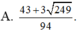
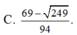
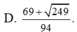
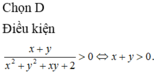






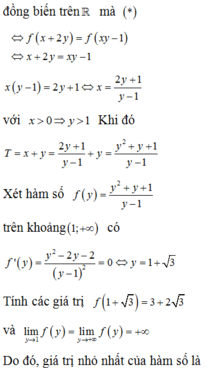

Chọn C