Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


d' song song d nên có dạng: \(x-2y+c=0\)
Gọi \(A\left(0;1\right)\) là 1 điểm thuộc d
\(\Rightarrow d\left(A;d'\right)=\sqrt{5}\)
\(\Leftrightarrow\frac{\left|0.1-2.1+c\right|}{\sqrt{1^2+\left(-2\right)^2}}=\sqrt{5}\)
\(\Leftrightarrow\left|c-2\right|=5\Rightarrow\left[{}\begin{matrix}c=7\\c=-3\end{matrix}\right.\)
Có 2 đường thẳng thỏa mãn: \(\left[{}\begin{matrix}x-2y+7=0\\x-3y-3=0\end{matrix}\right.\)


Gọi M là trung điểm AB \(\Rightarrow M\left(3;-\dfrac{1}{2}\right)\) ; \(\overrightarrow{AB}=\left(2;1\right)\) \(\Rightarrow AB=\sqrt{5}\)
Có 2 trường hợp có thể xảy ra:
TH1: d đi qua M và cách AB một khoảng \(\sqrt{5}\)
Nhưng theo định lý đường xiên - đường vuông góc, ta luôn có \(d\left(A;d\right)=d\left(B;d\right)\le AM=\dfrac{\sqrt{5}}{2}< \sqrt{5}\)
Nên ko tồn tại đường thẳng d thỏa mãn TH1
TH2: d song song AB và cách đường thẳng AB 1 khoảng \(\sqrt{5}\)
\(\Rightarrow\) d nhận (1;-2) là 1 vtpt nên pt có dạng: \(x-2y+c=0\)
\(d\left(B;d\right)=\sqrt{5}\Leftrightarrow\dfrac{\left|4+c\right|}{\sqrt{1^2+\left(-2\right)^2}}=\sqrt{5}\Leftrightarrow\left|c+4\right|=5\)
\(\Rightarrow\left[{}\begin{matrix}c=1\\c=-9\end{matrix}\right.\) \(\Rightarrow\) pt d: \(\left[{}\begin{matrix}x-2y+1=0\\x-2y-9=0\end{matrix}\right.\)

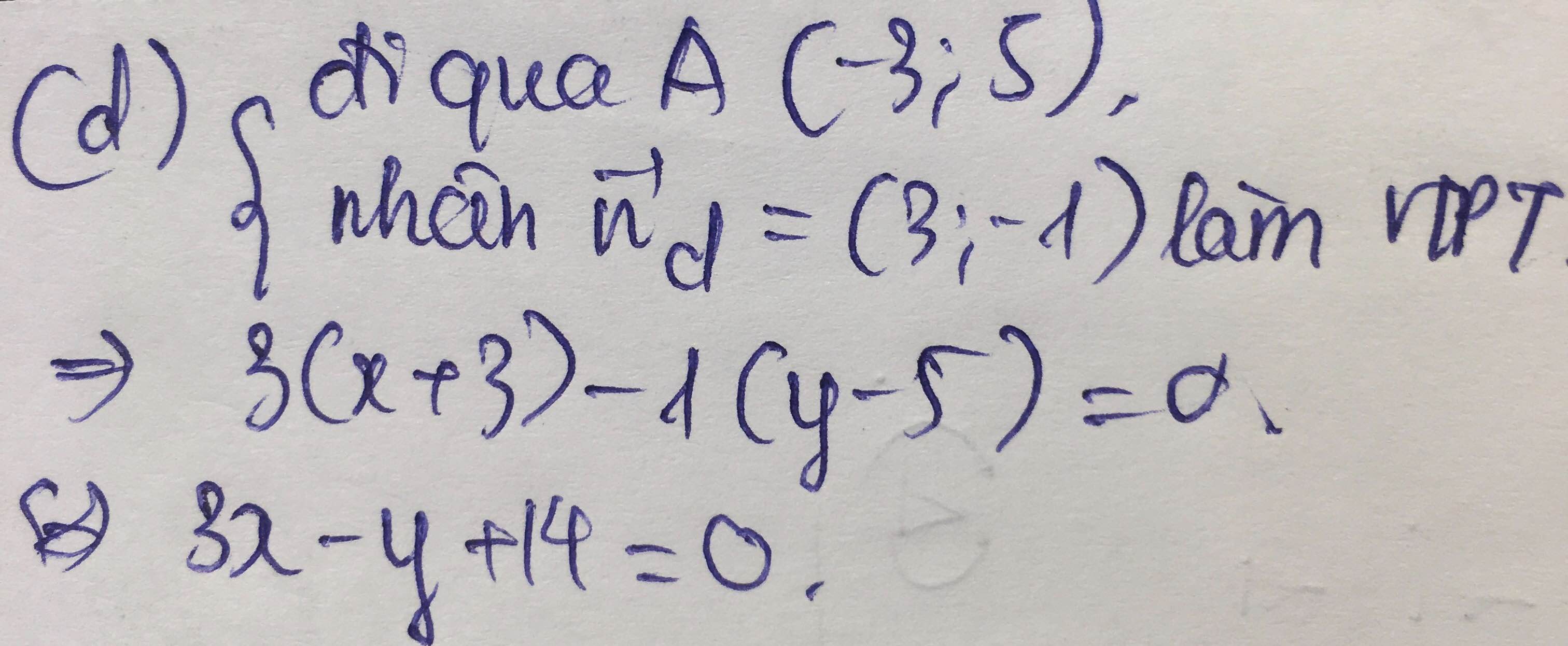
NX: \(\dfrac{2}{4}\)=\(\dfrac{-1}{-2}\)≠\(\dfrac{-2}{6}\)
=> (d) // (d')
Ta lấy điểm A(0;-2) ∈ d
d(d;d') = \(\dfrac{\left|4.0-2.\left(-2\right)+6\right|}{\sqrt{4^2+2^2}}\) = \(\sqrt{5}\)
=> Chọn C