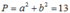Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

\(\lim\limits_{x\rightarrow0}\frac{\sqrt{3x+1}-1}{x}=\lim\limits_{x\rightarrow0}\frac{3x}{x\left(\sqrt{3x+1}+1\right)}=\lim\limits_{x\rightarrow0}\frac{3}{\sqrt{3x+1}+1}=\frac{3}{2}\)
\(\Rightarrow a^2+b^2=3^2+2^2=13\)

\(\lim\limits_{x\rightarrow1}\dfrac{\sqrt{3x^2+2}-\sqrt{4+x}}{x^2-1}=\lim\limits_{x\rightarrow1}\dfrac{\dfrac{3x^2-x-2}{\sqrt{3x^2+2}+\sqrt{4+x}}}{x^2-1}=\lim\limits_{x\rightarrow1}\dfrac{3x+2}{\left(x+1\right)\left(\sqrt{3x^2+2}+\sqrt{4+x}\right)}=\dfrac{5}{2.2\sqrt{5}}=\dfrac{\sqrt{5}}{4}\).
Từ đó a = 5; b = 4 nên a - b = 1.

Ta có:
\(sin18^0=cos72^0=2cos^236^0-1\)
\(cos36^0=1-2sin^218^0\)
Đặt \(sin18^0=x\), \(x\in\left(0;1\right)\) thì ta có:
\(x=2\left(1-2x^2\right)^2-1\)
\(\Leftrightarrow x=2\left(4x^4-4x^2+1\right)-1\)
\(\Leftrightarrow8x^4-8x^2-x+1=0\)
\(\Leftrightarrow\left(x-1\right)\left(2x+1\right)\left(x-\dfrac{-1+\sqrt{5}}{4}\right)\left(x-\dfrac{-1-\sqrt{5}}{4}\right)=0\)
Do \(x\in\left(0;1\right)\) nên \(x=\dfrac{-1+\sqrt{5}}{4}\) \(\Rightarrow S=a+b+c=-1+1+4=4\)
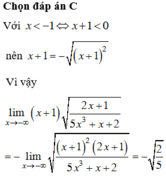
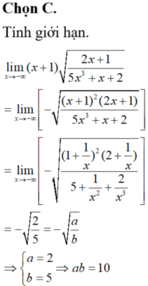
Chọn A
Ta có:
Do đó,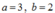 . Vậy
. Vậy