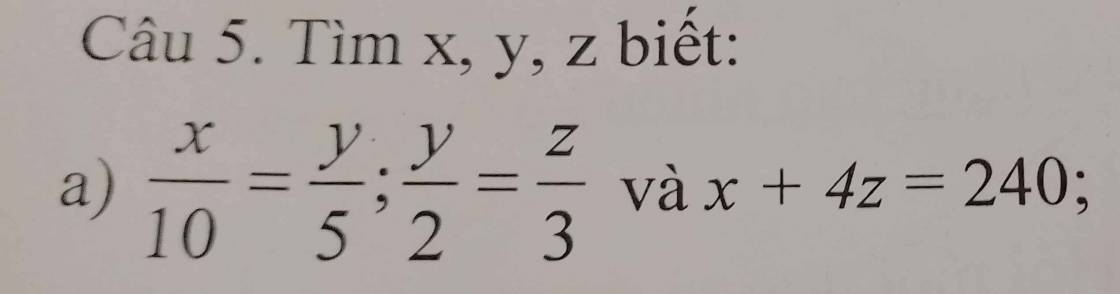giúp em giải câu này ạ e cần gấp ạ
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
TH
2
L
10 tháng 5 2022
2. There used to have many old buildings 10 years ago
3. I wish a new mall didn't build
4. I have had this wardrobe since my wedding day
5. She hasn't been seen for two years
TT
Trần Thị Ngọc Lan
Giáo viên
27 tháng 5 2022
câu 6: bài học: hãy là chính mình, biết sống hết mình, làm tốt những trách nhiệm, bổn phận của bản thân sẽ được mọi người tôn trọng, yêu quý
LT
0
A
0
TC
27 tháng 5 2022
\(x+\sqrt{4-x^2}=2\)
\(\Leftrightarrow4-x^2=\left(2-x\right)^2\)
\(\Leftrightarrow4-x^2=4-8x+x^2\)
\(\Leftrightarrow4-x^2-4+8x-x^2=0\)
\(\Leftrightarrow8x-2x^2=0\)
\(\Leftrightarrow2x\left(4-x\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}2x=0\\4-x=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=0\\x=4\end{matrix}\right.\)
TC
28 tháng 5 2022
\(x+\sqrt{1-x^2}=1\)
\(\Leftrightarrow1-x^2=\left(1-x\right)^2\)
\(\Leftrightarrow1-x^2=1-2x+x^2\)
\(\Leftrightarrow1-x^2-1+2x-x^2=0\)
\(\Leftrightarrow2x-2x^2=0\)
\(\Leftrightarrow2x\left(1-x\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}2x=0\\1-x=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=0\\x=1\end{matrix}\right.\)
Ta có:
\(\dfrac{x}{10}=\dfrac{y}{5}\)
\(\Rightarrow\dfrac{x}{20}=\dfrac{y}{10}\) \(\left(1\right)\)
\(\dfrac{y}{2}=\dfrac{z}{3}\)
\(\Rightarrow\dfrac{y}{10}=\dfrac{z}{15}\) \(\left(2\right)\)
Từ \(\left(1\right)\) và \(\left(2\right)\)
\(\Rightarrow\dfrac{x}{20}=\dfrac{y}{10}=\dfrac{z}{15}\)
Lại có:
\(\dfrac{z}{15}=\dfrac{4z}{60}\)
Áp dụng tính chất của dãy tỉ số bằng nhau,ta có:
\(\dfrac{x}{20}=\dfrac{y}{10}=\dfrac{4z}{60}=\dfrac{x+4z}{20+60}=\dfrac{240}{80}=3\)
\(\Rightarrow x=3\cdot20=60\)
\(y=3\cdot10=30\)
\(z=3\cdot15=45\)