Cho tam thức f (x) = x2 - 2(4m - 1)x +15m2 -2m -7. Tìm tất cả các giá trị tham số của m để f(x) > 0 với mọi x thuộc R.
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


Ta có \(f\left(x\right)>0,\forall x\in\left(0;1\right)\)
\(\Leftrightarrow-x^2-2\left(m-1\right)x+2m-1>0,\forall x\left(0;1\right)\)
\(\Leftrightarrow-2m\left(x-1\right)>x^2-2x+1,\forall x\in\left(0;1\right)\) (*)
Vì \(x\in\left(0;1\right)\Rightarrow x-1< 0\) nên (*) \(\Leftrightarrow-2m< \dfrac{x^2-2x+1}{x-1}=x-1=g\left(x\right),\forall x\in\left(0;1\right)\)
\(\Leftrightarrow-2m\le g\left(0\right)=-1\Leftrightarrow m\ge\dfrac{1}{2}\)

\(f\left(x\right)=-x^2-2x+m\)
\(f\left(x\right)\le0,\forall x\in R\left\{{}\begin{matrix}a< 0\\\Delta\le0\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}-1< 0\left(LĐ\right)\\\left(-2\right)^2-4.\left(-1\right).m\le0\end{matrix}\right.\)
\(\Leftrightarrow4+4m\le0\)
\(\Leftrightarrow4m\le-4\)
\(\Leftrightarrow m\le-1\)

\(\Leftrightarrow\left(x-y+m\right)^2+y^2+2\left(m+1\right)y-m^2+25\ge0\); \(\forall x;y\)
\(\Leftrightarrow y^2+2\left(m+1\right)y-m^2+25\ge0\) ;\(\forall y\)
\(\Leftrightarrow\Delta'=\left(m+1\right)^2-\left(-m^2+25\right)\le0\)
\(\Leftrightarrow m^2+m-12\le0\Rightarrow-4\le m\le3\)
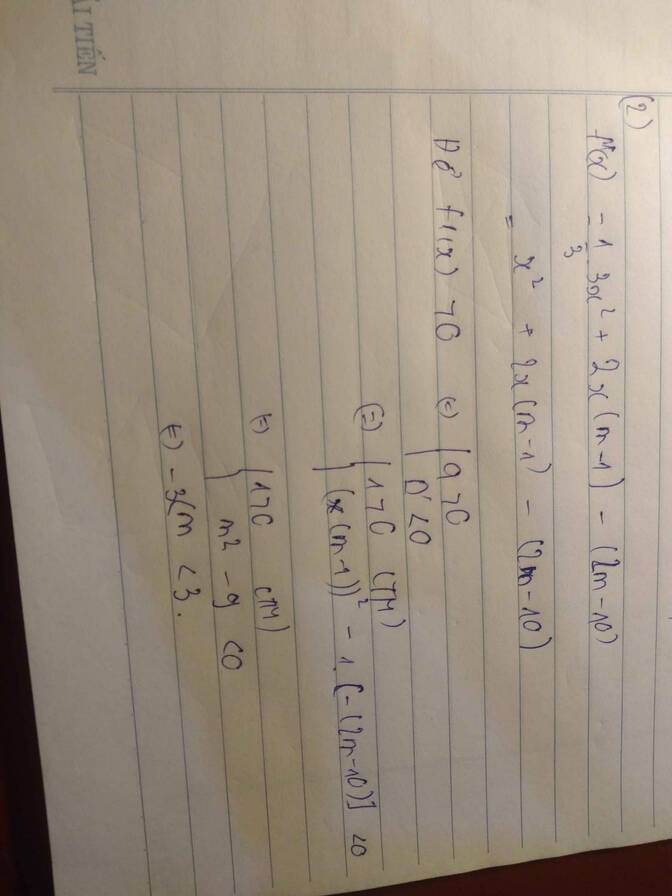
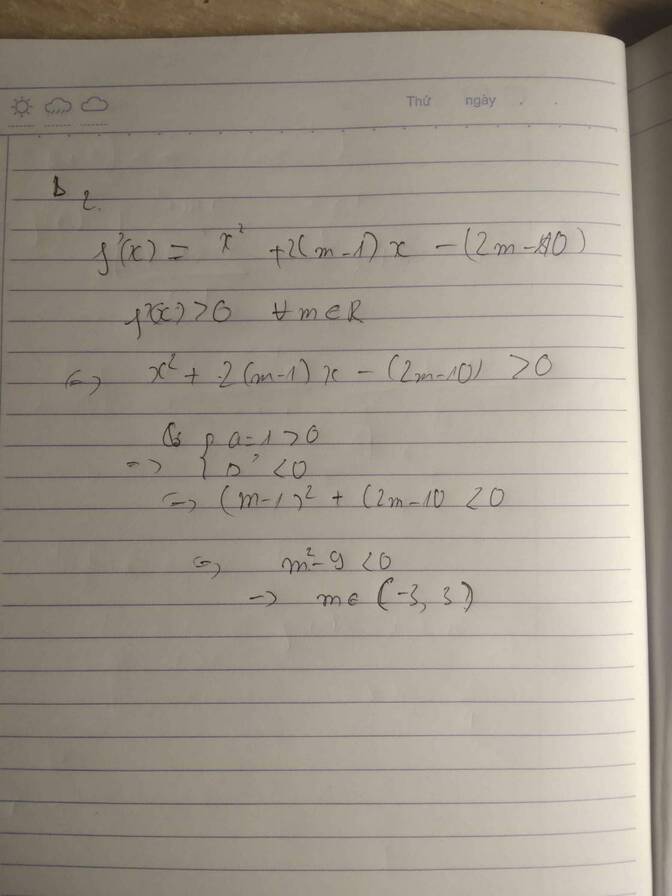


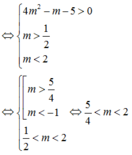
Do \(a=1>0\) nên để \(f\left(x\right)>0\) \(\forall x\)
\(\Leftrightarrow\Delta'< 0\)
\(\Leftrightarrow\left(4m-1\right)^2-\left(15m^2-2m-7\right)< 0\)
\(\Leftrightarrow m^2-6m+8< 0\)
\(\Leftrightarrow2< m< 4\)