K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
TA
17 tháng 6 2021
Đặt `y=f(x)=x-sinx`
Có: `f(-x)=-x-sin(-x)=-x+sinx=-(x-sinx)=-f(x)`
`=>` Hàm lẻ.
TA
16 tháng 6 2021
1) Đặt `y=f(x) =-2cosx`
Có: `f(-x) = -2cos(-x) = -2cosx=f(x)`
`=>` Hàm chẵn.
4) `y=f(x) = 1/2 tan^2 x`
`f(-x) = 1/2 [tan (-x)]^2 = 1/2 tan^2x=f(x)`
`=>` Hàm chẵn.
7) `y=f(x)=sin2x`
`f(-x)=sin(-2x)=-sin2x=-f(x)`
`=>` Hàm lẻ.
10) `y=f(x)=tanx.sinx`
`f(-x) = tan (-x) .sin(-x) =tanx.sinx=f(x)`
`=>` Hàm chẵn.
16 tháng 6 2021
2) TXĐ: \(D=R\Rightarrow\forall x\in R\) thì \(-x\in R\)
\(y=f\left(x\right)=sinx+x\)
\(\Rightarrow f\left(-x\right)=sin\left(-x\right)+\left(-x\right)=-sinx-x=-\left(sinx+x\right)=-f\left(x\right)\)
\(\Rightarrow\)\(y=sinx+x\) là hàm lẻ
5) TXĐ: \(D=R\Rightarrow\forall x\in R\) thì \(-x\in R\)
\(y=f\left(x\right)=sin\left|x\right|+x^2\)
\(\Rightarrow f\left(-x\right)=sin\left|-x\right|+\left(-x\right)^2=sin\left|x\right|+x^2=f\left(x\right)\)
\(\Rightarrow y=sin\left|x\right|+x^2\) là hàm chẵn
8) TXĐ: \(D=R\Rightarrow\forall x\in R\) thì \(-x\in R\)
\(y=f\left(x\right)=-2+3cosx\)
\(\Rightarrow f\left(-x\right)=-2+3cos\left(-x\right)=-2+3cosx=f\left(x\right)\)
\(\Rightarrow y=-2+3cosx\) là hàm chẵn
11) TXĐ:\(D=R\Rightarrow\forall x\in R\) thì \(-x\in R\)
\(y=f\left(x\right)=cosx-sin\left|x\right|\)
\(\Rightarrow f\left(-x\right)=cos\left(-x\right)-sin\left|-x\right|=cosx-sin\left|x\right|=\)\(f\left(x\right)\)
\(\Rightarrow y=cosx-sin\left|x\right|\) là hàm chẵn
N
3 tháng 9 2016
TXĐ:R
\(\forall x\in R\Rightarrow\begin{cases}-x\in R\\f\left(-x\right)=3sin\left(-x\right)-2=-3sinx-2\end{cases}\)
Gỉa sử:x=\(\frac{\pi}{2}\Rightarrow\)\(f\left(\frac{\pi}{2}\right)=1\)\(\ne f\left(-\frac{\pi}{2}\right)=-5\)\(\ne-f\left(\frac{\pi}{2}\right)\)=-1
Vậy hàm số không có tính chẵn-lẻ
NV
Nguyễn Việt Lâm
Giáo viên
17 tháng 12 2020
Miền xác định của hàm là miền đối xứng
\(y\left(-x\right)=cot\left(-x\right)-sin\left(-x-1\right)=-cotx+sin\left(x+1\right)\)
\(y\left(-x\right)\ne y\left(x\right)\) mà cũng khác \(-y\left(x\right)\) nên hàm không chẵn không lẻ
NT
0
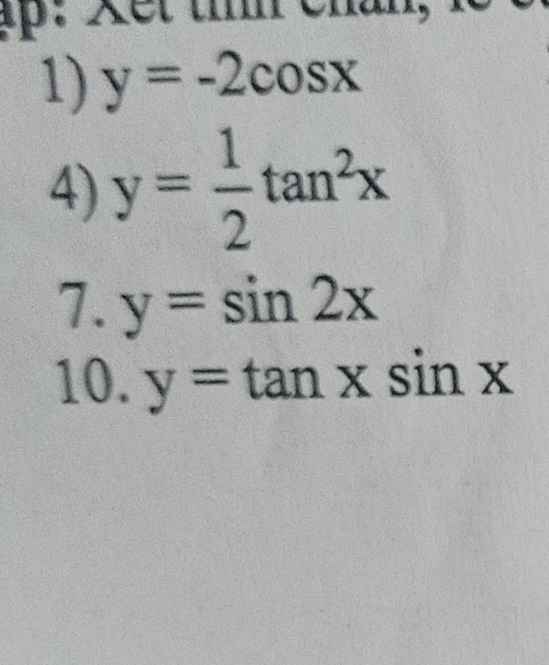
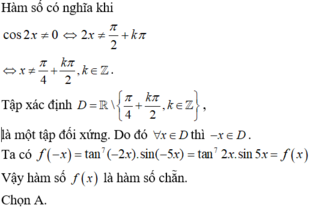
`y=f(x)=1/(x.cos3x)`
`f(-x)=1/(-x.cos(-3x))=-1/(x.cos3x)=-f(x)`
`=>` Lẻ.
.
`y=f(x)=(cos^3x+1)/(sin^3x)`
`f(-x)=((cos(-x))^3+1)/((sin(-x))^3)=(-cos^3x-1)/(sin^3x)=-f(x)`
`=>` Lẻ.