K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
NV
Nguyễn Việt Lâm
Giáo viên
22 tháng 5 2021
\(\Delta'=9-\left(2n-3\right)=12-2n>0\Rightarrow n< 6\)
Khi đó theo hệ thức Viet: \(\left\{{}\begin{matrix}x_1+x_2=6\\x_1x_2=2n-3\end{matrix}\right.\)
Do \(x_1\) là nghiệm của pt nên:
\(x_1^2-6x_1+2n-3=0\Leftrightarrow x_1^2-5x_1+2n-4=x_1-1\)
Tương tự ta có: \(x_2^2-5x_2+2n-4=x_2-1\)
Thế vào bài toán:
\(\left(x_1-1\right)\left(x_2-1\right)=-4\Leftrightarrow x_1x_2-\left(x_1+x_2\right)+1=-4\)
\(\Leftrightarrow2n-3-6+1=-4\Rightarrow n=2\)
28 tháng 5 2021
\(x^2-6x+2m-3=0\)
\(\Delta=b^2-4ac=36-4\left(2m-3\right)=36-8m+12=48-8m\)
Để phương trình có hai nghiệm phân biệt thì \(\Delta>0\)\(< =>48-8m>0< =>48>8m< =>6>m\)
Theo Vi-ét ta có :\(\hept{\begin{cases}x_1x_2=\frac{c}{a}=2m-3\\x_1+x_2=\frac{-b}{a}=6\end{cases}}\)là
\(x_1\)là nghiệm phương trình \(x_1^2-6x_1+2m-3=0\)
\(=>x_1^2=3-2m+6x_1\)
\(x_2\)là nghiệm phương trình \(x_2^2-6x_2+2m-3=0\)
\(=>x_2^2=3-2m+6x_2\)
Mà \(\left(x_1^2-5x_1+2m-4\right)\left(x_2^2-5x_2+2m-4\right)=2\)
\(\left(3-2m+6x_1-5x_1+2m-4\right)\left(3-2m+6x_2-5x_2+2m-4\right)=2\)
\(\left(3+x_1-4\right)\left(3+x_2-4\right)=2\)
\(\left(x_1-1\right)\left(x_2-1\right)=2\)
\(x_1x_2-x_1-x_2+1=2\)
\(x_1x_2-\left(x_1+x_2\right)=1\)
\(2m-3-6=1\)
\(2m-9=1\)
\(m=5\)
Vậy m=5
20 tháng 2 2022
Thay n = 4 vào pt (1) ta có
\(x^2-6x+5=0\\ ta.có.a+b+c=1-6+5=0\\ Vậy.pt.có.n_o:\\ x_1=1;x_2=\dfrac{c}{a}=5\)
\(Ta.có:\Delta=b^2-4ac=....=-8n+48\\ Để.pt.\left(1\right).có.1.n_o.phân.biệt.thì.\Delta>0\\ \Leftrightarrow n< 6\)
Vậy m < 6 thì pt (1) có nghiệm phân biệt \(x_1;x_2\) nên theo Vi ét ta có
\(x_1+x_2=\dfrac{-b}{a}=6\\ x_1x_2=\dfrac{c}{a}=2n-3\)
Ta có
\(x^2-6x+2n-3=0\\ \Leftrightarrow x^2-5x+2n-4=x-1\)
Vì x1 x2 là nghiệm pt \(x^2-6x+2n-3=0\) nên x1 x2 là nghiệm PT \(x^2-5x+2n-4=x-1\) nên ta có
\(x_1^2-5x+2x-4=x_1-1.và\\ x_2^2-5x_2+2n-4=x_2-1\\ \Rightarrow\left(x_1^2-5x_1+2n-4\right)\left(x_2^2-5x_2+2n-4\right)=\left(x_1-1\right)\left(x_2-1\right)\)
\(Mà\\ \left(x_1^2-5x_1+2n-4\right)\left(x_2^2-5x_2+2n-4\right)=-4\\ Nên\left(x_1-1\right)\left(x_2-1\right)=-4\\ \Leftrightarrow x_1x_2-\left(x_1+x_2\right)+1=-4\\ \Leftrightarrow2n-3-6+1=-4\\ \Leftrightarrow2n=4\Rightarrow n=2\left(tm\right)\\ ......\left(kl\right)\)
NV
Nguyễn Việt Lâm
Giáo viên
3 tháng 5 2021
\(\Delta'=9-\left(2n-3\right)>0\Leftrightarrow n< 6\)
Theo hệ thức Viet: \(\left\{{}\begin{matrix}x_1+x_2=6\\x_1x_2=2n-3\end{matrix}\right.\)
Do \(x_1;x_2\) là nghiệm nên:
\(\left\{{}\begin{matrix}x_1^2-6x_1+2n-3=0\\x_2^2-6x_2+2n-3=0\end{matrix}\right.\) \(\Leftrightarrow\left\{{}\begin{matrix}x_1^2-5x_1+2n-4=x_1-1\\x_2^2-5x_2+2n-4=x_2-1\end{matrix}\right.\)
Thay vào bài toán:
\(\left(x_1-1\right)\left(x_2-1\right)=-4\)
\(\Leftrightarrow x_1x_2-\left(x_1+x_2\right)+5=0\)
\(\Leftrightarrow2n-3-6+5=0\Leftrightarrow n=2\)
4 tháng 7 2021
\(\Delta=m^2-4\left(m-4\right)=\left(m^2-4m+4\right)+12=\left(m-2\right)^2+12>0;\forall m\)
Suy ra pt luôn có hai nghiệm pb với mọi m
Theo viet có:\(\left\{{}\begin{matrix}x_1+x_2=m\\x_1.x_2=m-4\end{matrix}\right.\)
\(\left(5x_1-1\right)\left(5x_2-1\right)< 0\)
\(\Leftrightarrow25x_1x_2-5\left(x_1+x_2\right)+1< 0\)
\(\Leftrightarrow25\left(m-4\right)-5m+1< 0\)
\(\Leftrightarrow m< \dfrac{99}{20}\)
Vậy...
AT
4 tháng 7 2021
\(\Delta=m^2-4m+16=\left(m-2\right)^2+12>0\)
\(\Rightarrow\) pt luôn có 2 nghiệm phân biệt
Áp dụng hệ thức Vi-ét: \(\left\{{}\begin{matrix}x_1+x_2=m\\x_1x_2=m-4\end{matrix}\right.\)
Ta có: \(\left(5x_1-1\right)\left(5x_2-1\right)=25x_1x_2-5\left(x_1+x_2\right)+1\)
\(=25\left(m-4\right)-5m+1=20m-99\)
\(\Rightarrow20m-99< 0\Rightarrow m< \dfrac{99}{20}\)
NT
2
DA
11 tháng 4 2023
xét ptr \(2x^2-x-3+0\)
△=\(\left(-1\right)^2-4.2.\left(-3\right)=25>0\)
⇒ptr có 2 ngh phân biệt \(x_1;x_2\)
Theo hệt thức viet \(x_1+x_2=\dfrac{1}{2};x_1x_2=\dfrac{-3}{2}\)
Xét A = \(x_1^2+x_2^2-x_1^2x_2^2=\left(x_1+x_2\right)^2-2x_1x_2-x_1^2x_2^2\)
=\(\left(\dfrac{1}{2}\right)^2-2\left(-\dfrac{3}{2}\right)-\left(-\dfrac{3}{2}\right)^2=\dfrac{1}{4}+3-\dfrac{9}{4}=3-2=1\)
Của cậu đây ạ, kh hiểu j thì hỏi tớ nha <3
CM
20 tháng 12 2017
a) 2 x 2 – 17 x + 1 = 0
Có a = 2; b = -17; c = 1
Δ = b 2 – 4 a c = ( - 17 ) 2 – 4 . 2 . 1 = 281 > 0 .
Theo hệ thức Vi-et: phương trình có hai nghiệm x1; x2 thỏa mãn:
x 1 + x 2 = − b / a = 17 / 2 x 1 x 2 = c / a = 1 / 2
b) 5 x 2 – x – 35 = 0
Có a = 5 ; b = -1 ; c = -35 ;
Δ = b 2 – 4 a c = ( - 1 ) 2 – 4 . 5 . ( - 35 ) = 701 > 0
Theo hệ thức Vi-et, phương trình có hai nghiệm x1; x2 thỏa mãn:
x 1 + x 2 = − b / a = 1 / 5 x 1 ⋅ x 2 = c / a = − 35 / 5 = − 7
c) 8 x 2 – x + 1 = 0
Có a = 8 ; b = -1 ; c = 1
Δ = b 2 – 4 a c = ( - 1 ) 2 – 4 . 8 . 1 = - 31 < 0
Phương trình vô nghiệm nên không tồn tại x1 ; x2.
d) 25 x 2 + 10 x + 1 = 0
Có a = 25 ; b = 10 ; c = 1
Δ = b 2 – 4 a c = 10 2 – 4 . 25 . 1 = 0
Khi đó theo hệ thức Vi-et có:
x 1 + x 2 = − b / a = − 10 / 25 = − 2 / 5 x 1 x 2 = c / a = 1 / 25
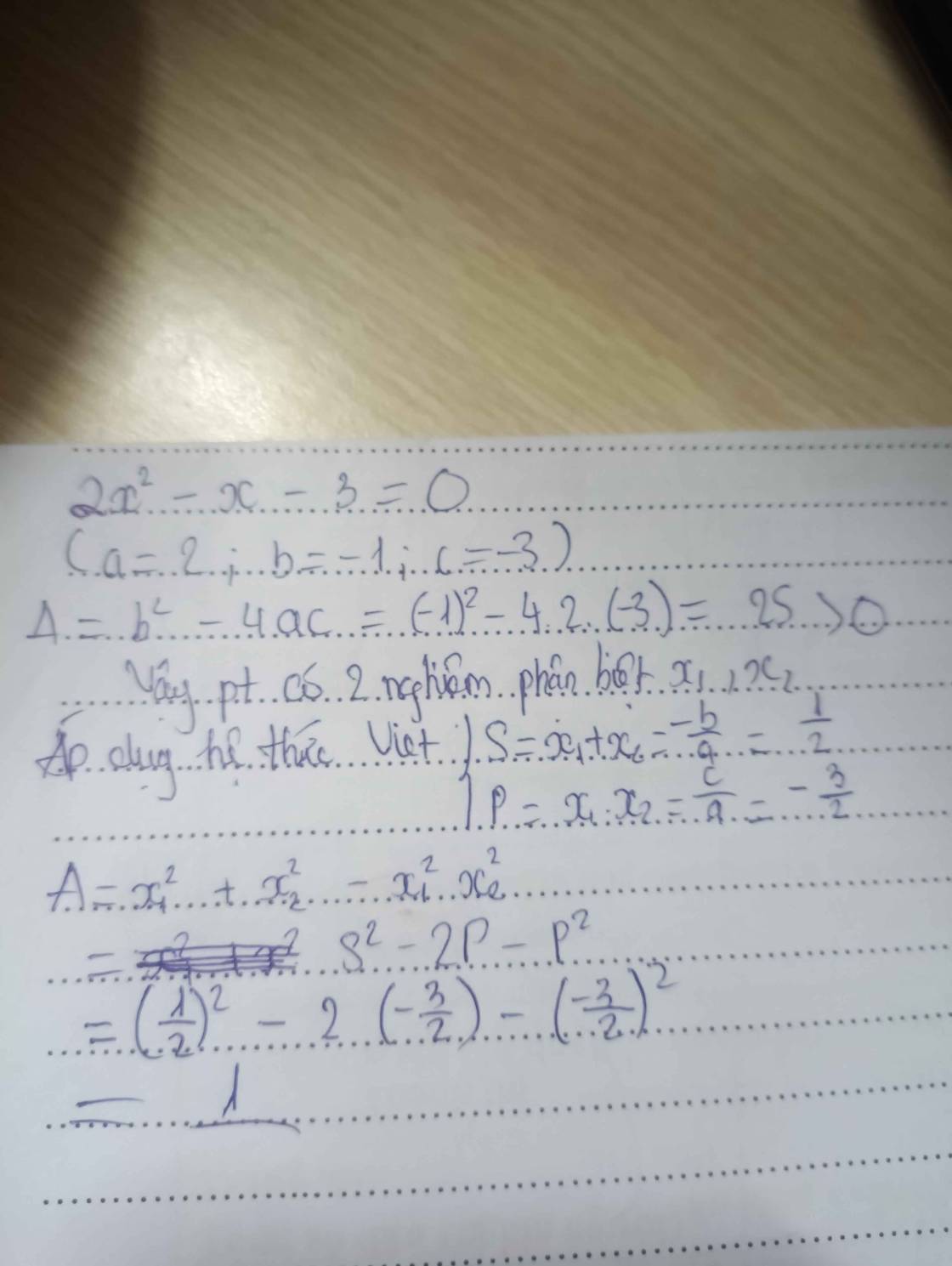
\(A=\dfrac{5x_1-x_2}{x_1}+\dfrac{5x_2-x_1}{x_2}\)
\(=\dfrac{5x_1\cdot x_2-x_2^2+5x_1x_2-x_1^2}{x_1x_2}\)
\(=\dfrac{10x_1x_2-\left[\left(x_1+x_2\right)^2-2x_1x_2\right]}{x_1x_2}\)
\(=\dfrac{10\cdot4-\left[5^2-2\cdot4\right]}{4}=\dfrac{40-25+8}{4}=\dfrac{23}{4}\)