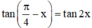Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Bài 6. Các giá trị cần tìm của x là các nghiệm của phương trình
tan 2x = tan (![]() - x) ,
- x) ,
Đáp số : ![]() ( k ∈ Z, k - 2 không chia hết cho 3).
( k ∈ Z, k - 2 không chia hết cho 3).
Giá trị của x cần tìm là nghiệm của phương trình:
\(tan\left(\dfrac{\pi}{4}-x\right)=tan2x\)
pt\(\Leftrightarrow\left\{{}\begin{matrix}cos\left(\dfrac{\pi}{4}-x\right)\ne0\\cos2x\ne0\\\dfrac{\pi}{4}-x=2x+k\pi\end{matrix}\right.\)\(\Leftrightarrow\left\{{}\begin{matrix}cos2x\ne0\\3x=\dfrac{\pi}{4}+k\pi\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}2x\ne\dfrac{\pi}{2}+k\pi\\x=\dfrac{\pi}{12}+\dfrac{k\pi}{3}\end{matrix}\right.\)\(\Leftrightarrow\left\{{}\begin{matrix}x\ne\dfrac{\pi}{4}+\dfrac{k\pi}{2}\\x=\dfrac{\pi}{12}+\dfrac{k\pi}{3}\end{matrix}\right.\)\(\Leftrightarrow x=\dfrac{\pi}{12}+\dfrac{k\pi}{3}\).

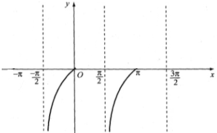
Quan sát đồ thị hàm số y = tan x trên đoạn [-π; 3π/2].
a. tan x = 0 tại các giá trị x = -π; 0; π.
(Các điểm trục hoành cắt đồ thị hàm số y = tanx).
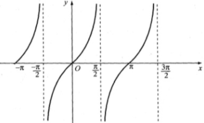
b. tan x = 1 tại các giá trị x = -3π/4; π/4; 5π/4.
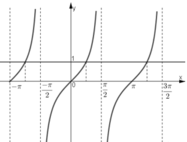
c. tan x > 0 với x ∈ (-π; -π/2) ∪ (0; π/2) ∪ (π; 3π/2).
(Quan sát hình dưới)
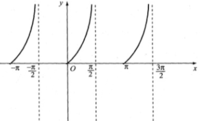
d. tan x < 0 khi x ∈ [-π/2; 0) ∪ [π/2; π)
(Quan sát hình dưới).

1. Không dịch được đề
2.
\(-1\le cos2x\le1\Rightarrow1\le y\le3\)
3.
a. \(-2\le2sinx\le2\Rightarrow-1\le y\le3\)
\(y_{min}=-1\) khi \(sinx=-1\Rightarrow x=-\dfrac{\pi}{2}+k2\pi\)
\(y_{max}=3\) khi \(sinx=1\Rightarrow x=\dfrac{\pi}{2}+k2\pi\)
b.
\(0\le cos^2x\le1\Rightarrow-1\le y\le2\)
\(y_{min}=-1\) khi \(cos^2x=1\Rightarrow x=k\pi\)
\(y_{max}=2\) khi \(cosx=0\Rightarrow x=\dfrac{\pi}{2}+k\pi\)
4.
\(y=\left(tanx-1\right)^2+2\ge2\)
\(y_{min}=2\) khi \(tanx=1\Rightarrow x=\dfrac{\pi}{4}+k\pi\)

y = \(\dfrac{sin^2x}{cosx\left(sinx-cosx\right)}+\dfrac{1}{4}\)
y = \(\dfrac{sin^2x}{sinx.cosx-cos^2x}+\dfrac{1}{4}=\dfrac{\dfrac{sin^2x}{cos^2x}}{\dfrac{sinx.cosx}{cos^2x}-1}+\dfrac{1}{4}\)
y = \(\dfrac{tan^2x}{tanx-1}+\dfrac{1}{4}\)
y = \(\dfrac{4tan^2x+tanx-1}{4tanx-4}\). Đặt t = tanx. Do x ∈ \(\left(\dfrac{\pi}{4};\dfrac{\pi}{2}\right)\) nên t ∈ (1 ; +\(\infty\))\
Ta đươc hàm số f(t) = \(\dfrac{4t^2+t-1}{4t-4}\)
⇒ ymin = \(\dfrac{17}{4}\) khi t = 2. hay x = arctan(2) + kπ
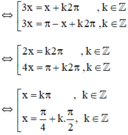
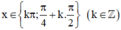 thì sin x = sin 3x.
thì sin x = sin 3x.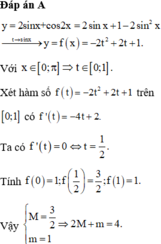
Kết hợp với điều kiện xác định suy ra
Vậy với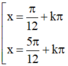 (k ∈ Z)
(k ∈ Z)
thì