VV
2
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
28 tháng 8 2015
a, | x + 1,3 | = 3,3
TH1 : x + 1,3 = 3,3
x = 3,3 - 1,3
x = 2
TH2 : x + 1,3 = -3,3
x = -3,3 - 1,3
x = -4,6
PD
4
26 tháng 6 2015
Mình làm rồi nhớ chọn Đúng đấy :
a) |2,5 - x| = 1,3
=> hoặc 2,5 - x = 1,3 hoặc 2,5 - x = -1,3
=> hoặc x = 1,2 hoặc x = 3,8
26 tháng 6 2015
a,
Ta co :
|2,5-x|=1,3
Ta thấy đây trên có 2 trườg hợp
suy ra :|2,5-x|=-+1,3
TH1:
2,5-x=-1,3
x = 2,5 - (-1,3)
x = 3,8
TH2:
2,5-x=1,3
x = 2,5-1,3
x = 1,2
Vậy :s=1,2 và 3,8
b,
Ta co :
16-|x-0,2|=0
|x-0,2| =16-0
|x-0,2| = 16
Ta thay day tren co 2 t/h
suy ra : |x-0,2|=-+16
TH1:
x-0,2=-16
x = -16 + 0,2
x = -15,8
TH2:
x-0,2=16
x = 16 + 0,2
x = 16,2
Vậy s=16,2 và -15,8
DN
6
10 tháng 8 2017
Ta có : |2,5 - x| = 1,3
\(\Leftrightarrow\orbr{\begin{cases}2,5-x=1,3\\2,5-x=-1,3\end{cases}}\)
\(\Leftrightarrow\orbr{\begin{cases}x=2,5-1,3\\x=2,5+1,3\end{cases}}\)
\(\Leftrightarrow\orbr{\begin{cases}x=1,2\\x=3,8\end{cases}}\)
b) Ta có : 1,6 - |x - 0,2| = 0
=> |x - 0,2| = 1,6
\(\Leftrightarrow\orbr{\begin{cases}x-0,2=1,6\\x-0,2=-1,6\end{cases}}\)
\(\Leftrightarrow\orbr{\begin{cases}x=1,6+0,2\\x=-1,6+0,2\end{cases}}\)
\(\Leftrightarrow\orbr{\begin{cases}x=1,8\\x=-1,4\end{cases}}\)
c) Ta có : \(\left|x-1,5\right|+\left|2,5-x\right|\ge\left|x-1,5+2,5-x\right|\forall x\)
\(\left|x-1,5\right|+\left|2,5-x\right|\ge\left|x-1,5+2,5-x\right|=1\forall x\)
Vậy sai đề : D
TN
10 tháng 8 2017
a, Th1: X = 2,5 - 1,3 = 1,2
TH2: X = -2,5 - 1,3 = -3,8
b, và c, bạn cx làm 2 trường hợp tương tự như vậy
16 tháng 8 2021
c: Ta có: \(\left|\dfrac{1}{2}x-2\right|-\left|x+3\right|=0\)
\(\Leftrightarrow\left|\dfrac{1}{2}x-2\right|=\left|x+3\right|\)
\(\Leftrightarrow\left[{}\begin{matrix}\dfrac{1}{2}x-2=x+3\\\dfrac{1}{2}x-2=-x-3\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x\cdot\dfrac{-1}{2}=5\\x\cdot\dfrac{3}{2}=-1\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=-10\\x=-\dfrac{2}{3}\end{matrix}\right.\)
RD
3
14 tháng 7 2015
a./2.5-x/=1,3 hay /5/2-x/=13/10. => 5/2-x= 13/10 hoặc -13/10
* 5/2-x=13/10 => x=6/5 * 5/2-x= -13/10 => x=19/5
b giải tương tự
c./x-1,5/+/2,5-x/=0
vì /x-1,5/> hoặc =0, /2,5-x/> hoặc =0
=> x-1,5=0 và 2,5-x=0
* x-1,5=0 => x=1,5 2,5-x=0 => x= 2,5
1 tháng 11 2017
minh cũng làm tương tự như bạn Tiên nha
k tui nha
thanks
16 tháng 6 2017
c) Ta có: \(\left\{{}\begin{matrix}\left|x-1,5\right|\ge0\forall x\in Q\\\left|2,5-x\right|\ge0\forall x\in Q\end{matrix}\right.\)
\(\Rightarrow\left|x-1,5\right|+\left|2,5-x\right|\ge0\forall x\in Q\)
Dấu "=" xảy ra khi \(\left\{{}\begin{matrix}\left|x-1,5\right|=0\\\left|2,5-x\right|=0\end{matrix}\right.\)
\(\Rightarrow\left\{{}\begin{matrix}x=1,5\\x=2,5\end{matrix}\right.\)
Vậy \(x=\left\{{}\begin{matrix}1,5\\2,5\end{matrix}\right.\).
e) \(\left(x-2\right)^2=1\)
\(\Rightarrow\left[{}\begin{matrix}x-2=\sqrt{1}\\x-2=-\sqrt{1}\end{matrix}\right.\) \(\Rightarrow\left[{}\begin{matrix}x=3\\x=1\end{matrix}\right.\)
Vậy \(\left[{}\begin{matrix}x=3\\x=1\end{matrix}\right.\).
Mấy câu kia dễ rồi.
AT
16 tháng 6 2017
sửa lại ý c của N.Anh
Áp dụng bđt \(\left|a\right|+\left|b\right|\ge\left|a+b\right|\) có:
\(\left|x-1,5\right|+\left|2,5-x\right|\ge\left|x-1,5+2,5-x\right|=1\)
\(\Rightarrow\left|x-1,5\right|+\left|2,5-x\right|\ge1>0\)
mà theo đề thì \(\left|x-1,5\right|+\left|2,5-x\right|=0\)
\(\Rightarrow\) k có gt \(x\) nào tm yêu cầu đề bài
LK
3
7 tháng 10 2016
a) | 2,5 - x | = 1,3
=> 2,5 - x = 1,3 hoặc 2,5 - x = -1,3
Hay: x = 1,3 + 2,5 hoặc x = (-1,3) + 2,5
=> x = 3,8 hoặc x = 1,2
b) 1,6 - | x - 0,2 | = 0
| x - 0,2 | = 1,6 - 0 = 1,6
=> x - 0,2 = 1,6 hoặc x - 0,2 = -1,6
Hay: x = 1,6 + 0,2 hoặc x = (-1,6) + 0,2
=> x = 1,8 hoặc x = -1,4
c) | x - 1,5 | + | 2,5 - x | = 0
Vì giá trị tuyệt đối luôn > hoặc = 0
=> | x - 1,5 | = 0 và | 2,5 - x | = 0
=> x - 1,5 = 0 và 2,5 - x = 0
=> x = 1,5 và x = 2,5
Mà 1,5 khác 2,5
=> Không thỏa mãn x sao cho | x - 1,5 | + | 2,5 - x | = 0
2 tháng 12 2017
x + x : 0,2 = 1,35
x * 1 + x * 5 = 1,35
x * ( 1 + 5 ) = 1,35
x * 6 = 1,35
x = 1,35 : 6
x = 0,225
hok tốt nha ^_^
NB
1
HH
15 tháng 9 2016
a.\(\Rightarrow\begin{cases}2,5-x=1,3\\2,5-x=-1,3\end{cases}\)\(\Rightarrow\begin{cases}x=1,2\\x=3,8\end{cases}\)
b.\(\Rightarrow\begin{cases}x-1,5=0\\2,5-x=0\end{cases}\)\(\Rightarrow\begin{cases}x=1,5\\x=2,5\end{cases}\)
c.\(\left|x-0,2\right|=1,6\)
\(\Rightarrow\begin{cases}x-0,2=1,6\\x-0,2=-1,6\end{cases}\)\(\Rightarrow\begin{cases}x=1,8\\x=-1,4\end{cases}\)
tick cho mk nhé
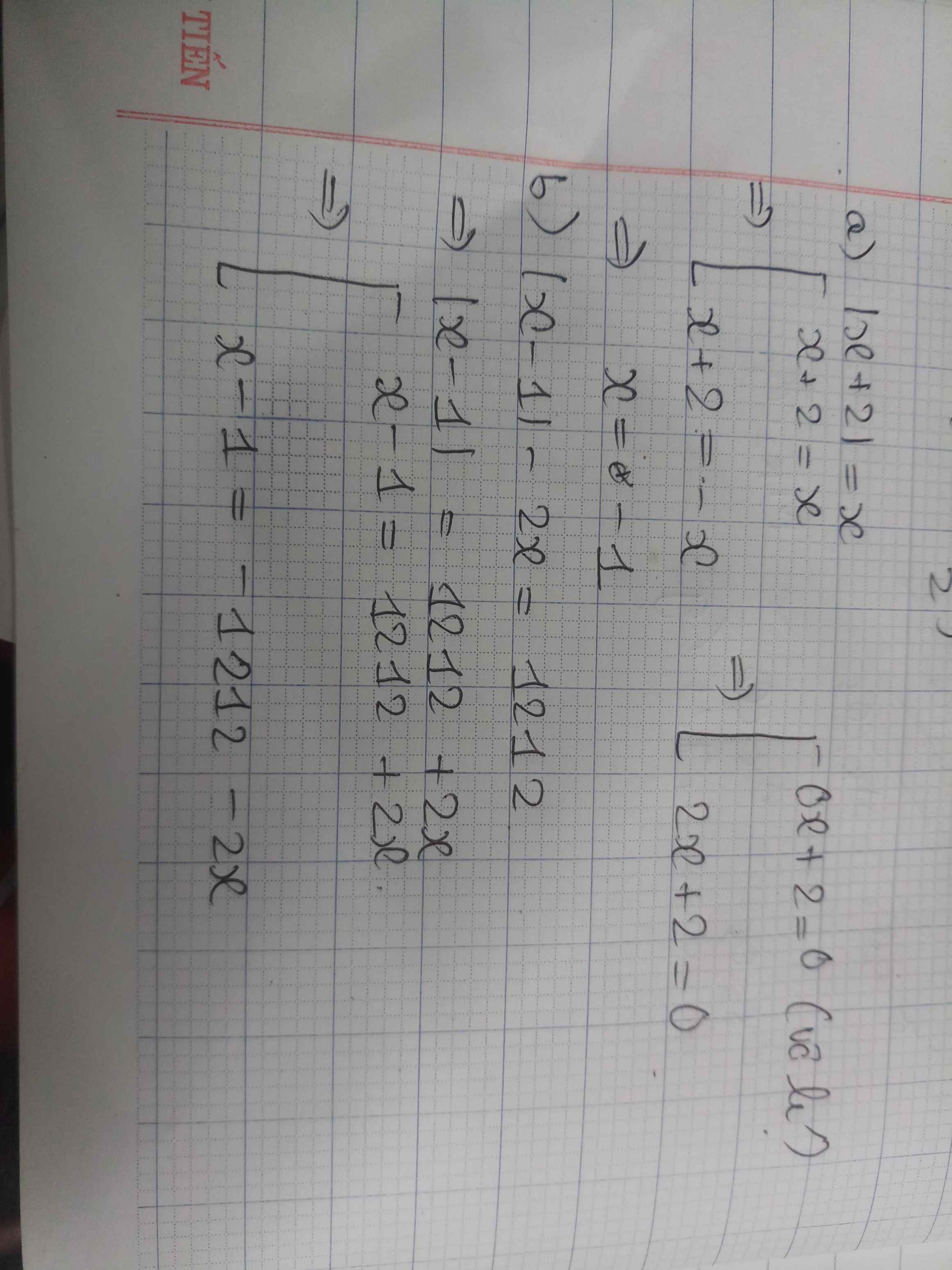
Giải:
a) \(\left|x\right|=1,3\)
\(\Leftrightarrow\left[{}\begin{matrix}x=1,3\\x=-1,3\end{matrix}\right.\)
Vậy ...
b) \(\left|x+1,3\right|=3,3\)
\(\Leftrightarrow\left[{}\begin{matrix}x+1,3=3,3\\x+1,3=-3,3\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=2\\x=-4,6\end{matrix}\right.\)
Vậy ...
c) \(\left|5,6-x\right|=4,6\)
\(\Leftrightarrow\left[{}\begin{matrix}5,6-x=4,6\\5,6-x=-4,6\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=1\\x=10,2\end{matrix}\right.\)
Vậy ...
d) \(\left|3x-5\right|+\left|x-2\right|=0\)
\(\Leftrightarrow\left\{{}\begin{matrix}3x-5=0\\x-2=0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=\dfrac{5}{3}\\x=2\end{matrix}\right.\)
=> Phương trình vô nghiệm
Vậy ...
* Trả lời:
\(a.\left|x\right|=1,3\)
\(\Rightarrow x=1,3\) hoặc \(x=-1,3\)
Vậy \(x=\pm1,3\)
\(b.\left|x+1,3\right|=3,3\)
\(\Rightarrow x+1,3=3,3\) hoặc \(x+1,3=-3,3\)
\(\Rightarrow x=3,3-1,3\) | \(x=-3,3-1,3\)
\(\Rightarrow x=2\) | \(x=-4,6\)
Vậy \(x=2\) ; \(x=-4,6\)
\(c.\left|5,6-x\right|=4,6\)
\(\Rightarrow5,6-x=4,6\) hoặc \(5,6-x=-4,6\)
\(\Rightarrow-x=4,6-5,6\) | \(-x=-4,6-5,6\)
\(\Rightarrow-x=-1\) | \(-x=-10,2\)
\(\Rightarrow x=1\) | \(x=10,2\)
Vậy \(x=1\) ; \(x=10,2\)
\(d.\left|3x-5\right|+\left|x-2\right|=0\)
Lý luận: giá trị tuyệt đối luôn lớn hơn hoặc bằng 0
Nên \(\left|3x-5\right|+\left|x-2\right|\ne0\)
\(\Rightarrow\) Không có giá trị \(x\)