Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

c/
\(\Leftrightarrow cos3x-\sqrt{3}sin3x=\sqrt{3}cos2x-sin2x\)
\(\Leftrightarrow\frac{1}{2}cos3x-\frac{\sqrt{3}}{2}sin3x=\frac{\sqrt{3}}{2}cos2x-\frac{1}{2}sin2x\)
\(\Leftrightarrow cos\left(3x+\frac{\pi}{3}\right)=cos\left(2x+\frac{\pi}{6}\right)\)
\(\Leftrightarrow\left[{}\begin{matrix}3x+\frac{\pi}{3}=2x+\frac{\pi}{6}+k2\pi\\3x+\frac{\pi}{3}=-2x-\frac{\pi}{6}+k2\pi\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=-\frac{\pi}{6}+k2\pi\\x=-\frac{\pi}{10}+\frac{k2\pi}{5}\end{matrix}\right.\)
b/
\(\Leftrightarrow cosx-\sqrt{3}sinx=sin2x-\sqrt{3}cos2x\)
\(\Leftrightarrow\frac{1}{2}cosx-\frac{\sqrt{3}}{2}sinx=\frac{1}{2}sin2x-\frac{\sqrt{3}}{2}cos2x\)
\(\Leftrightarrow cos\left(x+\frac{\pi}{3}\right)=sin\left(2x-\frac{\pi}{3}\right)\)
\(\Leftrightarrow sin\left(2x-\frac{\pi}{3}\right)=sin\left(\frac{\pi}{6}-x\right)\)
\(\Leftrightarrow\left[{}\begin{matrix}2x-\frac{\pi}{3}=\frac{\pi}{6}-x+k2\pi\\2x-\frac{\pi}{3}=\frac{5\pi}{6}+x+k2\pi\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\frac{\pi}{6}+\frac{k2\pi}{3}\\x=\frac{7\pi}{6}+k2\pi\end{matrix}\right.\)

Hướng dẫn giải:
Chọn A.
Ta có: sin2x – 2( m- 1)sinx. cosx – (m- 1).cos2x = m
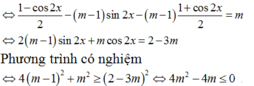
![]()
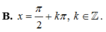
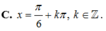


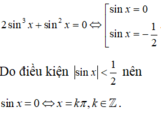

\(\left|cosx\right|-\left|sinx\right|-\left(\left|cosx\right|-\left|sinx\right|\right)\left(\left|cosx\right|+\left|sinx\right|\right)\sqrt{1+sin2x}=0\)
\(\Leftrightarrow\left(\left|cosx\right|-\left|sinx\right|\right)\left(1-\left(\left|cosx\right|+\left|sinx\right|\right)\sqrt{1+sin2x}\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}\left|cosx\right|=\left|sinx\right|\Leftrightarrow cos2x=0\left(1\right)\\\left(\left|cosx\right|+\left|sinx\right|\right)\sqrt{1+sin2x}=1\left(2\right)\end{matrix}\right.\)
\(\left(1\right)\Leftrightarrow x=\dfrac{\pi}{4}+\dfrac{k\pi}{2}\)
\(\left(2\right)\Leftrightarrow\left|cosx\right|+\left|sinx\right|=\dfrac{1}{\sqrt{1+sin2x}}\) (với \(sin2x\ne-1\))
\(\Leftrightarrow1+2\left|sinx.cosx\right|=\dfrac{1}{1+sin2x}\)
\(\Leftrightarrow1+\left|sin2x\right|=\dfrac{1}{1+sin2x}\)
TH1: \(-1< sin2x< 0\Rightarrow1-sin2x=\dfrac{1}{1+sin2x}\)
\(\Leftrightarrow1-sin^22x=1\Rightarrow sin2x=0\) (loại)
TH2: \(0\le sin2x\le1\Rightarrow1+sin2x=\dfrac{1}{1+sin2x}\)
\(\Leftrightarrow1+sin2x=1\Leftrightarrow sin2x=0\Rightarrow x=\dfrac{k\pi}{2}\)
Vậy \(\left[{}\begin{matrix}x=\dfrac{\pi}{4}+\dfrac{k\pi}{2}\\x=\dfrac{k\pi}{2}\end{matrix}\right.\)
Bạn tự tìm số giá trị nhé
@Nguyễn Việt Lâm giúp em với