Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Lời giải:
a. $-x^2-2x-8=-7-(x^2+2x+1)=-7-(x+1)^2$
Vì $(x+1)^2\geq 0$ với mọi $x\in\mathbb{R}$ nên
$-x^2-2x-8=-7-(x+1)^2\leq -7< 0$ với mọi $x\in\mathbb{R}$
Vậy biểu thức luôn nhận giá trị âm với mọi $x$
b.
$-x^2-5x-11=-11+2,5^2-(x^2+5x+2,5^2)< -11+3^2-(x+2,5)^2$
$=-2-(x+2,5)^2\leq -2< 0$ với mọi $x\in\mathbb{R}$ (đpcm)
c.
$-4x^2-4x-2=-1-(4x^2+4x+1)=-1-(2x+1)^2\leq -1< 0$ với mọi $x\in\mathbb{R}$ (đpcm)
d.
$-9x^2+6x-7=-6-(9x^2-6x+1)=-6-(3x-1)^2\leq -6< 0$ với mọi $x\in\mathbb{R}$ (đpcm)

a) 6x(5x + 3) + 3x(1 – 10x) = 7
⇒ 30x2+18x+3x-30x2=7
⇒21x=7
⇒x=\(\dfrac{7}{21}\)
⇒x= \(\dfrac{1}{3}\)
b) (3x – 3)(5 – 21x) + (7x + 4)(9x – 5) = 44
⇒15x-63x2-15+63x + 63x2-35x+36x-20=44
⇒79x-35=44
⇒79x=44+35
⇒79x=79
⇒x=1

a: \(=\dfrac{x^3-3x^2-7x+x^2-3x-7}{x^2-3x-7}=x+1\)
b:\(=\dfrac{x^3+x^2+3x^2+3x+5x+5}{x+1}=x^2+3x+5\)
c:\(=\dfrac{x^3-3x^2-7x+2x^2-6x-14}{x^2-3x-7}=x+2\)
d: \(=\dfrac{x^2\left(x+5\right)+5x+25-25}{x+5}=x^2+5-\dfrac{25}{x+5}\)

`@` `\text {Ans}`
`\downarrow`

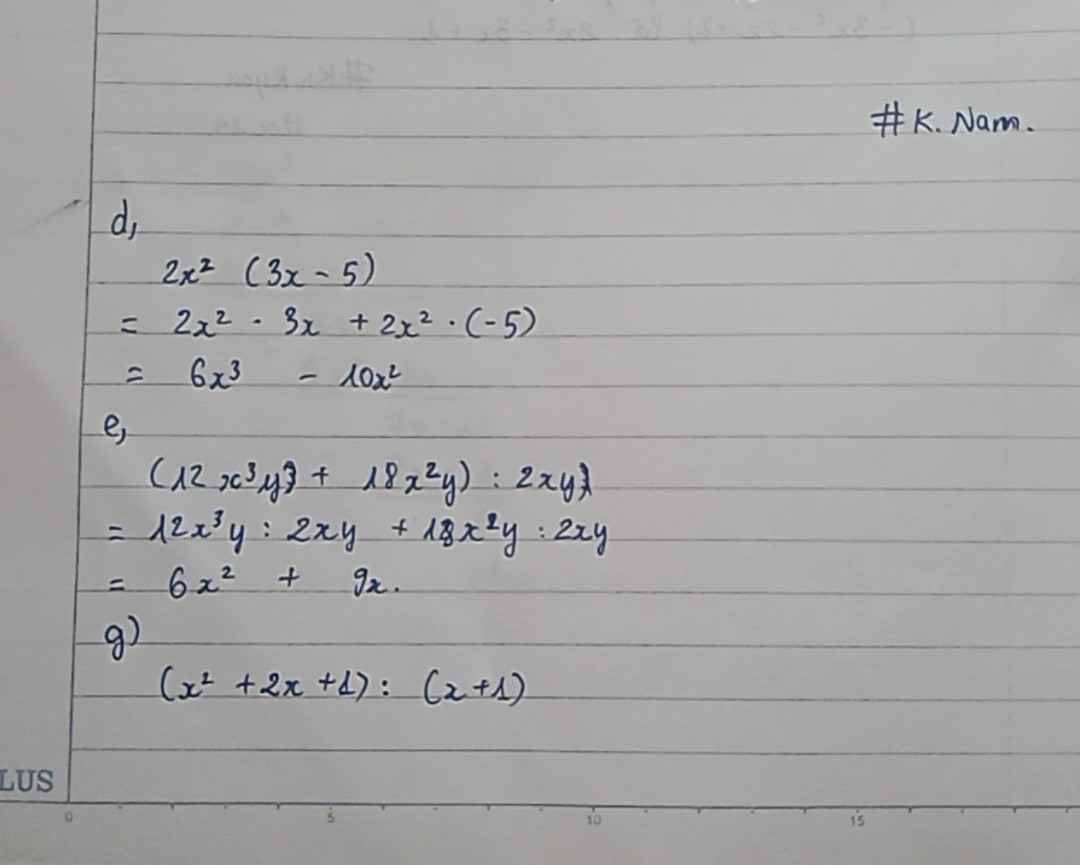

*Máy tớ cam hơi mờ, cậu thông cảm ._.*
Cậu viết lại rõ đề câu c, nhé.

a) \(A=x^2-4x+1=\left(x-2\right)^2-3\ge-3\)
\(minA=-3\Leftrightarrow x=2\)
b) \(B=-x^2-8x+5=-\left(x+4\right)^2+21\le21\)
\(maxB=21\Leftrightarrow x=-4\)
c) \(C=2x^2-8x+19=2\left(x-2\right)^2+11\ge11\)
\(minC=11\Leftrightarrow x=2\)
d) \(D=-3x^2-6x+1=-3\left(x+1\right)^2+4\le4\)
\(maxD=4\Leftrightarrow x=-1\)

\(âP\left(x\right)=13x^3+4x^2-11x-2\)
\(b.Q\left(x\right)=x^3+9x-5\)
\(c.A\left(x\right)=14x^3-x^2+10x+14\)
\(d.B\left(x\right)=2x^2+x+3\)
1, A = x^2 + 6x + 2018
= x^2 + 2.x.3 + 3^2 - 3^2 + 2018
= (x + 3)^2 -3^2 + 2018
= (x + 3)^2 + 2009
=>. GTNN of A là 2009
Mình cũng không chắc nữa, nếu đúng thì các ý khác bạn tham khảo nhé
\(A=x^2+6x+2018\)
\(A=\left(x^2+6x+9\right)+2009\)
\(A=\left(x+3\right)^2+2009\)
Mà \(\left(x+3\right)^2\ge0\forall x\)
\(\Rightarrow A\ge2009\)
Dấu "=" xảy ra khi : \(x+3=0\Leftrightarrow x=-3\)
Vậy ...
\(B=x^2-5x+20\)
\(B=\left(x^2-5x+\frac{25}{4}\right)+\frac{55}{4}\)
\(B=\left(x-\frac{5}{2}\right)^2+\frac{55}{4}\)
Mà \(\left(x-\frac{5}{2}\right)^2\ge0\forall x\)
\(\Rightarrow B\ge\frac{55}{4}\)
Dấu "=" xảy ra khi : \(x-\frac{5}{2}=0\Leftrightarrow x=\frac{5}{2}\)
Vậy ...
\(C=x^2+5x+10\)
\(C=\left(x^2+5x+\frac{25}{4}\right)+\frac{15}{4}\)
\(C=\left(x+\frac{5}{2}\right)^2+\frac{15}{4}\)
Mà \(\left(x+\frac{5}{2}\right)^2\ge0\forall x\)
\(\Rightarrow C\ge\frac{15}{4}\)
Dấu "=" xảy ra khi : \(x+\frac{5}{2}=0\Leftrightarrow x=-\frac{5}{2}\)
Vậy ...
\(D=x^2+10x-30\)
\(D=\left(x^2+10x+25\right)-55\)
\(D=\left(x+5\right)^2-55\)
Mà \(\left(x+5\right)^2\ge0\forall x\)
\(\Rightarrow D\ge-55\)
Dấu "=" xảy ra khi : \(x+5=0\Leftrightarrow x=-5\)
Vậy ...