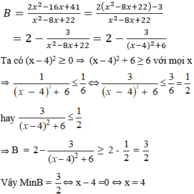LT
0
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
T
0
DT
0
NT
1
20 tháng 12 2017
A = 2.(x^2-8x+22)-1/x^2-8x+22 = 2 - 1/x^2-8x+22
Có : x^2-8x+22 = (x^2-8x+16)+6 = (x-4)^2+6 >= 6 => 1/x^2-8x+22 < = 1/6
=> A = 2 - 1/x^2-8x+22 >= 2-1/6 = 11/6
Dấu "=" xảy ra <=> x-4 = 0 <=> x=4
Vậy GTNN của A = 11/6 <=> x=4
k mk nha
PT
1
31 tháng 7 2015
bài 1
1, A= x2-2x6+62-3=(x-6)2-3
vì (x-6)2>=0 với mọi x ( lũy thùa bậc chẵn)
=> (x-6)2-3 <=-3
dấu = xảy ra <=> x-6=0
x=6
vậy Amax=-3 tại x=6
ý b tương tự chỉ cần đẩy -16 ra ngoài rồi làm như ý a
bài 2 nhóm x2+2x và y2 -6y
tách 10 thứ tự 1;3;6 rồi làm như trên
tách 10 ra thứ tự
TV
1
1 tháng 1 2022
\(A=\dfrac{2x^2-8x+17}{x^2-2x+1}\left(x\ne1\right)\)
\(\Leftrightarrow A\left(x^2-2x+1\right)=2x^2-8x+17\)
\(\Leftrightarrow Ax^2-2Ax+A=2x^2-8x+17\)
\(\Leftrightarrow x^2\left(A-2\right)-2x\left(A-4\right)+A-17=0\left(1\right)\)
\(A-2=0\Leftrightarrow A=2\Leftrightarrow x=3,75\left(tm\right)\left(2\right)\)
\(A-2\ne0\Leftrightarrow A\ne2\Rightarrow\Delta'\ge0\Leftrightarrow\left(A-4\right)^2-\left(A-17\right)\left(A-2\right)\ge0\Leftrightarrow A\ge\dfrac{18}{11}\Rightarrow A_{min}=\dfrac{18}{11}\Leftrightarrow x=\dfrac{13}{2}\left(tm\right)\left(3\right)\)
\(\left(2\right)và\left(3\right)\Rightarrow A_{min}=\dfrac{18}{11}\Leftrightarrow x=\dfrac{13}{2}\)
3 tháng 12 2021
c: \(-x^2+2x-2=-\left(x-1\right)^2-1\le-1\forall x\)
\(\Leftrightarrow V\ge-1\forall x\)
Dấu '=' xảy ra khi x=1
HD
0
