Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

c) Đặt \(A=\left(x^2+3x+1\right)\left(x^2+3x+2\right)-6\)
Đặt \(x^2+3x+1,5=a\)
\(\Rightarrow A=\left(a-0,5\right)\left(a+0,5\right)-6\)
\(\Rightarrow A=a^2-0,25-6\)
\(\Rightarrow A=a^2-\frac{25}{4}\)
\(\Rightarrow A=\left(a-\frac{5}{2}\right)\left(a+\frac{5}{2}\right)\)
Thay \(a=x^2+3x+0,5\)vào A ta có :
\(A=\left(x^2+3x+0,5-\frac{5}{2}\right)\left(x^2+3x+0,5+\frac{5}{2}\right)\)
\(A=\left(x^2+3x-2\right)\left(x^2+3x+3\right)\)
c, Đặt \(x^2+3x+2=a\)
Ta có : \(\left(a-1\right)a-6=a^2-a-6=\left(a^2-3a\right)+\left(2a-6\right)\)
\(=a\left(a-3\right)+2\left(a-3\right)\)
\(=\left(a+2\right)\left(a-3\right)\)
\(=\left(x^2+3x+4\right)\left(x^2+3x-1\right)\)
Câu d làm tương tự .
Gợi ý : (x+3)(x+5) = x2 + 8x + 15
đặt bằng a rồi giải tiếp

Rút gọn thôi chứ phân tích sao được ._.
( x - 3 )2 - ( 4x + 5 )2 - 9( x + 1 )2 - 6( x - 3 )( x + 1 )
= x2 - 6x + 9 - ( 16x2 + 40x + 25 ) - 9( x2 + 2x + 1 ) - 6( x2 - 2x - 3 )
= x2 - 6x + 9 - 16x2 - 40x - 25 - 9x2 - 18x - 9 - 6x2 + 12x + 18
= -30x2 - 52x - 7
Sửa đề lại 1 chút là phân tích được mà bn Quỳnh:))
Ta có: \(\left(x-3\right)^2-\left(4x+5\right)^2+9\left(x+1\right)^2-6\left(x-3\right)\left(x+1\right)\)
\(=\left[\left(x-3\right)^2-6\left(x-3\right)\left(x+1\right)+9\left(x+1\right)^2\right]-\left(4x+5\right)^2\)
\(=\left(x-3-9x-9\right)^2-\left(4x+5\right)^2\)
\(=\left(8x+12\right)^2-\left(4x+5\right)^2\)
\(=\left(4x+7\right)\left(12x+17\right)\)

(x - 5)2 - 4(x - 3)2 + 2(2x - 1)(x - 5) + (2x - 1)2
= [(x - 5)2 + 2(2x - 1)(x - 5) + (2x - 1)2) - [2(x - 3)]2
= (x - 5 + 2x - 1)2 - (2x - 6)2
= (3x - 6)2 - (2x - 6)2
= (3x - 6 - 2x + 6)(3x - 6 + 2x - 6) = x(5x - 12)
( x - 5 )2 - 4( x - 3 )2 + 2( 2x - 1 )( x - 5 ) + ( 2x - 1 )2
= [ ( x - 5 )2 + 2( 2x - 1 )( x - 5 ) + ( 2x - 1 )2 ] - 22( x - 3 )2
= ( x - 5 + 2x - 1 )2 - ( 2x - 6 )2
= ( 3x - 6 )2 - ( 2x - 6 )2
= ( 3x - 6 - 2x + 6 )( 3x - 6 + 2x - 6 )
= x( 5x - 12 )

Bạn nên tách bài ra để đăng. Không nên đăng 1 loạt như thế này.

1: \(=\left(x^2+x\right)^2+3\left(x^2+x\right)+2-12\)
=(x^2+x)^2+3(x^2+x)-10
=(x^2+x+5)(x^2+x-2)
=(x^2+x+5)(x+2)(x-1)
2: \(=\left(x^2+5ax+4a^2\right)\left(x^2+5ax+6a^2\right)+a^4\)
\(=\left(x^2+5ax\right)^2+10a^2\left(x^2+5ax\right)+25a^2\)
\(=\left(x^2+5ax+5a^2\right)^2\)
3: \(=\left(a+b\right)^3+c^3-3ab\left(a+b\right)-3abc\)
\(=\left(a+b+c\right)\left(a^2+2ab+b^2-ac-bc+c^2\right)-3ab\left(a+b+c\right)\)
\(=\left(a+b+c\right)\left(a^2+b^2+c^2-ab-ac-bc\right)\)
5: \(M=\left(n+1\right)\left(n^2+2n\right)+360\)
=n(n+1)(n+2)+360 chia hết cho 6
6A
7D

Ta có:
(x + 2)(x + 3)(x + 4)(x + 5) - 24
= [(x + 2)(x + 5)][(x + 3)(x + 4)] - 24
= (x2 + 5x + 2x + 10)(x2 + 4x + 3x + 12) - 24
= (x2 + 7x + 10)(x2 + 7x + 12) - 24
Đặt x2 + 7x + 10 = k
=> k(k + 2) - 24 = k2 + 2k - 24 = k2 + 6x - 4x - 24
= k(k + 6) - 4(k + 6)
= (k - 4)(k + 6)
=> (x + 2)(x + 3)(x + 4)(x + 5) - 24
= (x2 + 7x + 10 - 4)(x2 + 7x + 10 + 6)
= (x2 + 7x + 6)(x2 + 7x + 16)
\(\left(x+2\right)\left(x+3\right)\left(x+4\right)\left(x+5\right)-24\)
\(=\left[\left(x+2\right)\left(x+5\right)\right]\left[\left(x+3\right)\left(x+4\right)\right]-24\)
\(=\left(x^2+7x+10\right)\left(x^2+7x+12\right)-24\)(1)
Đặt \(x^2+7x+11=t\)thay vào (1) ta được:
\(\left(t-1\right)\left(t+1\right)-24\)
\(=t^2-1-24\)
\(=t^2-25\)
\(=\left(t-5\right)\left(t+5\right)\)Thay \(t=x^2+7x+11\)ta được:
\(\left(x^2+7x+11-5\right)\left(x^2+7x+11+5\right)\)
\(=\left(x^2+7x+6\right)\left(x^2+7x+16\right)\)
\(=\left(x^2+x+6x+6\right)\left(x^2+7x+16\right)\)
\(=\left[x\left(x+1\right)+6\left(x+1\right)\right]\left(x^2+7x+16\right)\)
\(=\left(x+1\right)\left(x+6\right)\left(x^2+7x+16\right)\)
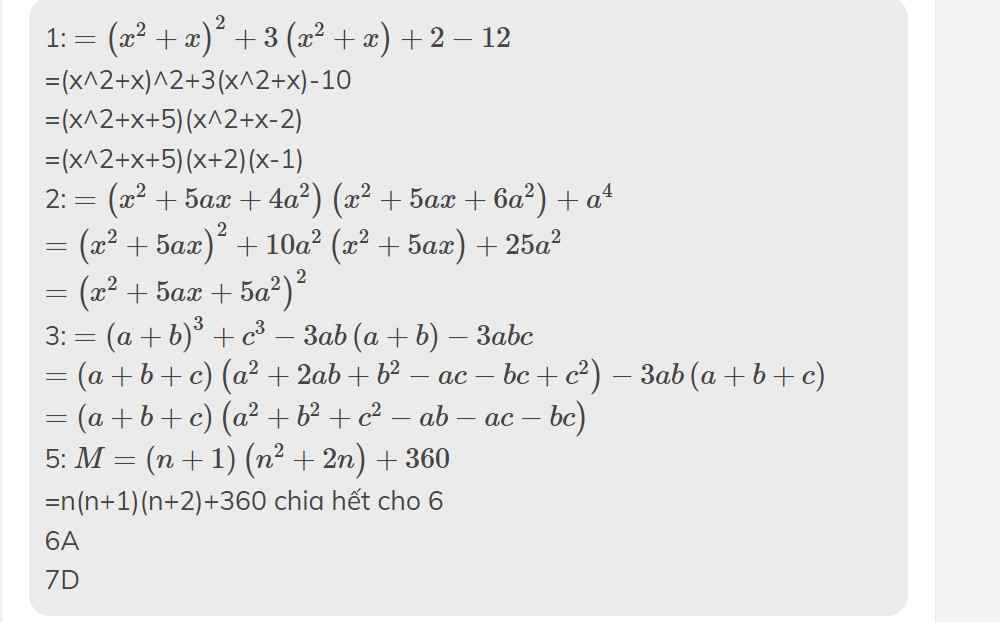
(x+1)(x+3)(x+5)(x+8)+15
=[(x+1)(x+7)][(x+3)(x+5)]+15
=(x2+8x+7)(x2+8x+15)+15
Đặt t=x2+8x+7
=>x2+8x+15=t+8
=>(x2 +8x+7)(x2+8x+15)+15
=t(t+8)+15
=t2+8t+15
=t2+3t+5t+15
=t(t+3)+5(t+3)
=(t+3)(t+5)
=(x2+8x+10)(x2+8x+12)
Đặt \(A=\left(x+1\right)\left(x+3\right)\left(x+5\right)\left(x+7\right)+15\)
\(\Rightarrow A=\left(x+1\right)\left(x+7\right)\left(x+3\right)\left(x+5\right)+15\)
\(=\left(x^2+8x+7\right)\left(x^2+8x+15\right)+15\)
Đặt \(x^2+8x+11=t\)
\(\Rightarrow A=\left(t-4\right)\left(t+4\right)+15=t^2-16+15=t^2-1=\left(t+1\right)\left(t-1\right)\)
\(=\left(x^2+8x+11+1\right)\left(x^2+8x+11-1\right)=\left(x^2+8x+12\right)\left(x^2+8x+10\right)\)
\(=\left(x^2+2x+6x+12\right)\left(x^2+8x+10\right)\)\(=\left[x\left(x+2\right)+6\left(x+2\right)\right]\left(x^2+8x+10\right)\)
\(=\left(x+2\right)\left(x+6\right)\left(x^2+8x+10\right)\)