Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Đây là một dạng phân tích thừa số nguyên tố khá quen, cô sẽ hướng dẫn e nhé :) Ta cần ghép các hạng tử để xuất hiện các thành phần chứa biến giống nhau.
\(A=\left(4x+1\right)\left(12x-1\right)\left(3x+2\right)\left(x+1\right)-4=\left(4x+1\right)\left(3x+2\right)\left(12x-1\right)\left(x+1\right)-4\)
\(=\left(12x^2+11x+2\right)\left(12x^2+11x-1\right)-4\)
Đặt \(12x^2+11x+2=t\Rightarrow A=t\left(t-3\right)-4=t^2-3t-4=\left(t-4\right)\left(t+1\right)\)
Quay lại biến x ta có: \(A=\left(12x^2+11x-2\right)\left(12x^2+11x+3\right)\)
Câu sau tương tự nhé :)

a) \(=x^4-14x^2+40-72=x^4-14x^2-32=\left(x-4\right)\left(x+4\right)\left(x^2+2\right)\)
b) \(=\left(x^2+5x+4\right)\left(x^2+5x+6\right)+1=\left(x^2+5x\right)^2+2\left(x^2+5x\right)+1=\left(x^2+5x+1\right)^2\)
c) \(=x^4+3x^3-3x^2+3x^3+9x^2-9x+x^2+3x-3-5=x^4+6x^3+7x^2-6x-8=\left(x-1\right)\left(x+1\right)\left(x+2\right)\left(x+4\right)\)
a: Ta có: \(\left(x^2-4\right)\left(x^2-10\right)-72\)
\(=x^4-14x^2-32\)
\(=\left(x^2-16\right)\left(x^2+2\right)\)
\(=\left(x-4\right)\left(x+4\right)\left(x^2+2\right)\)
b: Ta có: \(\left(x+1\right)\left(x+2\right)\left(x+3\right)\left(x+4\right)+1\)
\(=\left(x^2+5x+6\right)\left(x^2+5x+4\right)+1\)
\(=\left(x^2+5x\right)^2+10\left(x^2+5x\right)+24+1\)
\(=\left(x^2+5x+1\right)^2\)
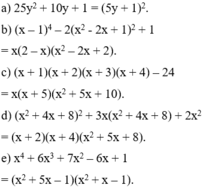
a)
\((x+2)(x+3)(x+4)(x+5)-24\)
\(=[[(x+2)(x+5)]][(x+3)(x+4)]-24\)
\(=(x^2+7x+10)(x^2+7x+12)-24\)
\(=a(a+2)-24\) (đặt $x^2+7x+10=a$)
\(=a^2+2a-24=a^2+6a-4a-24\)
\(=a(a+6)-4(a+6)=(a-4)(a+6)\)
\(=(x^2+7x+6)(x^2+7x+16)\)
\(=(x+1)(x+6)(x^2+7x+16)\)
b)
\((4x+1)(12x-1)(3x+2)(x+1)-4\)
\(=[(4x+1)(3x+2)][(12x-1)(x+1)]-4\)
\(=(12x^2+11x+2)(12x^2+11x-1)-4\)
\(=(a+2)(a-1)-4\) (đặt $12x^2+11x=a$)
\(=a^2+a-6=a^2+3a-2a-6=a(a+3)-2(a+3)\)
\(=(a-2)(a+3)=(12x^2+11x-2)(12x^2+11x+3)\)
c)
Đặt $x^2+3x+1=a$. Khi đó:
\((x^2+3x+1)(x^2+3x+2)-6=a(a+1)-6\)
\(=a^2+3a-2a-6=a(a+3)-2(a+3)=(a-2)(a+3)\)
\(=(x^2+3x-1)(x^2+3x+4)\)
d)
\(4(x+5)(x+6)(x+10)(x+12)-3x^2\)
\(=4[(x+5)(x+12)][(x+6)(x+10)]-3x^2\)
\(=4(x^2+17x+60)(x^2+16x+60)-3x^2\)
\(=4(a+x)a-3x^2\) (đặt \(x^2+16x+60=a\))
\(=4a^2+4ax-3x^2=4a^2-2ax+6ax-3x^2\)
\(=2a(2a-x)+3x(2a-x)=(2a-x)(2a+3x)\)
\(=(2x^2+32x+120-x)(2x^2+32x+120+3x)\)
\(=(2x^2+31x+120)(2x^2+35x+120)\)
\(=[2x(x+8)+15(x+8)](2x^2+35x+120)\)
\(=(2x+15)(x+8)(2x^2+35x+120)\)