Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

\(P=\frac{8x^3-12x^2+6x-1}{4x^2-4x+1}\)
a) ĐKXĐ: x \(\ne\pm\frac{1}{2}\)
b) Theo đề bài ta có:
\(2x^2+x=0\)
\(\Rightarrow x\left(2x+1\right)=0\)
\(\Rightarrow\orbr{\begin{cases}x=0\\2x+1=0\end{cases}\Rightarrow\orbr{\begin{cases}x=0\\x=\frac{-1}{2}\left(Loại\right)\end{cases}}}\)
Thay x = 0 (thỏa mãn điều kiện) vào P ta có:
\(P=\frac{0-0+0-1}{0-0+1}=\frac{-1}{1}=-1\)
Vậy khi x = 0 thì P = -1
c) \(P=\frac{8x^3-12x^2+6x-1}{4x^2-4x+1}=\frac{\left(2x-1\right)^3}{\left(2x-1\right)^2}=2x-1\)
Để P \(\inℤ\Leftrightarrow2x-1\inℤ\)
Mà -1\(\inℤ;x\inℤ\Rightarrow-1⋮2x\)
\(\Rightarrow2x\inƯ\left(-1\right)=\left\{1;-1\right\}\)
Ta có bảng giá trị:
| 2x | 1 | -1 |
| x | \(\frac{1}{2}\) | \(-\frac{1}{2}\) |
| Loại | Loại |
Vậy không có x thỏa mãn P \(\inℤ\)
d) Với x \(\ne\pm\frac{1}{2};P=2\)
\(\Leftrightarrow2x-1=2\)
\(\Leftrightarrow2x=3\)
\(\Leftrightarrow x=\frac{3}{2}\)
Vậy \(x=\frac{3}{2}\)thì \(P=2\)

a, 4x^2 - 4x = -1
\(\Leftrightarrow\)4x^2 - 4x + 1 = 0
\(\Leftrightarrow\)(2x-1)2 =0
\(\Leftrightarrow\)2x - 1 = 0
\(\Leftrightarrow\)x = 1/2
b, \(\Leftrightarrow\)( 2x + 1)^3 = 0
\(\Leftrightarrow\)2x + 1 = 0
\(\Leftrightarrow\)x = -1/2
đúng thì
a) \(4x^2-4x=-1\)
\(\Leftrightarrow4x^2-4x+1=0\)
\(\Leftrightarrow\left(2x-1\right)^2=0\)
\(\Leftrightarrow2x-1=0\)
\(\Leftrightarrow x=\frac{1}{2}\)
b) \(8x^3+12x^2+6x+1=0\)
\(\Leftrightarrow\left(2x+1\right)^3=0\)
\(\Leftrightarrow2x+1=0\)
\(\Leftrightarrow x=-\frac{1}{2}\)

a) ĐKXĐ: 4x2-4x+1>0
(2x)2-2.2x+1= (2x-1)2≥ 0
b) P= \(\frac{8x^3-12x^2+6x-1}{4x^2-4x+1}\) = \(\frac{\left(2x\right)^3-3.\left(2x\right)^2.1+3.2x.1-1}{\left(2x-1\right)^2}\) = \(\frac{\left(2x-1\right)^3}{\left(2x-1\right)^2}\) = 2x-1 c) ta có: x ∈ Z ⇒ 2.x ∈ Z ⇔2x-1 ∈ Z
a) ĐKXĐ của phương trình : \(4x^2+4x+1\ne0\)\(\Rightarrow x\ne-\frac{1}{2}\)
b) \(P=\frac{4x^3+8x^2-x-2}{4x^2+4x+1}\)
\(\Rightarrow P=\frac{\left(4x^3-x\right)+\left(8x^2-2\right)}{\left(2x+1\right)^2}\)
\(\Rightarrow P=\frac{x\left(4x^2-1\right)+2\left(4x^2-1\right)}{\left(2x+1\right)^2}\)
\(\Rightarrow P\left(x\right)=\frac{\left(x+2\right)\left(2x-1\right)\left(2x+1\right)}{\left(2x+1\right)^2}\)
\(\Rightarrow P\left(x\right)=\frac{\left(x+2\right)\left(2x-1\right)}{\left(2x+1\right)}=\frac{3}{2}\)\(\Rightarrow P\left(x\right)=2\left(x+2\right)\left(2x-1\right)=3\left(2x+1\right)\)
\(\Rightarrow P\left(x\right)=4x^2+6x-6-\left(6x+3\right)=0\)
\(\Rightarrow P\left(x\right)=4x^2-9=0\)\(\Rightarrow P\left(x\right)=x^2=\frac{9}{4}\)
\(\Rightarrow P\left(x\right)=x^2=\sqrt{\frac{9}{4}}\)\(\Rightarrow P\left(x\right)=\frac{3}{2}\)
câu c) cx tương tự

a) (x + 3)2 - (x - 2)2 = 2x
=> (x + 3 - x + 2)(x + 3 + x - 2) = 2x
=> 5(2x + 1) = 2x
=> 10x + 5 = 2x
=> 10x - 2x = -5
=> 8x = -5
=> x = -5/8
b) 7x(x - 2) = x - 2
=> 7x(x - 2) - (x - 2) = 0
=> (7x - 1)(x - 2) = 0
=> \(\orbr{\begin{cases}7x-1=0\\x-2=0\end{cases}}\)
=> \(\orbr{\begin{cases}x=\frac{1}{7}\\x=2\end{cases}}\)
c) 8x3 - 12x2 + 6x - 1 = 0
=> (2x - 1)3 = 0
=> 2x - 1 = 0
=> 2x = 1
=> x = 1/2

Bài 1.
a) ( 7x - 3 )2 - 5x( 9x + 2 ) - 4x2 = 18
<=> 49x2 - 42x + 9 - 45x2 - 10x - 4x2 = 18
<=> -52x + 9 = 18
<=> -52x = 9
<=> x = -9/52
b) ( x - 7 )2 - 9( x + 4 )2 = 0
<=> x2 - 14x + 49 - 9( x2 + 8x + 16 ) = 0
<=> x2 - 14x + 49 - 9x2 - 72x - 144 = 0
<=> -8x2 - 86x - 95 = 0
<=> -8x2 - 10x - 76x - 95 = 0
<=> -8x( x + 5/4 ) - 76( x + 5/4 ) = 0
<=> ( x + 5/4 )( -8x - 76 ) = 0
<=> \(\orbr{\begin{cases}x+\frac{5}{4}=0\\-8x-76=0\end{cases}}\Leftrightarrow\orbr{\begin{cases}x=-\frac{5}{4}\\x=-\frac{19}{2}\end{cases}}\)
c) ( 2x + 1 )2 + ( 4x - 1 )( x + 5 ) = 36
<=> 4x2 + 4x + 1 + 4x2 + 19x - 5 = 36
<=> 8x2 + 23x - 4 - 36 = 0
<=> 8x2 + 23x - 40 = 0
=> Vô nghiệm ( lớp 8 chưa học nghiệm vô tỉ nghen ) :))
Bài 2.
a) x2 - 12x + 39 = ( x2 - 12x + 36 ) + 3 = ( x - 6 )2 + 3 ≥ 3 > 0 ∀ x ( đpcm )
b) 17 - 8x + x2 = ( x2 - 8x + 16 ) + 1 = ( x - 4 )2 + 1 ≥ 1 > 0 ∀ x ( đpcm )
c) -x2 + 6x - 11 = -( x2 - 6x + 9 ) - 2 = -( x - 3 )2 - 2 ≤ -2 < 0 ∀ x ( đpcm )
d) -x2 + 18x - 83 = -( x2 - 18x + 81 ) - 2 = -( x - 9 )2 - 2 ≤ -2 < 0 ∀ x ( đpcm )

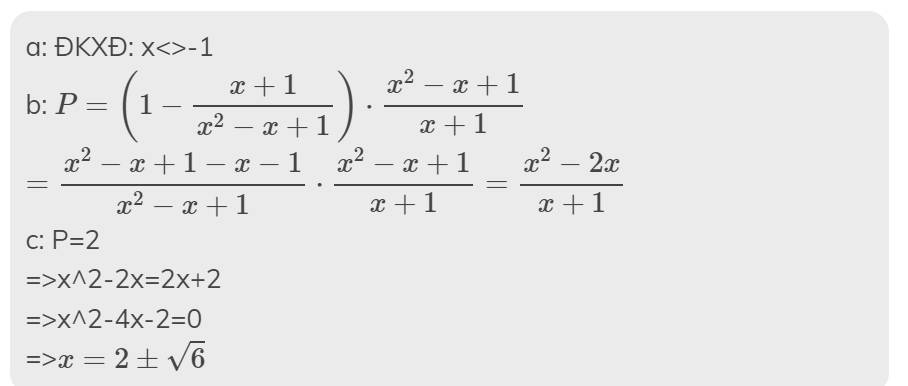
a: ĐKXĐ: x<>-1
b: \(P=\left(1-\dfrac{x+1}{x^2-x+1}\right)\cdot\dfrac{x^2-x+1}{x+1}\)
\(=\dfrac{x^2-x+1-x-1}{x^2-x+1}\cdot\dfrac{x^2-x+1}{x+1}=\dfrac{x^2-2x}{x+1}\)
c: P=2
=>x^2-2x=2x+2
=>x^2-4x-2=0
=>\(x=2\pm\sqrt{6}\)
mai mình nộp bài r ai đó giúp mình với huhu
a) ĐKXĐ: \(4x^2-4x+1\ne0\)
Ta sẽ giải phương trình \(4x^2-4x+1=0\) để loại các nghiệm:
\(4x^2-4x+1=4\left(x^2-x-\frac{1}{4}\right)=4\left(x-\frac{1}{2}\right)^2\)
Để \(4x^2-4x+1=0\) thì \(4\left(x-\frac{1}{2}\right)^2=0\Leftrightarrow\left(x-\frac{1}{2}\right)^2=0\Leftrightarrow x=\frac{1}{2}\)
Vậy ĐKXĐ: \(x\ne\frac{1}{2}\)
b) \(P=\frac{8x^3-12x^2+6x-1}{4x^2-4x+1}=\frac{8\left(x-\frac{1}{2}\right)^3}{4\left(x-\frac{1}{2}\right)^2}=2x-1\) (chịu khó ngồi phân tích cả tử và mẫu thành nhân tử giúp mình)
c) Ta có: \(P=2x-1\).Với mọi x nguyên thì \(2x\) nguyên.
Do vậy \(P=2x-1\)nguyên.
Suy ra đpcm.