K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
BT
2
14 tháng 8 2021
d: \(\left(\dfrac{1}{2}x-1\right)\left(2x-3\right)\)
\(=x^2-\dfrac{3}{2}x-2x+3\)
\(=x^2-\dfrac{7}{2}x+3\)
e: Ta có: \(\left(x-7\right)\left(x-5\right)\)
\(=x^2-5x-7x+35\)
\(=x^2-12x+35\)
f: Ta có: \(\left(x-\dfrac{1}{2}\right)\left(x+\dfrac{1}{2}\right)\left(4x-1\right)\)
\(=4\left(x-\dfrac{1}{4}\right)\left(x-\dfrac{1}{4}\right)\)
\(=4\left(x-\dfrac{1}{4}\right)^2\)
\(=4\left(x^2-\dfrac{1}{2}x+\dfrac{1}{16}\right)\)
\(=4x^2-2x+\dfrac{1}{4}\)
28 tháng 5 2017
Câu 1:
\(3x\left(12x+4\right)+9x\left(4x+3\right)\)
\(\Leftrightarrow3x\left(12x+4\right)+3x\left[3.\left(4x+3\right)\right]\)
\(\Leftrightarrow3x\left(12x+4\right)+3x\left(12x+6\right)\)
\(\Leftrightarrow3x\left[12x+4+12x+6\right]\)
\(\Leftrightarrow3x.\left(24x+10\right)\)
\(\Leftrightarrow72x^2+30x\)
Câu 2:
\(x\left(5+2x\right)+2x^2\left(x-1\right)\)
\(\Leftrightarrow5x+2x^2+2x^3-2x^2\)
\(\Leftrightarrow2x^3+5x\)
T
28 tháng 5 2017
Đề 1
- Use different phrasing or notations
- Enter whole words instead of abbreviations
- Avoid mixing mathemaal and other notations
- Check your spelling
- Give your input in English
- Wolfram|Alpha answers specific questions rather than explaining general topicsEnter "2 cups of sugar", not "nutrition information"
- You can only get answers about objective factsTry "highest mountain", not "most beautiful painting"
- Only what is known is known to Wolfram|AlphaAsk "how many men in Mauritania", not "how many monsters in Loch Ness"
- Only public information is availableRequest "GDP of France", not "home phone of Michael Jordan"
- Approximate form
TN
6
NV
Nguyễn Việt Lâm
Giáo viên
21 tháng 8 2021
\(a^2-4b^2=a^2-\left(2b\right)^2=\left(a-2b\right)\left(a+2b\right)\)
TN
3
C
21 tháng 8 2021
\(5x\left(x-2\right)-x+2=0\)
\(\Rightarrow5x\left(x-2\right)-\left(x-2\right)=0\)
\(\Rightarrow\left(5x-1\right)\left(x-2\right)=0\)
\(\Rightarrow\left[{}\begin{matrix}5x-1=0\\x-2=0\end{matrix}\right.\Rightarrow\left[{}\begin{matrix}x=\dfrac{1}{5}\\x=2\end{matrix}\right.\)
Vậy x=1/5 hoặc x=2
DG
1
H
3 tháng 11 2021
\(x^2-2x-3=x^2-3x+x-3=x\left(x-3\right)+\left(x-3\right)=\left(x+1\right)\left(x-3\right)\)
23 tháng 6 2017
\(x^3+y^3+z^3-3xyz\)
\(=\left(x^3+3x^2y+3xy^2+y^3\right)+z^3-3x^2y-3xy^2-3xyz\)
\(=\left[\left(x+y\right)^3+z^3\right]-3xy\left(x+y+z\right)\)
\(=\left(x+y+z\right)\left(x^2+y^2+z^2-xy-xz+2xy\right)-3xy\left(x+y+z\right)\)
\(=\left(x+y+z\right)\left(x^2+y^2+z^2-xy-xz-yz\right)\)
TH
23 tháng 6 2017
Giỏi toán cần phải cọ xát nhiếu;
\(x^3+y^3+z^3-3xyz=\left(x^3+3x^2y+3xy^2+y^3\right)+z^3-3abc-3x^2y-3xy^2\)
Bạn thêm vào 2 hạng tử , sau đó bớt 2 hạng tử để biểu thức ko thay đổi nhé, ở đây xuất hiện 1 hằng đẳng thức:
\(=\left(x+y\right)^3+z^3-3xy\left(x+y+z\right)\)
Ta thấy lại tiếp tục xuất hiên 1 hằng đẳng thức: a^3+b^3 nên ta có:
\(=\left(x+y+z\right)\left(\left(x+y\right)^2-\left(x+y\right)z+z^2\right)-3xy\left(x+y+z\right)\)
\(=\left(x+y+z\right)\left(x^2+2xy+y^2-xz-yz+z^2-3xy\right)\)
\(=\left(x+y+z\right)\left(x^2+y^2+z^2-xy-yz-xz\right)\)
ủng hộ nha các bạn
19 tháng 6 2016
\(2x^2+5x-3=\left(2x^2-x\right)+\left(6x-3\right)\)\(=x\left(2x-1\right)+3\left(2x-1\right)=\left(x+3\right)\left(2x-1\right)\)
DG
1
H
3 tháng 11 2021
\(x^2-4x-y^2+4=\left(x^2-4x+4\right)-y^2=\left(x-2\right)^2-y^2=\left(x-2-y\right)\left(x-2+y\right)\)
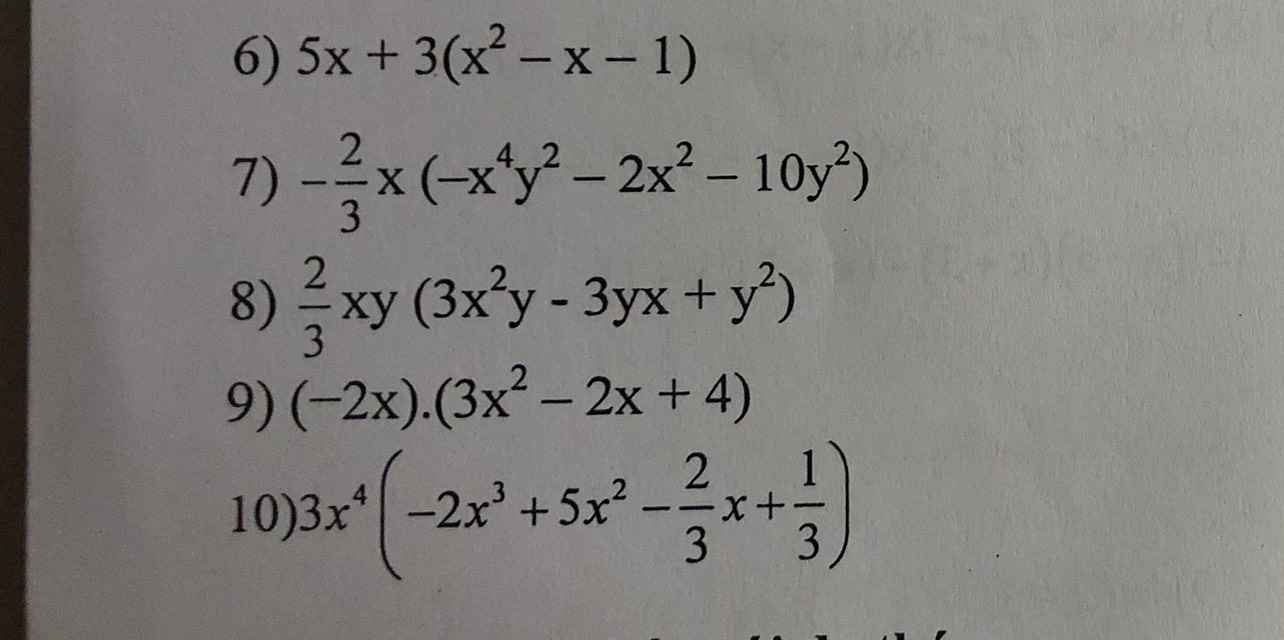
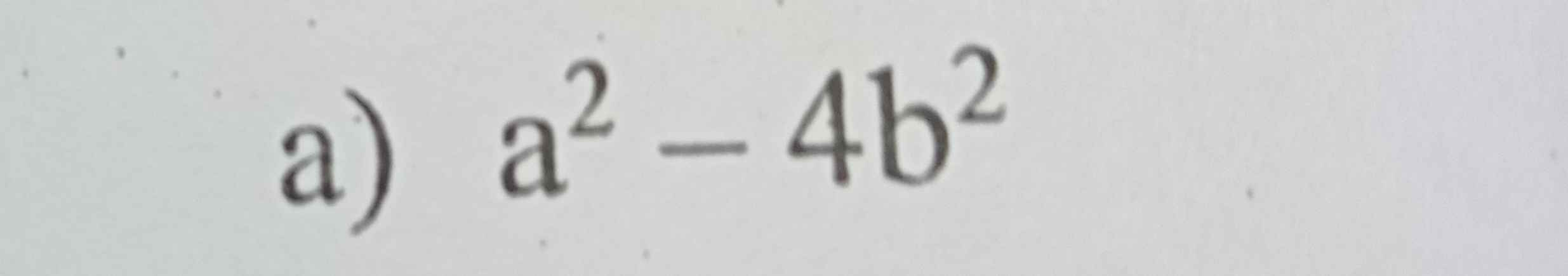
9: \(-2x\left(3x^2-2x+4\right)=-6x^3+4x^2-8x\)
8: \(\dfrac{2}{3}xy\left(3x^2y-3xy+y^2\right)=2x^3y^2-2x^2y^2+\dfrac{2}{3}xy^3\)