K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
28 tháng 2 2022
\(a)7x-21=0.\\ \Leftrightarrow x=3.\)
\(b)3x-5=2x+7.\\ \Leftrightarrow x=12.\)
\(c)6x+18=0.\\ \Leftrightarrow x=-3.\\ d)5x+2=4x-1.\\ \Leftrightarrow x=-3.\)
28 tháng 2 2022
Bài 1:
a: 7x-21=0
=>7x=21
hay x=3
b: 3x-5=2x+7
=>3x-2x=7+5
=>x=12
c: 6x+18=0
=>6x=-18
hay x=-3
d: 5x+2=4x-1
=>5x-4x=-1-2
=>x=-3
11 tháng 6 2021
do tam giác ABC có góc A=90\(^o\)=>\(\Delta ABC\) vuông tại A
\(\Delta ABH\sim\Delta CBA\left(g.g\right)\)( vì góc B chung, góc AHB=góc BAC)
\(=>\dfrac{AB}{BC}=\dfrac{BH}{BA}=>AB^2=BH.BC\)(1)
b,dựa vào (1)\(=>AB=\sqrt{BH.BC}=\sqrt{BH\left(BH+HC\right)}=\sqrt{4.\left(4+9\right)}=2\sqrt{13}cm\)
theo pytago\(=>AC=\sqrt{BC^2-AB^2}=\sqrt{13^2-\left(2\sqrt{13}\right)^2}=3\sqrt{13}cm\)
c,theo tính chất phan giác=>\(\dfrac{AB}{BC}=\dfrac{AD}{DC}=>\dfrac{AD}{DC}=\dfrac{2}{3}\)\(=>DC=\dfrac{3}{2}AD\)
có: \(AD+DC=AC=>AD+\dfrac{3}{2}AD=3\sqrt{13}=>AD=\dfrac{6\sqrt{13}}{5}cm\)
\(=>S\left(\Delta DBA\right)=\dfrac{AB.AD}{2}=15,6cm^2\)
có: tam giac BDA đồng dạng tam giác BEH(g.g)(do góc B1=góc B2, góc A=góc H=90 độ)
=>góc E2= góc D1
mà góc E2=góc E1(đối đỉnh)=>góc D1=góc E1=>tam giác AED cân tại A
=>AE=AD=\(\dfrac{6\sqrt{13}}{5}cm\),
theo pytago=>AH=\(\sqrt{AB^2-BH^2}=\sqrt{ \left(2\sqrt{13}\right)^2-4^2}=6cm\)
=>EH=AH-AE=\(6-\dfrac{6\sqrt{13}}{5}cm\)
=>\(S\left(\Delta EBH\right)=\dfrac{1}{2}BH.EH\) rồi tự tính ra rồi lập tỉ số 2 S tam giác (mỏi tay)
DX
17 tháng 12 2022
11)\(\dfrac{3x+1}{x-5}+\dfrac{2x}{x-5}=\dfrac{3x+2x+1}{x-5}=\dfrac{5x+1}{x-5}\)
12)\(\dfrac{4-x^2}{x-3}+\dfrac{2}{x^2-9}=\dfrac{4-x^2}{x-3}+\dfrac{2}{\left(x-3\right)\left(x+3\right)}=\dfrac{\left(4-x^2\right)\left(x+3\right)}{\left(x-3\right)\left(x+3\right)}+\dfrac{2}{\left(x-3\right)\left(x+3\right)}=\dfrac{2+\left(2-x\right)\left(2+x\right)\left(x+3\right)}{\left(x-3\right)\left(x+3\right)}\)
13)
\(\dfrac{3}{4x-2}+\dfrac{2x}{4x^2-1}=\dfrac{3}{2\left(2x-1\right)}+\dfrac{2x}{\left(2x-1\right)\left(2x+1\right)}=\dfrac{3\left(2x+1\right)}{2\left(2x-1\right)\left(2x+1\right)}+\dfrac{2.2x}{2\left(2x-1\right)\left(2x+1\right)}=\dfrac{6x+3+4x}{2\left(2x-1\right)\left(2x+1\right)}=\dfrac{10x+3}{2\left(2x-1\right)\left(2x+1\right)}\)
14)
\(\dfrac{2x+1}{2x-4}+\dfrac{5}{x^2-4}=\dfrac{2x+1}{2\left(x-2\right)}+\dfrac{5}{\left(x-2\right)\left(x+2\right)}=\dfrac{\left(2x+1\right)\left(x+2\right)}{2\left(x-2\right)\left(x+2\right)}+\dfrac{5.2}{2\left(x-2\right)\left(x+2\right)}=\dfrac{2x^2+5x+12}{2\left(x-2\right)\left(x+2\right)}\)
AB
0
30 tháng 4 2022
\(\dfrac{x+1}{39}+\dfrac{x+2}{38}+\dfrac{x+3}{37}=0\)
\(\Leftrightarrow\dfrac{x+1}{39}+1+\dfrac{x+2}{38}+1+\dfrac{x+3}{37}+1-3=0\)
\(\Leftrightarrow\dfrac{x+40}{39}+\dfrac{x+40}{38}+\dfrac{x+40}{37}=3\)
\(\Leftrightarrow\left(x+40\right)\left(\dfrac{1}{39}+\dfrac{1}{38}+\dfrac{1}{37}\right)=3\)
\(\Leftrightarrow\left(x+40\right).\dfrac{4331}{54834}=3\)
\(\Leftrightarrow x+40=\dfrac{164502}{4331}\)
\(\Leftrightarrow x=\dfrac{-8738}{4331}\)
-Vậy \(S=\left\{\dfrac{-8738}{4331}\right\}\)
16 tháng 12 2020
Ta có (x+1)^3 - (x-1)^3
=(x3+3x2+3x+1)-(x3-3x2+3x-1)
= x3 + 3x2 +3x +1 - x3 + 3x2 -3x + 1
=6x2 + 2
Vậy biểu thức này có phụ thuộc vào biến x (vì vẫn còn 6x2)
Chúc bạn học tốt!
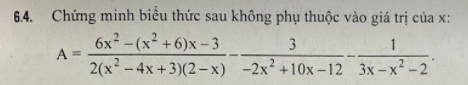
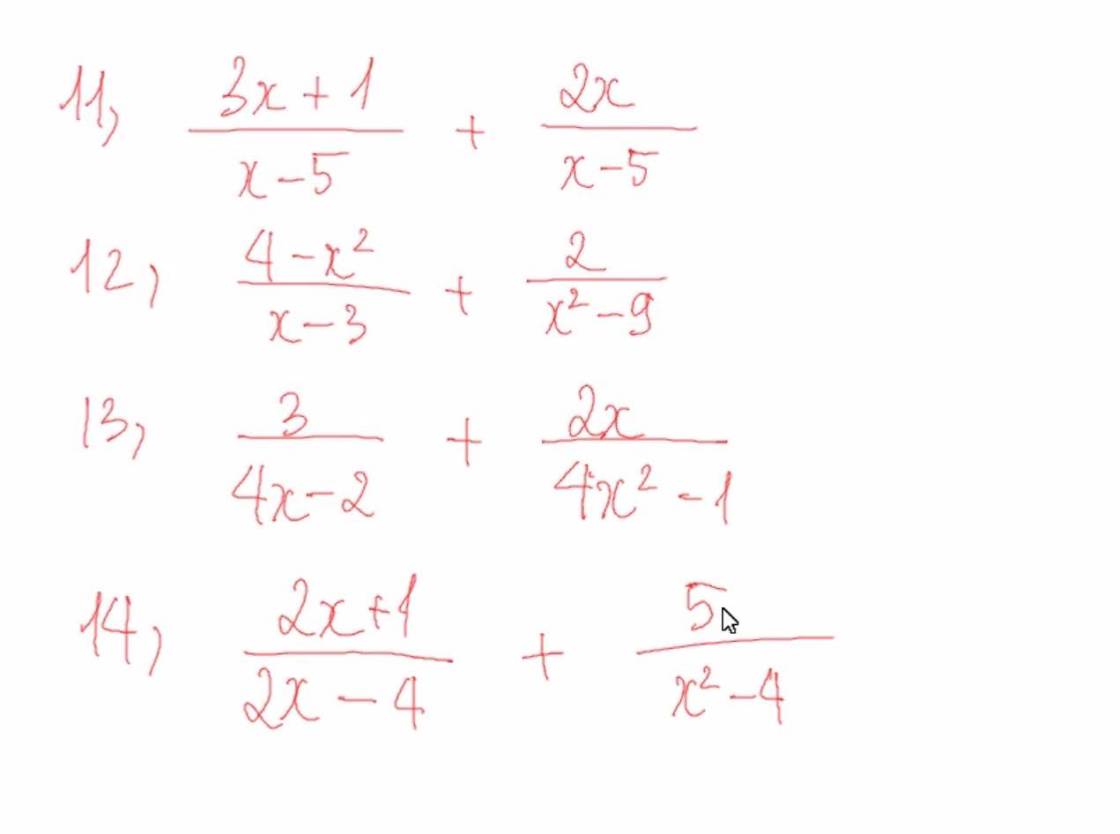
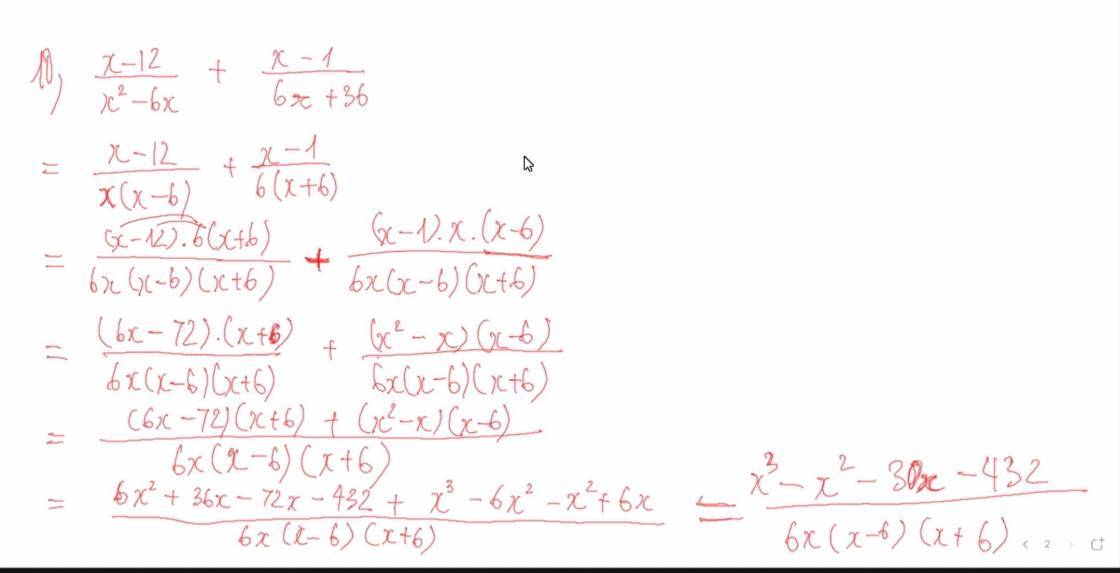
giải phân thức ra là xong