K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
10 tháng 10 2021
f: Ta có: \(\sqrt{2-x}=\sqrt{x^2-2x+4}\)
\(\Leftrightarrow x^2-2x+4=2-x\)
\(\Leftrightarrow x^2-x+2=0\)
\(\Leftrightarrow x\in\varnothing\)
TM
1
15 tháng 10 2021
\(1,\\ a,=\dfrac{12\left(3+\sqrt{3}\right)}{6}=2\left(3+\sqrt{3}\right)\\ b,=\dfrac{8\left(\sqrt{5}-2\right)}{1}=8\left(\sqrt{5}-2\right)\\ c,=\dfrac{14\left(\sqrt{10}-\sqrt{3}\right)}{7}=2\left(\sqrt{10}-\sqrt{3}\right)\)
7 tháng 10 2021
4: Ta có: \(\sqrt{3+2\sqrt{2}}+\sqrt{6-4\sqrt{2}}\)
\(=\sqrt{2}+1+2-\sqrt{2}\)
=3
7 tháng 10 2021
5: Ta có: \(2+\sqrt{17-4\sqrt{9}+4\sqrt{5}}\)
\(=2+\sqrt{5+4\sqrt{5}}\)
6: Ta có: \(2\sqrt{3}+\sqrt{2}+\sqrt{18-8\sqrt{2}}\)
\(=2\sqrt{3}+\sqrt{2}+4-\sqrt{2}\)
\(=4+2\sqrt{3}\)
AT
5 tháng 7 2021
a) (d) cắt trục hoành tại điểm có hoành độ bằng 2
\(\Rightarrow\) tọa độ điểm đó là \(\left(2;0\right)\)
\(\Rightarrow0=2a-3\Rightarrow a=\dfrac{3}{2}\Rightarrow\left(d\right):y=\dfrac{3}{2}x-3\)
b) Vì (d) song song với đồ thị của hàm \(y=2x+1\)
\(\Rightarrow\left\{{}\begin{matrix}a=2\\-3\ne1\end{matrix}\right.\Rightarrow a=2\Rightarrow\left(d\right):y=2x-3\)
c) Gọi A là giao điểm của (d) và (d')
\(\Rightarrow x_A=1\Rightarrow y_A=2+3=5\Rightarrow A\left(1;5\right)\)
\(\Rightarrow5=a-3\Rightarrow a=8\Rightarrow\left(d\right):y=8x-3\)
7 tháng 7 2021
a) Thay a=3 vào (d), ta được:
y=3x+b
Vì (d): y=3x+b cắt trục hoành tại điểm có hoành độ bằng 2 nên
Thay x=2 và y=0 vào (d), ta được:
\(3\cdot2+b=0\)
\(\Leftrightarrow b=-6\)
Vậy: (d): y=3x-6
b) Thay a=2 vào (d), ta được:
y=2x+b
Thay x=1 và y=6 vào (d), ta được:
\(b+2\cdot1=6\)
hay b=4
Vậy: (d): y=2x+4
23 tháng 7 2021
a) Ta có: \(\sqrt{12+2\sqrt{35}}-\sqrt{12-2\sqrt{35}}\)
\(=\sqrt{7}+\sqrt{5}-\sqrt{7}+\sqrt{5}\)
\(=2\sqrt{5}\)
b) Ta có: \(\left(\dfrac{5+\sqrt{5}}{\sqrt{5}+1}+2\right)\left(\dfrac{5-\sqrt{5}}{\sqrt{5}-1}-2\right)\)
\(=\left(\sqrt{5}+2\right)\left(\sqrt{5}-2\right)\)
=1
c) Ta có: \(\dfrac{7\sqrt{2}+2\sqrt{7}}{\sqrt{14}}-\dfrac{5}{\sqrt{7}+\sqrt{2}}\)
\(=\sqrt{7}+\sqrt{2}-\sqrt{7}+\sqrt{2}\)
\(=2\sqrt{2}\)
AH
Akai Haruma
Giáo viên
30 tháng 10 2021
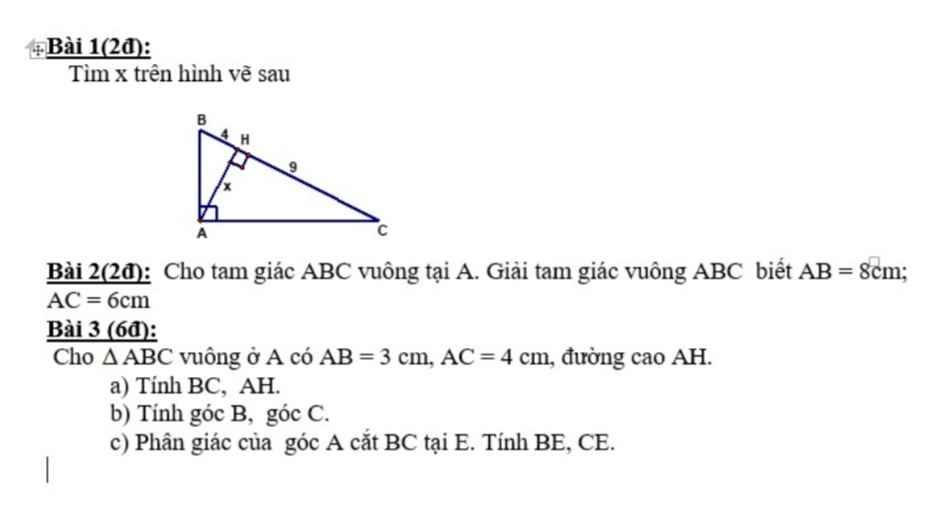
Bài 1:
Áp dụng HTL trong tam giác vuông:
$AH^2=BH.CH$
$\Leftrightarrow x^2=4.9=36$
$\Rightarrow x=6$ (do $x>0$)
AH
Akai Haruma
Giáo viên
30 tháng 10 2021
Bài 2:
Áp dụng định lý Pitago:
$BC=\sqrt{AB^2+AC^2}=\sqrt{6^2+8^2}=10$ (cm)
$\sin B=\frac{AC}{BC}=\frac{6}{10}=\frac{3}{5}$
$\Rightarrow \widehat{B}=36,87^0$
$\widehat{C}=90^0-\widehat{B}=90^0-36,87^0=53,13^0$
