K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
23 tháng 7 2021
b) Gọi (d3): y=ax+b
Vì (d3)//(d1) nên \(a=-\dfrac{2}{3}\)
Vậy: (d3): \(y=\dfrac{-2}{3}x+b\)
Thay x=6 vào (d2), ta được:
\(y=-2\cdot6+4=-12+4=-8\)
Thay x=6 và y=-8 vào (d3), ta được:
\(\dfrac{-2}{3}\cdot6+b=-8\)
\(\Leftrightarrow b=-4\)
Vậy: (d3): \(y=\dfrac{-2}{3}x-4\)
LA
1
31 tháng 7 2021
b) Để P nguyên thì \(\sqrt{x}+5⋮3\sqrt{x}-1\)
\(\Leftrightarrow3\sqrt{x}+15⋮3\sqrt{x}-1\)
\(\Leftrightarrow16⋮3\sqrt{x}-1\)
\(\Leftrightarrow3\sqrt{x}-1\in\left\{-1;1;2;4;8;16\right\}\)
\(\Leftrightarrow3\sqrt{x}\in\left\{0;2;3;5;9;17\right\}\)
\(\Leftrightarrow3\sqrt{x}\in\left\{0;3;9\right\}\)
\(\Leftrightarrow\sqrt{x}\in\left\{0;1;3\right\}\)
hay \(x\in\left\{0;1;9\right\}\)
29 tháng 8 2021
thay \(x=3-2\sqrt{2}\) vào P ta có:
\(\dfrac{x+8}{\sqrt{x}+1}=\dfrac{3-2\sqrt{2}+8}{\sqrt{3-2\sqrt{2}}+1}=\dfrac{11-2\sqrt{2}}{\sqrt{2}-1+1}=\dfrac{11-2\sqrt{2}}{\sqrt{2}}\)
29 tháng 8 2021
\(b,x=3-2\sqrt{2}=\left(\sqrt{2}-1\right)^2\)
Thay vào P, ta được:
\(P=\dfrac{3-2\sqrt{2}+8}{\sqrt{\left(\sqrt{2}-1\right)^2}+1}=\dfrac{11-2\sqrt{2}}{\sqrt{2}}=\dfrac{11\sqrt{2}-4}{2}\)
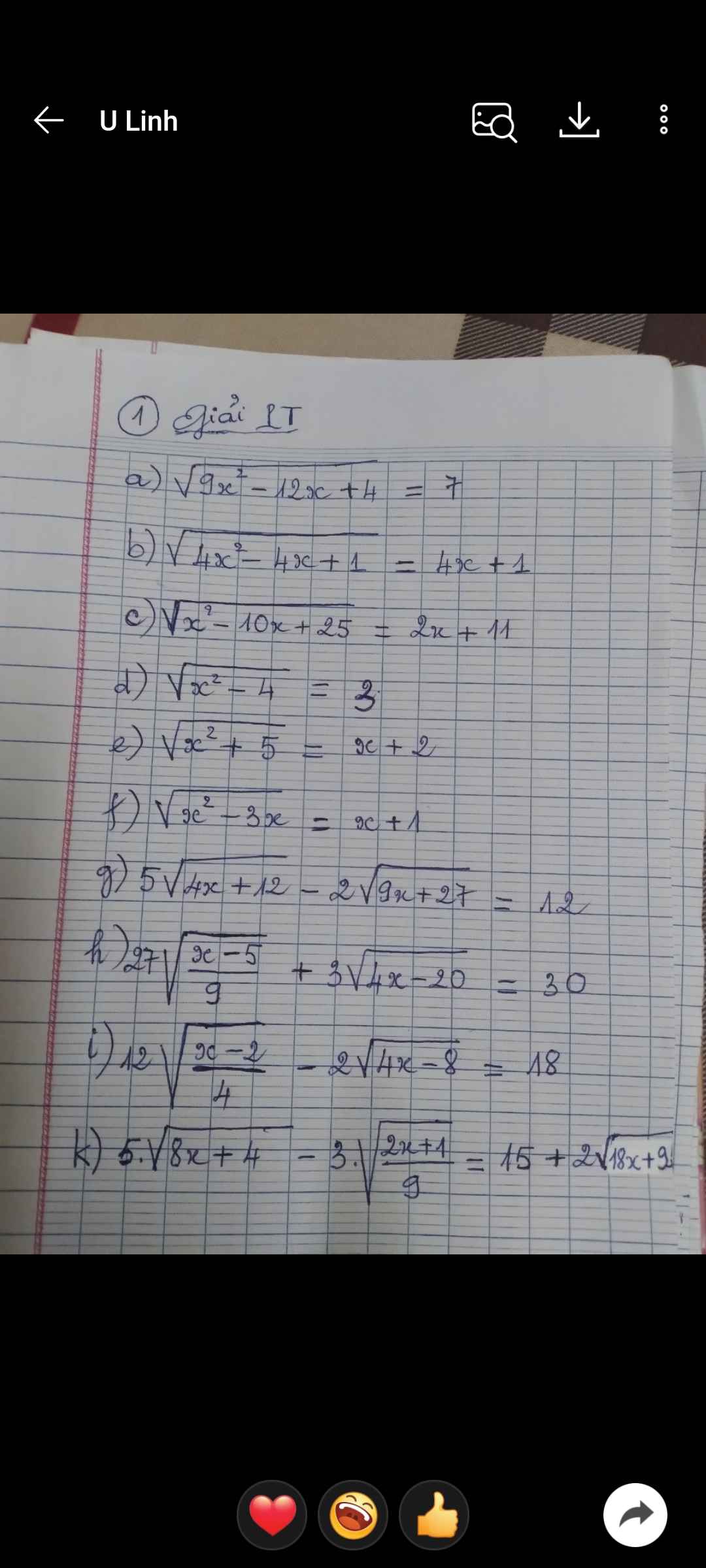
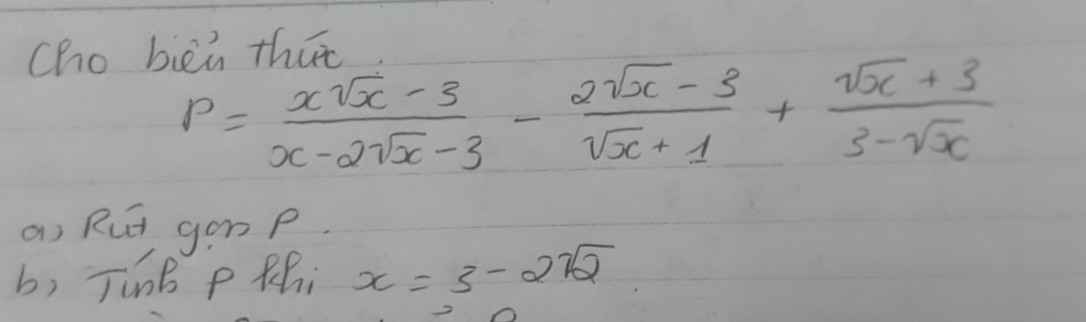
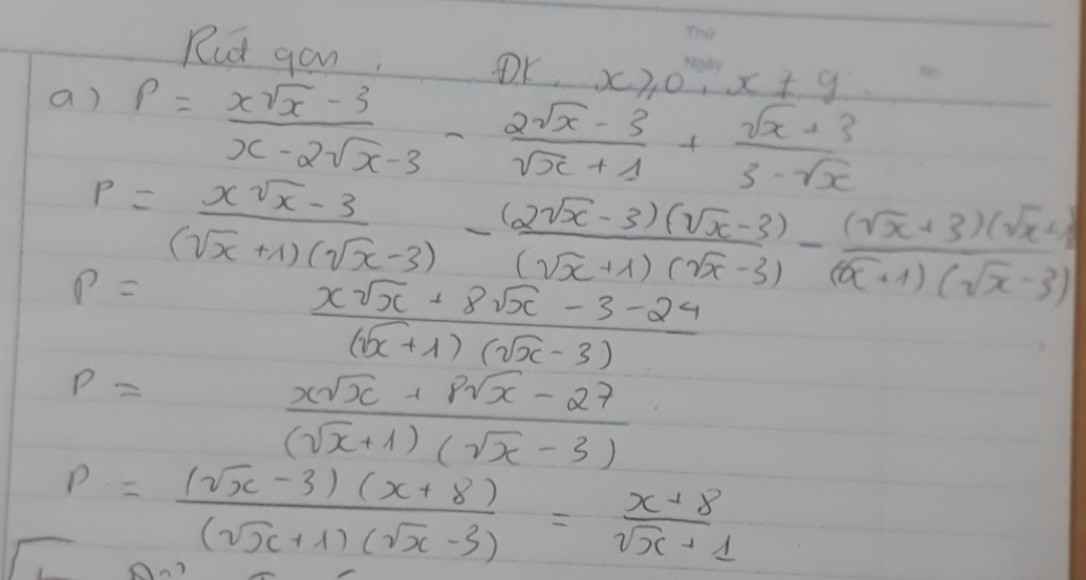
a, bạn tự vẽ
b, Hoành độ giao điểm tm pt
\(\dfrac{x^2}{2}=\left(2m-1\right)x+m+2\Leftrightarrow x^2-2\left(2m-1\right)x-2m-4=0\)
\(\Delta'=\left(2m-1\right)^2-\left(-2m-4\right)=4m^2-2m+5\)
Để (P) cắt (d) tại 2 điểm pb khi \(4m^2-2m+5>0\)