K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
LT
0
AH
Akai Haruma
Giáo viên
19 tháng 11 2017
Câu 69:
Ta có:
\(f(x)+f(y)=1\Leftrightarrow \frac{9^x}{9^x+m^2}+\frac{9^y}{9^y+m^2}=1\)
\(\Leftrightarrow \frac{9^x}{9^x+m^2}=1-\frac{9^y}{9^y+m^2}=\frac{m^2}{9^y+m^2}\)
\(\Leftrightarrow 9^{x+y}=m^4\Leftrightarrow (3^{x+y}-m^2)(3^{x+y}+m^2)=0\)
\(\Rightarrow 3^{x+y}=m^2\) (do \(3^{x+y}>0; m^2\geq 0\Rightarrow 3^{x+y}+m^2>0\) ) (1)
------------------------------------------------
Tiếp theo: \(e^{x+y}\leq e(x+y)\Leftrightarrow e^{x+y-1}\leq x+y\)
Đặt \(x+y=k\Rightarrow e^{k-1}\leq k\Leftrightarrow e^{k-1}-k\leq 0\)
Đặt \(e^{k-1}-k=f(k)\Rightarrow f(k)\leq 0(*)\)
Có: \(f'(k)=e^{k-1}-1=0\Leftrightarrow k=1\)
Lập bảng biến thiên ta thấy rằng \(f(k)_{\min}=f(1)=0\) hay \(f(k)\geq 0(**)\)
Từ \((1);(2)\Rightarrow f(k)=0\) hay \(k=1\Leftrightarrow x+y=1\)
Thay vào (1) ta có \(m^2=3\Leftrightarrow m=\pm \sqrt{3}\)
Vậy có 2 giá trị m thỏa mãn. đáp án D
AH
Akai Haruma
Giáo viên
19 tháng 11 2017
Câu 70:
Để hai pt lần lượt có hai nghiệm phân biệt thì
\(\Delta _1=\Delta_2=b^2-20a>0\Leftrightarrow b^2> 20a\) (1)
Khi đó, áp dụng hệ thức Viete ta có:
Đối với PT 1: \(\ln x_1+\ln x_2=\frac{-b}{a}\Leftrightarrow \ln (x_1x_2)=\frac{-b}{a}\)
\(\Leftrightarrow x_1x_2=e^{\frac{-b}{a}}\)
Đối với PT 2: \(\log x_1+\log x_2=\frac{-b}{5}\Leftrightarrow \log (x_1x_2)=\frac{-b}{5}\)
\(\Leftrightarrow x_3x_4=10^{\frac{-b}{5}}\)
Vì \(x_1x_2> x_3x_4\Leftrightarrow e^{\frac{-b}{a}}>10^{\frac{-b}{5}}\)
\(\Leftrightarrow 10^{\frac{-b}{a\ln 10}}> 10^{\frac{-b}{5}}\)
\(\Leftrightarrow \frac{-b}{a\ln 10}>\frac{-b}{5}\Leftrightarrow a>\frac{5}{\ln 10}\)
\(\Leftrightarrow a> 2,71...\Rightarrow a\geq 3\) (vì a nguyên dương)
Theo (1) ta có: \(b^2>20a\geq 60\Rightarrow b\geq 8\) (do b nguyên dương)
Vậy \(2a+3b\geq 2.3+3.8\Leftrightarrow 2a+3b\geq 30\)
Đáp án A
AH
Akai Haruma
Giáo viên
28 tháng 5 2023
Lời giải:
\(\log_2^2x+\log_2(\frac{x}{4})=0\)
$\Leftrightarrow \log_2^2x+\log_2x+\log_2(\frac{1}{4})=0$
$\Leftrightarrow \log_2^2x+\log_2x-2=0$
$\Leftrightarrow (\log_2x-1)(\log_2x+2)=0$
\Leftrightarrow \log_2x=1$ hoặc $\log_2x=-2$
$\Leftrightarrow x=2$ hoặc $x=\frac{1}{4}$
Tích các nghiệm: $2.\frac{1}{4}=\frac{1}{2}$
Đáp án D
NV
Nguyễn Việt Lâm
Giáo viên
6 tháng 8 2021
ĐKXĐ:
1.
\(\left\{{}\begin{matrix}x-1>0\\log_2\left(x-1\right)-1>0\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}x>1\\x-1>2\end{matrix}\right.\) \(\Leftrightarrow x>3\)
2.
\(\left\{{}\begin{matrix}x-1>0\\x-1\ne1\\\dfrac{x}{2-x^2}>0\end{matrix}\right.\) \(\Leftrightarrow\left\{{}\begin{matrix}x>1\\x\ne2\\\left[{}\begin{matrix}x< -\sqrt{2}\\0< x< \sqrt{2}\end{matrix}\right.\end{matrix}\right.\)
\(\Rightarrow1< x< \sqrt{2}\)
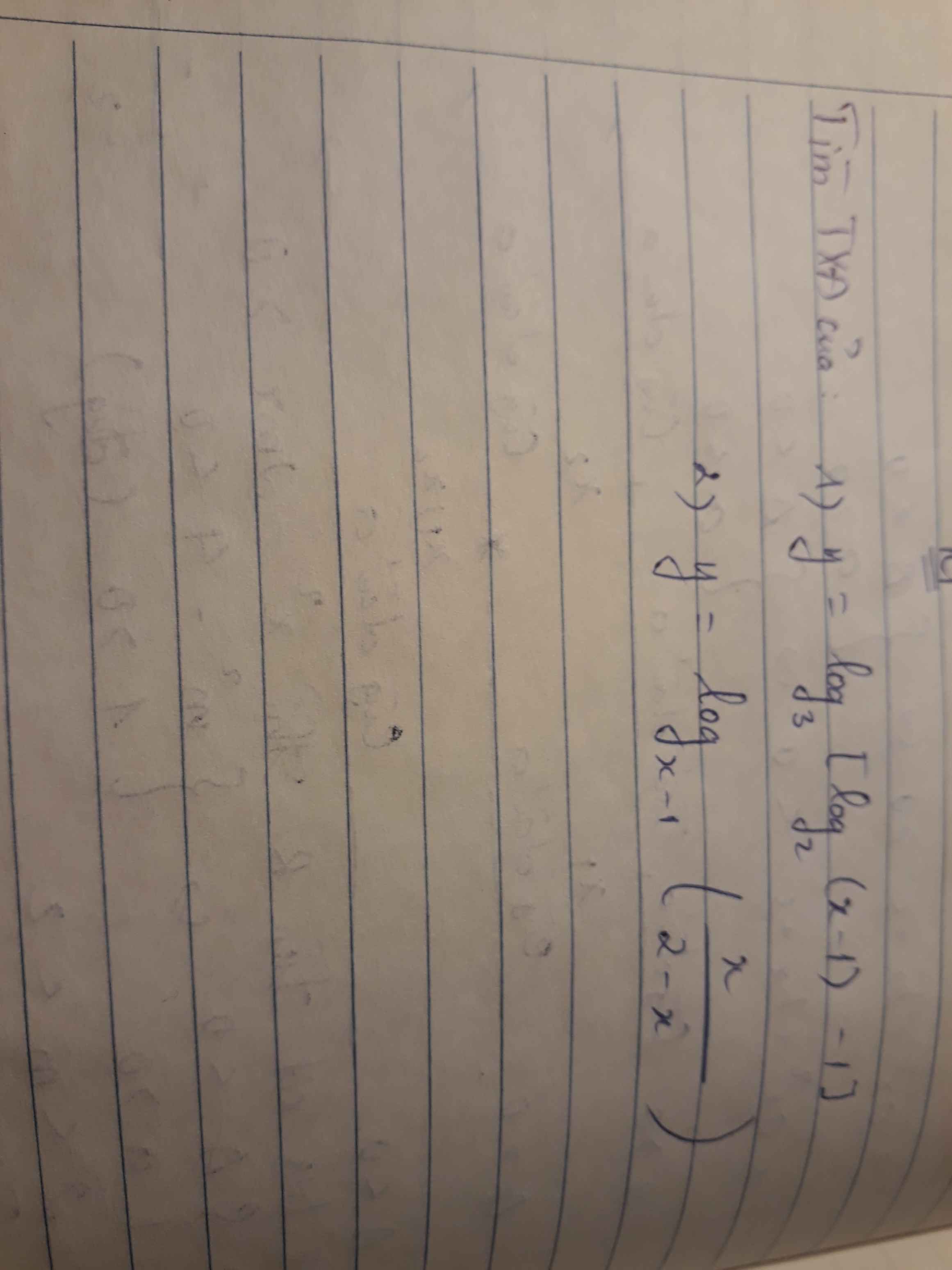
\(\int\left(\dfrac{7}{cos^2x}+cosx-3^x+2\right)dx=7tanx+sinx-\dfrac{3^x}{ln3}+2x+C\)
dạ e cảm ơn