Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Điều kiện x>0. Nhận thấy x=2 là nghiệm.
Nếu x>2 thì
\(\frac{x}{2}>\frac{x+2}{4}>1\); \(\frac{x+1}{3}>\frac{x+3}{5}>1\)
Suy ra
\(\log_2\frac{x}{2}>\log_2\frac{x+2}{4}>\log_4\frac{x+2}{4}\)hay :\(\log_2x>\log_2\left(x+2\right)\)
\(\log_3\frac{x+1}{3}>\log_3\frac{x+3}{5}>\log_5\frac{x+3}{5}\) hay \(\log_3\left(x+1\right)>\log_5\left(x+3\right)\)
Suy ra vế trái < vế phải, phương trình vô nghiệm.
Đáp số x=2

Đặt :
\(t=\sqrt{x^2-5x+5}\left(t\ge0\right)\)
Bất phương trình trở thành :
\(\log_2\left(t+1\right)+\log_3\left(t^2+2\right)\le2\)
Xét \(f\left(t\right)=\log_2\left(t+1\right)+\log_3\left(t^2+2\right)\) trên \(\left(0;+\infty\right)\)
Do \(t\ge0\) nên \(\log_2\left(t+1\right)\) và \(\log_3\left(t^2+2\right)\) đều là các hàm số đồng biến, do đó f(t) đồng biến trên \(\left(0;+\infty\right)\)
Lại có f(1)=2, từ đó suy ra \(t\le1\)Giải ra được :\(1\le x\)\(\le\frac{5-\sqrt{5}}{2}\) hoặc \(\frac{5-\sqrt{5}}{2}\le x\) \(\le4\)
Đặt \(\sqrt{x^2-5x+5}=t>0\)
\(\Rightarrow log_2\left(t+1\right)+log_3\left(t^2+2\right)-2=0\)
Nhận thấy \(t=1\) là 1 nghiệm của pt
Xét hàm \(f\left(t\right)=log_2\left(t+1\right)+log_3\left(t^2+2\right)-2\)
\(f'\left(t\right)=\dfrac{1}{\left(t+1\right)ln2}+\dfrac{2t}{\left(t^2+2\right)ln3}>0\Rightarrow f\left(t\right)\) đồng biến
\(\Rightarrow f\left(t\right)\) có tối đa 1 nghiệm
\(\Rightarrow t=1\) là nghiệm duy nhất của pt
\(\Rightarrow\sqrt{x^2-5x+5}=1\Rightarrow\left[{}\begin{matrix}x=1\\x=4\end{matrix}\right.\)

Điều kiện \(\begin{cases}x\ne1\\x>\frac{1}{2}\end{cases}\)
\(\log_3\left(x-1\right)^2+\log_{\sqrt{3}}\left(2x-1\right)=2\Leftrightarrow2\log_3\left|x-1\right|+2\log_3\left(2x-1\right)=2\)
\(\Leftrightarrow\log_3\left|x-1\right|\left(2x-1\right)=\log_33\)
\(\Leftrightarrow\left|x-1\right|\left(2x-1\right)=3\)
\(\frac{1}{2}\)<x<1 và \(2x^2-3x+4=0\)
hoặc x>1 và \(2x^2-3x-2=0\)
\(\Leftrightarrow x=2\) thỏa mãn điều kiện. Vậy x=2

a) Đặt t = 13x > 0 ta được phương trình:
13t2 – t – 12 = 0 ⇔ (t – 1)(13t + 12) = 0
⇔ t = 1 ⇔ 13x = 1 ⇔ x = 0
b)
Chia cả hai vế phương trình cho 9x ta được phương trình tương đương
(1+(23)x)(1+3.(23)x)=8.(23)x(1+(23)x)(1+3.(23)x)=8.(23)x
Đặt t=(23)xt=(23)x (t > 0) , ta được phương trình:
(1 + t)(1 + 3t) = 8t ⇔ 3t2 – 4t + 1 = 0 ⇔ t∈{13,1}t∈{13,1}
Với t=13t=13 ta được nghiệm x=log2313x=log2313
Với t = 1 ta được nghiệm x = 0
c) Điều kiện: x > 2
Vì nên phương trình đã cho tương đương với:
[log3(x−2)=0log5x=1⇔[x=3x=5[log3(x−2)=0log5x=1⇔[x=3x=5
d) Điều kiện: x > 0
log22x – 5log2x + 6 = 0
⇔(log2x – 2)(log2x – 3) = 0
⇔ x ∈ {4, 8}


a. \(y=\left(3^x-9\right)^{-2}\)
Điều kiện : \(3^x-9\ne0\Leftrightarrow3^x\ne3^2\)
\(\Leftrightarrow x\ne2\)
Vậy tập xác định là \(D=R\backslash\left\{2\right\}\)
b. \(y=\sqrt{\log_{\frac{1}{3}}\left(x-3\right)-1}\)
Điều kiện : \(\log_{\frac{1}{3}}\left(x-3\right)-1\ge0\Leftrightarrow\log_{\frac{1}{3}}\left(x-3\right)\ge1=\log_{\frac{1}{3}}\frac{1}{3}\)
\(\Leftrightarrow0< x-3\le\frac{1}{3}\)
\(\Leftrightarrow3< x\le\frac{10}{3}\)
Vậy tập xác định \(D=\) (3;\(\frac{10}{3}\)]
c. \(y=\sqrt{\log_3\sqrt{x^2-3x+2}+4-x}\)
Điều kiện :
\(\log_3\sqrt{x^2-3x+2}+4-x\ge0\Leftrightarrow x^2-3x+2+4-x\ge1\)
\(\Leftrightarrow\sqrt{x^2-3x+2}\ge-x-3\)
\(\Leftrightarrow\begin{cases}x-3< 0\\x^2-3x+2\ge0\end{cases}\) hoặc \(\begin{cases}x-3\ge0\\x^2-3x+2\ge\left(x-3\right)^2\end{cases}\)
\(\Leftrightarrow\left[\begin{array}{nghiempt}x\le1\\2\le x< 3\\x\ge3\end{array}\right.\) \(\Leftrightarrow\left[\begin{array}{nghiempt}x\le1\\x\ge2\end{array}\right.\)
Vậy tập xác định là : D=(\(-\infty;1\)]\(\cup\) [2;\(+\infty\) )


Điều kiện x>0.
Phương trình đã cho tương đương :
\(\log_3\left(x^2+2x\right)-\log_3\left(3x+2\right)=0\)
\(\Leftrightarrow\log_3\left(x^2+2x\right)=\log_3\left(3x+2\right)\)
\(\Leftrightarrow x^2+2x=3x+2\)
\(\Leftrightarrow x^2-x-2=0\)
\(\Leftrightarrow\begin{cases}x=-1\\x=2\end{cases}\)
Đối chiếu điều kiện ta có phương trình đã cho có nghiệm là \(x=2\)

a. Vì \(0< 0,1< 1\) nên bất phương trình đã cho
\(\Leftrightarrow0< x^2+x-2< x+3\)
\(\Leftrightarrow\left\{{}\begin{matrix}x^2+x-2>0\\x^2-5< 0\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}\left[{}\begin{matrix}x< -2\\x>1\end{matrix}\right.\\-\sqrt{5}< x< \sqrt{5}\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}-\sqrt{5}< x< -2\\1< x< \sqrt{5}\end{matrix}\right.\)
Vậy tập nghiệm của bất phương trình là \(S=\left\{-\sqrt{5};-2\right\}\) và \(\left\{1;\sqrt{5}\right\}\)
b. Điều kiện \(\left\{{}\begin{matrix}2-x>0\\x^2-6x+5>0\end{matrix}\right.\)
Ta có:
\(log_{\dfrac{1}{3}}\left(x^2-6x+5\right)+2log^3\left(2-x\right)\ge0\)
\(\Leftrightarrow log_{\dfrac{1}{3}}\left(x^2-6x+5\right)\ge log_{\dfrac{1}{3}}\left(2-x\right)^2\)
\(\Leftrightarrow x^2-6x+5\le\left(2-x\right)^2\)
\(\Leftrightarrow2x-1\ge0\)
Bất phương trình tương đương với:
\(\left\{{}\begin{matrix}x^2-6x+5>0\\2-x>0\\2x-1\ge0\end{matrix}\right.\) \(\Leftrightarrow\left\{{}\begin{matrix}\left[{}\begin{matrix}x< 1\\x>5\end{matrix}\right.\\x< 2\\x\ge\dfrac{1}{2}\end{matrix}\right.\)
\(\Leftrightarrow\dfrac{1}{2}\le x< 1\)
Vậy tập nghiệm của bất phương trình là: \(\left(\dfrac{1}{2};1\right)\)
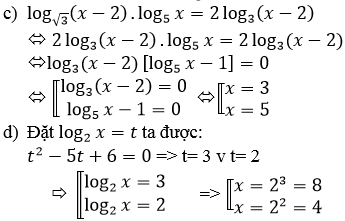
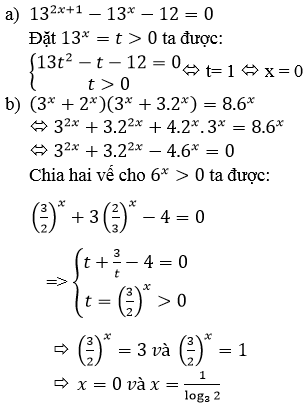
đk: x>0
pt \(\Leftrightarrow\left(1-log_3x\right)log_2x-\left(3log_3x-\frac{1}{2}\right)\)\(=\frac{1}{2}+\frac{1}{2}log_2x\)
\(\Leftrightarrow log_2x-2log_2x.log_3x-6log_3x=0\)
\(\Leftrightarrow log_23log_3x-2log_2x.log_3x-6log_3x=0\)
\(\Leftrightarrow log_3x\left(log_23-2log_2x-6\right)=0\)
\(\Leftrightarrow log_3x\left(log_2x^2-log_2\frac{3}{64}\right)=0\)
\(\Leftrightarrow\orbr{\begin{cases}log_3x=0\\log_2x^2=log_2\frac{3}{64}\end{cases}\Leftrightarrow\orbr{\begin{cases}x=1\\x=\pm\frac{\sqrt{3}}{8}\end{cases}}}\)
Vậy tập nghiệm: S=....