K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
8 tháng 6 2021
Câu 5:
\(y=1-\left(sin2x+cos2x\right)^3\)
\(=1-\left[\sqrt{2}sin\left(2x+\dfrac{\pi}{4}\right)\right]^3\)\(=1-2\sqrt{2}.sin^3\left(2x+\dfrac{\pi}{4}\right)\)
Có \(-1\le sin\left(2x+\dfrac{\pi}{4}\right)\le1\)
\(\Leftrightarrow-1\le sin^3\left(2x+\dfrac{\pi}{4}\right)\le1\) \(\Leftrightarrow1+2\sqrt{2}\ge y\ge1-2\sqrt{2}\)
\(\Rightarrow y_{min}=1-2\sqrt{2}\Leftrightarrow sin\left(2x+\dfrac{\pi}{4}\right)=1\)\(\Leftrightarrow x=\dfrac{\pi}{8}+k\pi\left(k\in Z\right)\)
\(\Rightarrow y_{max}=1+2\sqrt{2}\Leftrightarrow sin\left(2x+\dfrac{\pi}{4}\right)=-1\)\(\Leftrightarrow x=\dfrac{-3\pi}{8}+k\pi\left(k\in Z\right)\)
Ý B
8 tháng 6 2021
Câu 6: Hàm số có TXĐ: D=R
\(y=\sqrt{4-2sin^52x}-8\)
Có \(-1\le sin2x\le1\)
\(\Leftrightarrow-1\le sin^52x\le1\)
\(\Leftrightarrow2\ge-2sin^52x\ge-2\)
\(\Leftrightarrow\)\(\sqrt{6}-8\ge y\ge\sqrt{2}-8\)
Ý A
Câu 7: TXĐ: D=R
\(y=\dfrac{3}{3-\sqrt{1-cosx}}\)
Có \(-1\le cosx\le1\) \(\Leftrightarrow2\ge1-cosx\ge0\) \(\Leftrightarrow3-\sqrt{2}\ge3-\sqrt{1-cosx}\ge3\)
\(\Leftrightarrow\dfrac{3}{3-\sqrt{2}}\le y\le1\)
Vậy \(y_{min}=\dfrac{3}{3-\sqrt{2}}\Leftrightarrow x=\pi+k2\pi\) (k nguyên)
\(y_{max}=1\Leftrightarrow x=k2\pi\) (k nguyên)
PB
0
NV
Nguyễn Việt Lâm
Giáo viên
30 tháng 7 2021
a.
Do \(-1\le sin2x\le1\) nên pt có nghiệm khi:
\(-1\le\dfrac{m+3}{2m-1}\le1\) \(\Leftrightarrow\left\{{}\begin{matrix}\dfrac{m+3}{2m-1}+1\ge0\\\dfrac{m+3}{2m-1}-1\le0\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}\dfrac{3m+2}{2m-1}\ge0\\\dfrac{4-m}{2m-1}\le0\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}m\le-\dfrac{2}{3}\\m\ge4\end{matrix}\right.\)
TT
1
DT
7 tháng 3 2022
Áp dụng công thức \(\left(\dfrac{1}{v}\right)'=\dfrac{-v'}{v^2}\)
Ta có \(y'=\dfrac{-\left(x^2+x-1\right)'}{\left(x^2+x-1\right)^2}=-\dfrac{\left(2x+1\right)}{\left(x^2+x-1\right)^2}\)
HP
7 tháng 9 2021
5.
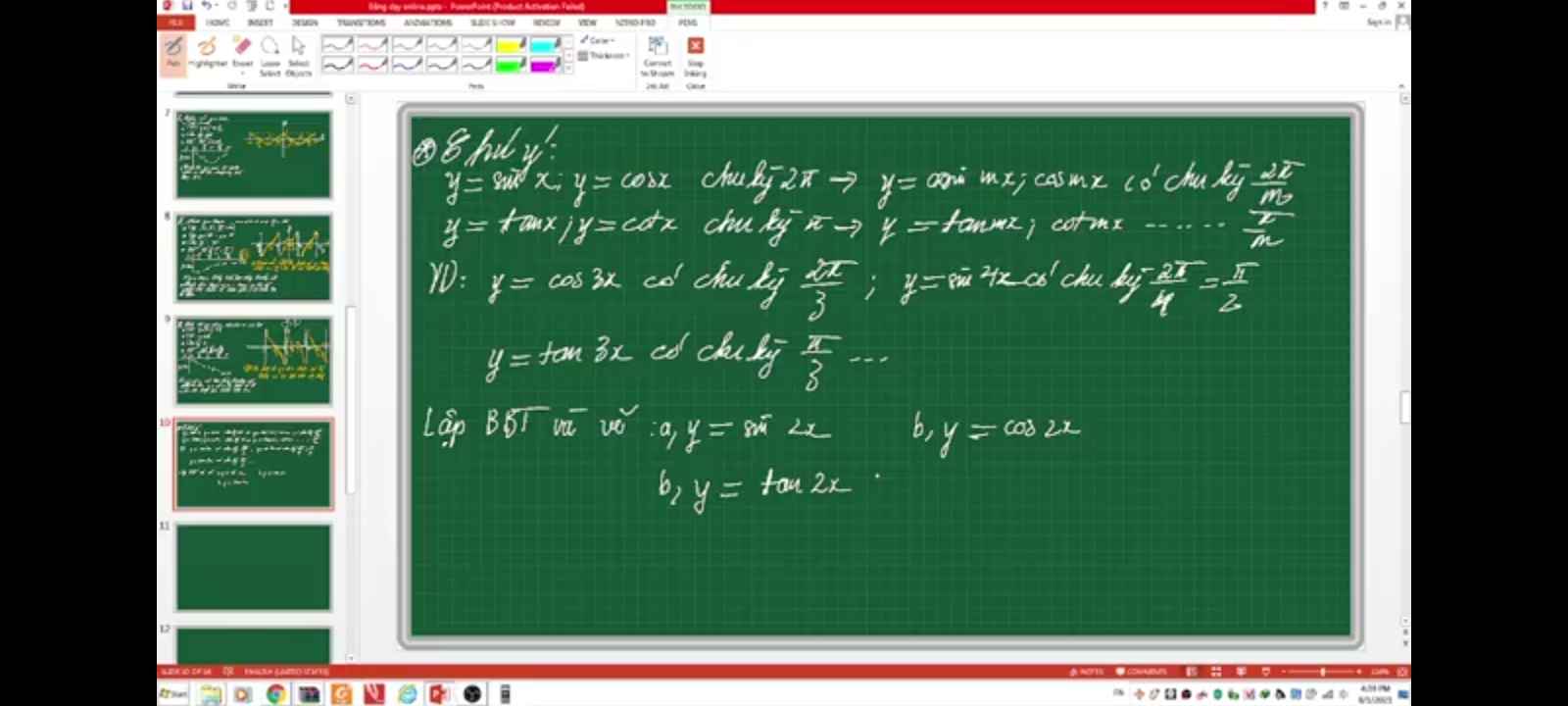
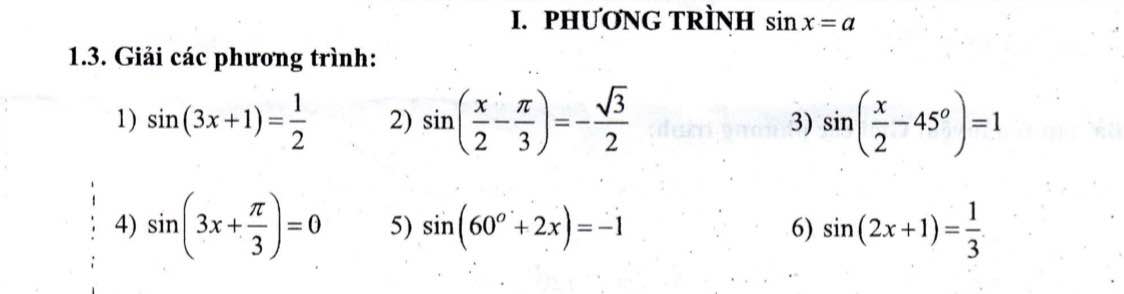
\(sin\left(60^o+2x\right)=-1\)
\(\Leftrightarrow60^o+2x=-90^o+k.360^o\)
\(\Leftrightarrow x=-75^o+k.180^o\)
6.
\(sin\left(2x+1\right)=\dfrac{1}{3}\)
\(\Leftrightarrow\left[{}\begin{matrix}2x+1=arcsin\dfrac{1}{3}+k2\pi\\2x+1=\pi-arcsin\dfrac{1}{3}+k2\pi\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{1}{2}arcsin\dfrac{1}{3}-\dfrac{1}{2}+k\pi\\x=\dfrac{\pi}{2}-\dfrac{1}{2}arcsin\dfrac{1}{3}-\dfrac{1}{2}+k\pi\end{matrix}\right.\)
7 tháng 9 2021
Cách làm :
sina = \(\dfrac{1}{2}\) ⇔ \(\left[{}\begin{matrix}a=\dfrac{\pi}{6}+k2\pi\\a=\dfrac{5\pi}{6}+k2\pi\end{matrix}\right.\)
sina = \(-\dfrac{\sqrt{3}}{2}\) ⇔ \(\left[{}\begin{matrix}a=-\dfrac{\pi}{3}+k2\pi\\a=\dfrac{4\pi}{3}+k2\pi\end{matrix}\right.\)
sina = 1 ⇔ \(a=\dfrac{\pi}{2}+k.2\pi\)
sina = 0 ⇔ \(a=k\pi\)
sina = -1 ⇔ \(a=-\dfrac{\pi}{2}+k.2\pi\)
sina = \(\dfrac{1}{3}\) ⇔ \(\left[{}\begin{matrix}a=arcsin\left(\dfrac{1}{3}\right)+k2\pi\\a=\pi-arcsin\left(\dfrac{1}{3}\right)+k2\pi\end{matrix}\right.\)
Với a là một đa thức xác định trên R
NV
Nguyễn Việt Lâm
Giáo viên
19 tháng 9 2021
\(\Leftrightarrow2cosx+2cos3x=cosx-\sqrt{3}sinx\)
\(\Leftrightarrow\dfrac{1}{2}cosx+\dfrac{\sqrt{3}}{2}sinx=-cos3x\)
\(\Leftrightarrow cos\left(x-\dfrac{\pi}{3}\right)=cos\left(\pi-3x\right)\)
\(\Leftrightarrow...\)
