
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


a) \(\dfrac{A}{x-2}=\dfrac{x^2+3x+2}{x^2-4}\)
\(\Leftrightarrow\dfrac{A}{x-2}=\dfrac{\left(x+2\right)\left(x+1\right)}{\left(x-2\right)\left(x+2\right)}\)
\(\Leftrightarrow\dfrac{A}{x-2}=\dfrac{x+1}{x-2}\Leftrightarrow A=x+1\)
b) \(\dfrac{M}{x-1}=\dfrac{x^2+3x+2}{x+1}\)
\(\Leftrightarrow\dfrac{M}{x-1}=\dfrac{\left(x+1\right)\left(x+2\right)}{x+1}\)
\(\Leftrightarrow\dfrac{M}{x-1}=x+2\Leftrightarrow M=\left(x-1\right)\left(x+2\right)=x^2+x-2\)


Gọi số sản phẩm àm 2 ng công nhân được giao là x (x∈N*, sản phẩm)
Thời gian hoàn thành công việc của người thứ nhất là: \(\dfrac{x}{40}\left(h\right)\)
Thời gian hoàn thành công việc của ngươi thứ hai là: \(\dfrac{x}{50}\left(h\right)\)
Vì ng thứ nhất hoàn thành công việc chậm hơn người thứ hai 2 giờ nên ta có PT:
\(\dfrac{x}{40}-\dfrac{x}{50}=2\)
⇔\(50x-40x=4000\)
⇔\(10x=4000\)
⇔\(x=400\)
Vậy số sản phẩm mỗi công nhân được giao là 400 (sản phẩm)

\(\left(x-1\right)^3+x^3+\left(x+1\right)^3=\left(x+2\right)^3\)
\(\Leftrightarrow x^3-3x^2+3x-1+x^3+x^3+3x^2+3x+1-x^3-6x^2-12x-8=0\)
\(\Leftrightarrow2x^3-6x^2-6x-8=0\)
\(\Leftrightarrow2.\left(x^3-3x^2-3x-4\right)=0\)
\(\Leftrightarrow x^3-4x^2+x^2-4x+x-4=0\)
\(\Leftrightarrow x^2.\left(x-4\right)+x.\left(x-4\right)+\left(x-4\right)=0\)
\(\Leftrightarrow\left(x-4\right).\left(x^2+x+1\right)=0\)
Mà \(x^2+x+1=\left(x+\dfrac{1}{2}\right)^2+\dfrac{3}{4}>0\)
\(\Rightarrow x-4=0\Leftrightarrow x=4\)

Bài 1.2
1: ĐKXĐ: \(\left\{{}\begin{matrix}x\ge0\\x\ne9\end{matrix}\right.\)
2) Ta có: \(A=\dfrac{2\sqrt{x}}{\sqrt{x}+3}-\dfrac{\sqrt{x}+1}{3-\sqrt{x}}-\dfrac{3-11\sqrt{x}}{x-9}\)
\(=\dfrac{2\sqrt{x}\left(\sqrt{x}-3\right)}{\left(\sqrt{x}+3\right)\left(\sqrt{x}-3\right)}+\dfrac{\left(\sqrt{x}+1\right)\left(\sqrt{x}+3\right)}{\left(\sqrt{x}-3\right)\left(\sqrt{x}+3\right)}-\dfrac{3-11\sqrt{x}}{\left(\sqrt{x}+3\right)\left(\sqrt{x}-3\right)}\)
\(=\dfrac{2x-6\sqrt{x}+x+4\sqrt{x}+3-3+11\sqrt{x}}{\left(\sqrt{x}-3\right)\left(\sqrt{x}+3\right)}\)
\(=\dfrac{3x+9\sqrt{x}}{\left(\sqrt{x}-3\right)\left(\sqrt{x}+3\right)}=\dfrac{3\sqrt{x}}{\sqrt{x}-3}\)

a) x(x-y)+(x-y)=(x+1)(x-y)
b) 2x+2y -x(x+y)= 2(x+y)-x(x+y)=(2-x)(x+y)

cách 1: x2 + 5x + 4
= x2 + x + 4x + 4
= (x2 + x) (4x + 4)
= x(x + 1) + 4(x + 1)
= (x + 1) (x + 4)
cách 2: x2 + 5x + 4
= x2 + 5x + 5 - 1
= (x2 - 1) (5x + 5)
= (x + 1) (x - 1) + 5(x + 1)
= (x + 1) (x - 1 + 5)
= (x + 1) (x + 4)
cách 3: x2 + 5x + 4
= x2 + 2x + 3x + 1 + 3
= (x2 + 2x + 1) (3x + 3)
= (x + 1)2 + 3(x + 1)
= (x + 1) (x + 1 + 3)
= (x + 1) (x + 4)
cách 4: x2 + 5x + 4
= x2 + \(2\frac{5}{2}x+\frac{25}{4}-\frac{9}{4}\)
= \(\left(x+\frac{5}{2}\right)^2-\left(\frac{3}{2}\right)^2\)
= \(\left(x+\frac{5}{2}-\frac{3}{2}\right)\left(x+\frac{5}{2}+\frac{3}{2}\right)\)
= (x + 1) (x + 4)
t i c k nhé!!! 4656576765876898745724724724745756765786876876876876

a/
\(\left(x-1\right)^2-\left(x+1\right)^2=2x-6\\ x^2-2x+1-\left(x^2+2x+1\right)=2x-6\\ \)
\(\Leftrightarrow x^2-2x+1-x^2-2x-1-2x+6=0\)
\(\Leftrightarrow6-6x=0\)
=> x=1

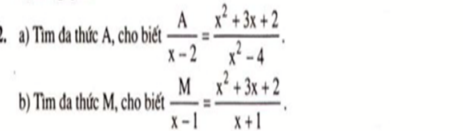 mọi người giải giúp em bài này với ạ em đang cần gấp ạ
mọi người giải giúp em bài này với ạ em đang cần gấp ạ

 em cần giải gấp bài 3 chi tiết mọi người giúp em với ạ. Làm bài dưới dạng phân số ạ em cần gấp
em cần giải gấp bài 3 chi tiết mọi người giúp em với ạ. Làm bài dưới dạng phân số ạ em cần gấp


Câu 3:
Gọi độ dài quãng đường AB là x(km)
Thời gian xe máy đi từ A đến B là: \(\dfrac{x}{30}\left(h\right)\)
Thời gian xe máy đi từ B về A là: \(\dfrac{x}{35}\left(h\right)\)
Theo đề, ta có phương trình: \(\dfrac{x}{30}+\dfrac{x}{35}=\dfrac{13}{3}\)
\(\Leftrightarrow\dfrac{7x}{210}+\dfrac{6x}{210}=\dfrac{910}{210}\)
\(\Leftrightarrow13x=910\)
hay x=70(thỏa ĐK)
Vậy: Độ dài quãng đường AB là 70km