
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


11.
\(=\frac{\sqrt{x}-3}{2-\sqrt{x}}+\frac{\sqrt{x}-2}{3+\sqrt{x}}+\frac{9-x}{(2-\sqrt{x})(\sqrt{x}+3)}\)
\(=\frac{(\sqrt{x}-3)(\sqrt{x}+3)}{(2-\sqrt{x})(\sqrt{x}+3)}+\frac{\sqrt{x}-2}{3+\sqrt{x}}+\frac{9-x}{(2-\sqrt{x})(\sqrt{x}+3)}\)
\(=\frac{x-9}{(2-\sqrt{x})(\sqrt{x}+3)}+\frac{\sqrt{x}-2}{3+\sqrt{x}}+\frac{9-x}{(2-\sqrt{x})(\sqrt{x}+3)}\)
\(=\frac{\sqrt{x}-2}{3+\sqrt{x}}\)
12.
\(=\frac{(3-\sqrt{x})(3\sqrt{x}-2)+(5\sqrt{x}+7)(3\sqrt{x}+4)}{(5\sqrt{x}+7)(3\sqrt{x}-2)}-\frac{42\sqrt{x}+34}{(5\sqrt{x}+7)(3\sqrt{x}-2)}\)
\(=\frac{12x+52\sqrt{x}+22}{(5\sqrt{x}+7)(3\sqrt{x}-2)}-\frac{42\sqrt{x}+34}{(5\sqrt{x}+7)(3\sqrt{x}-2)}\)
\(=\frac{12x+10\sqrt{x}-12}{(5\sqrt{x}+7)(3\sqrt{x}-2)}=\frac{2(3\sqrt{x}-2)(2\sqrt{x}+3)}{(5\sqrt{x}+7)(3\sqrt{x}-2)}=\frac{2(2\sqrt{x}+3)}{5\sqrt{x}+7}\)

`15)(-5sqrtx+4)/(3sqrtx-2)+(6sqrtx+4)/(2sqrtx+3)+(29sqrtx-28)/(3(6x+5sqrtx-6))`
`=(3(-5sqrtx+4)(2sqrtx+3)+3(6sqrtx+4)(3sqrtx-2)+29sqrtx-28)/(3(3sqrtx-2)(2sqrtx+3))`
`=(-30x-21sqrtx+36+54x-24+29sqrtx-28)/(3(3sqrtx-2)(2sqrtx+3))`
`=(24x+8sqrtx-16)/(3(3sqrtx-2)(2sqrtx+3))`
`=(8(3sqrtx-2)(sqrtx+1))/(3(3sqrtx-2)(2sqrtx+3))`
`=(8(sqrtx+1))/(3(2sqrtx+3))`

b) \(\sqrt{25a^2}+3a\) \(=5\left|a\right|+3a\)
Vì a > 0 => |a| = a
=> 5|a| + 3a = 5a + 3a = 8a

Với n > 0 Ta có:
\(\frac{1}{\sqrt{n+1}-\sqrt{n}}=\frac{\sqrt{n+1}+\sqrt{n}}{\left(\sqrt{n+1}-\sqrt{n}\right)\left(\sqrt{n+1}+\sqrt{n}\right)}=\frac{\sqrt{n+1}+\sqrt{n}}{n+1-n}\)
\(=\sqrt{n+1}+\sqrt{n}\)
\(\Rightarrow\frac{1}{\sqrt{16}-\sqrt{15}}-\frac{1}{\sqrt{15}-\sqrt{14}}+...+\frac{1}{\sqrt{10}-\sqrt{9}}\)
\(=\sqrt{16}+\sqrt{15}-\sqrt{15}-\sqrt{14}+...+\sqrt{10}+\sqrt{9}\)
\(\sqrt{16}+\sqrt{9}=3+4=7\)

nếu tính đầy đủ thì = 19 + 1 , 18 + 2 , 17 + 3 , 16 + 4 , 15 + 5 , 14 + 6 , 13 + 7 , 12 + 8 , 11 + 9 = 20 , 20 , 20 , 20 , 20 , 20 , 20 , 20 , 20 20 + 20 + 20 + 20 + 20 + 20 + 20 + 20 + 20 = 40 + 40 + 40 + 40 + 20 = 180 nếu tính tắt thì : 20 x 9 = 180
Ta lấy (1+19)+(2+18)+....(9+11) + 10 + 20
vì 1+19 = 20
mà từ 1 - 19 ( bỏ số 10 ra vì nó chẵn ) có tất cả là 9 cặp
=> 20*9=180 + 10+20 = 210
tk minh nha

Câu 20: A
Câu 16: B
Câu 19: B
Câu 18: A
Câu 4: B
Câu 11; B

cho P = \(\frac{\sqrt{x}+2}{\sqrt{x}+1}\) , Tìm GTLN của P
 Giải giúp câu 10 11 12 13 của bài 15 i
Giải giúp câu 10 11 12 13 của bài 15 i giải giúp em bài 11 12 13 của bài 14 đi ạ
giải giúp em bài 11 12 13 của bài 14 đi ạ Giải giúp câu 15 của bài 14 i
Giải giúp câu 15 của bài 14 i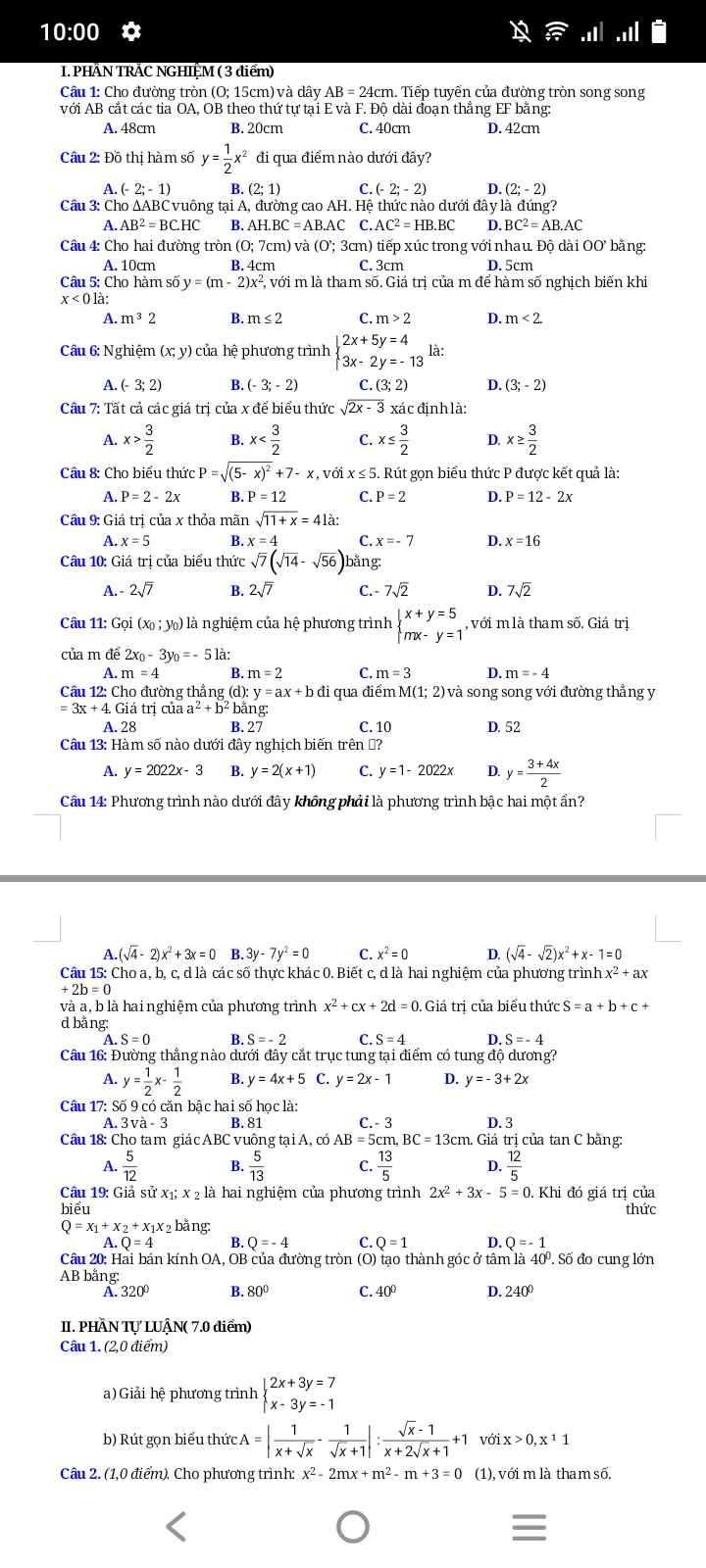
`11)1/(3+sqrt5)+1/(sqrt5-3)=(3-sqrt5)/(9-5)+(sqrt5+3)/(5-9)=(3-sqrt5-3-sqrt5)/4=-sqrt5/2` $\\$ `12)1/(sqrt2-sqrt6)-1/(sqrt6-sqrt2)=(sqrt2+sqrt6)/(2-6)-(sqrt6-sqrt2)/(6-2)=(-sqrt2-sqrt6-sqrt6+sqrt2)/4=-sqrt6/2` $\\$ `13)1/(sqrt2-sqrt3)-3/(sqrt{18}+2sqrt3)=(sqrt2+sqrt3)/(2-3)-(3(sqrt{18}-2sqrt3))/(18-12)=-(sqrt2+sqrt3)-(sqrt{18}-3sqrt2)/2=(-2sqrt2-2sqrt3-3sqrt2+2sqrt3)/2=-(5sqrt2)/2` $\\$ `14)3/(1-sqrt2)+(sqrt2-1)/(sqrt2+1)=(3(1+sqrt2))/(1-2)+(sqrt2-1)^2/(2-1)=-3(1+sqrt2)+3-2sqrt2=-5sqrt2`
Mình đọc không kĩ xin lỗi bạn.
`10)(sqrt5+sqrt6)/(sqrt5-sqrt6)+(sqrt6-sqrt5)/(sqrt6+sqrt5)`
`=(sqrt5+sqrt6)^2/(5-6)+(sqrt6-sqrt5)^2/(6-5)`
`=((sqrt6-sqrt5)^2-(sqrt6+sqrt5)^2)/1`
`=11-2sqrt{30}-11-2sqrt{30}=-4sqrt{30}`