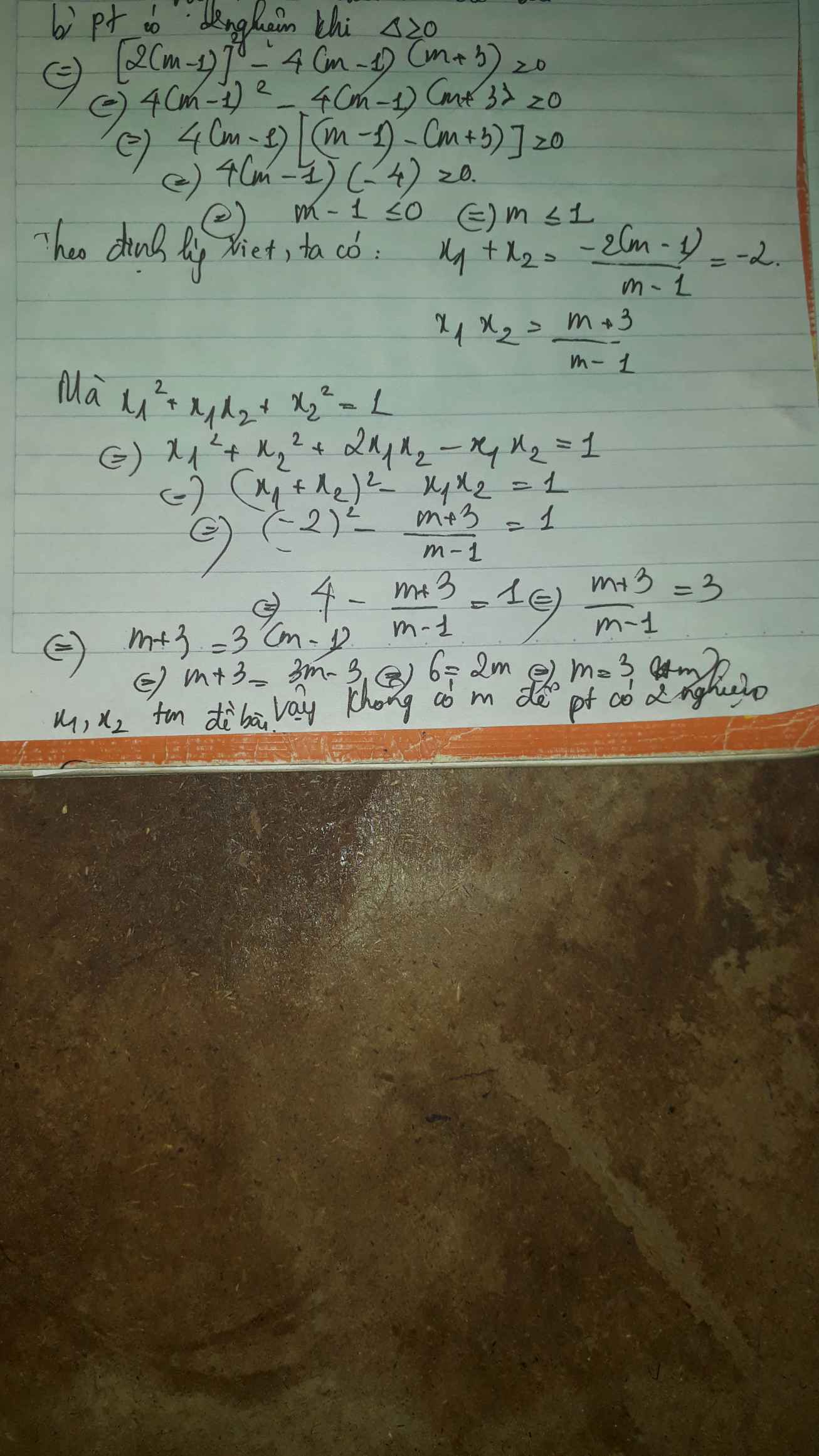Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

a: x1+x2=-2; x1x2=-4
x1+x2+2+2=-2+2+2=2
(x1+2)(x2+2)=x1x2+2(x1+x2)+4
=-4+2*(-2)+4=-4
Phương trình cần tìm là x^2-2x-4=0
b: \(\dfrac{1}{x_1+1}+\dfrac{1}{x_2+1}=\dfrac{x_1+x_2+2}{\left(x_1+1\right)\left(x_2+1\right)}\)
\(=\dfrac{x_1+x_2+2}{x_1x_2+\left(x_1+x_2\right)+1}\)
\(=\dfrac{-2+2}{-4+\left(-2\right)+1}=0\)
\(\dfrac{1}{x_1+1}\cdot\dfrac{1}{x_2+1}=\dfrac{1}{x_1x_2+x_1+x_2+1}=\dfrac{1}{-4-2+1}=\dfrac{-1}{5}\)
Phương trình cần tìm sẽ là; x^2-1/5=0
c: \(\dfrac{x_1}{x_2}+\dfrac{x_2}{x_1}=\dfrac{x_1^2+x_2^2}{x_1x_2}=\dfrac{\left(-2\right)^2-2\cdot\left(-4\right)}{-4}=\dfrac{4+8}{-4}=-3\)
x1/x2*x2/x1=1
Phương trình cần tìm sẽ là:
x^2+3x+1=0

Giả sử x 1 , x 2 là hai nghiệm của phương trình bậc hai a x 2 + bx + c = 0 có ∆’ = 0
Do đó, phương trình có nghiệm kép 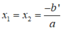
Chọn B

a) Ta có: \(\text{Δ}=\left[-2\left(m-1\right)\right]^2-4\cdot1\cdot\left(-m\right)\)
\(=\left(2m-2\right)^2+4m\)
\(=4m^2-8m+4+4m\)
\(=4m^2-4m+4\)
\(=4m^2-4m+1+3\)
\(=\left(2m-1\right)^2+3>0\forall x\)
Do đó: Phương trình luôn có hai nghiệm x1,x2 với mọi m(Đpcm)
b) Áp dụng hệ thức Vi-et, ta được:
\(\left\{{}\begin{matrix}x_1+x_2=2\left(m-1\right)=2m-2\\x_1\cdot x_2=-m\end{matrix}\right.\)
Ta có: \(y_1+y_2=x_1+\dfrac{1}{x_2}+x_2+\dfrac{1}{x_1}\)
\(=\left(x_1+x_2\right)+\left(\dfrac{1}{x_1}+\dfrac{1}{x_2}\right)\)
\(=\left(2m-2\right)+\dfrac{2m-2}{-m}\)
\(=2m-2-\dfrac{2m-2}{m}\)
\(=\dfrac{2m^2-2m-2m+2}{m}\)
\(=\dfrac{2m^2-4m+2}{m}\)
\(=\dfrac{2\left(m^2-2m+1\right)}{m}\)
\(=\dfrac{2\left(m-1\right)^2}{m}\)
Ta có: \(y_1y_2=\left(x_1+\dfrac{1}{x_2}\right)\left(x_2+\dfrac{1}{x_1}\right)\)
\(=x_1x_2+2+\dfrac{1}{x_1x_2}\)
\(=-m+2+\dfrac{1}{-m}\)
\(=-m+2-\dfrac{1}{m}\)
\(=\dfrac{-m^2}{m}+\dfrac{2m}{m}-\dfrac{1}{m}\)
\(=\dfrac{-m^2+2m-1}{m}\)
\(=\dfrac{-\left(m-1\right)^2}{m}\)
Phương trình đó sẽ là:
\(x^2-\dfrac{2\left(m-1\right)^2}{m}x-\dfrac{\left(m-1\right)^2}{m}=0\)

b) phương trình có 2 nghiệm \(\Leftrightarrow\Delta'\ge0\)
\(\Leftrightarrow\left(m-1\right)^2-\left(m-1\right)\left(m+3\right)\ge0\)
\(\Leftrightarrow m^2-2m+1-m^2-3m+m+3\ge0\)
\(\Leftrightarrow-4m+4\ge0\)
\(\Leftrightarrow m\le1\)
Ta có: \(x_1^2+x_1x_2+x_2^2=1\)
\(\Leftrightarrow\left(x_1+x_2\right)^2-2x_1x_2=1\)
Theo viet: \(\left\{{}\begin{matrix}x_1+x_2=-\dfrac{b}{a}=2\left(m-1\right)\\x_1x_2=\dfrac{c}{a}=m+3\end{matrix}\right.\)
\(\Leftrightarrow\left[-2\left(m-1\right)^2\right]-2\left(m+3\right)=1\)
\(\Leftrightarrow4m^2-8m+4-2m-6-1=0\)
\(\Leftrightarrow4m^2-10m-3=0\)
\(\Leftrightarrow\left[{}\begin{matrix}m_1=\dfrac{5+\sqrt{37}}{4}\left(ktm\right)\\m_2=\dfrac{5-\sqrt{37}}{4}\left(tm\right)\end{matrix}\right.\Rightarrow m=\dfrac{5-\sqrt{37}}{4}\)

x1+x2=1/3; x1x2=-7/3
(x1-1)(x2-1)
=x1x2-(x1+x2)+1
=-7/3-1/3+1
=-8/3+1=-5/3
\(\dfrac{3}{x_1-2}+\dfrac{3}{x_2-2}=\dfrac{3x_2-6+3x_1-6}{\left(x_1-2\right)\left(x_2-2\right)}\)
\(=\dfrac{3\left(x_1+x_2\right)-12}{x_1x_2-2\left(x_1+x_2\right)+4}\)
\(=\dfrac{3\cdot\dfrac{1}{3}-12}{\dfrac{-7}{3}-2\cdot\dfrac{1}{3}+4}=-11\)

Δ=(m+2)^2-4*2m=(m-2)^2
Để PT có hai nghiệm pb thì m-2<>0
=>m<>2
\(\dfrac{1}{x_1}+\dfrac{1}{x_2}=\dfrac{x_1x_2}{4}\)
=>\(\dfrac{x_1+x_2}{x_1x_2}=\dfrac{x_1x_2}{4}\)
=>\(\dfrac{m+2}{2m}=\dfrac{2m}{4}=\dfrac{m}{2}\)
=>2m^2=2m+4
=>m^2-m-2=0
=>m=2(loại) hoặc m=-1

Lời giải:
Theo định lý Viet:
$x_1+x_2=3$
$x_1x_2=-7$
Khi đó:
$A=\frac{1}{x_1-1}+\frac{1}{x_2-1}=\frac{x_2-1+x_1-1}{(x_1-1)(x_2-1)}$
$=\frac{(x_1+x_2)-2}{x_1x_2-(x_1+x_2)+1}=\frac{3-2}{-7-3+1}=\frac{-1}{9}$
$E=x_1^4+x_2^4=(x_1^2+x_2)^2-2(x_1x_2)^2=[(x_1+x_2)^2-2x_1x_2]^2-2(x_1x_2)^2$
$=[3^2-2(-7)]^2-2(-7)^2=431$

Theo vi ét: \(\left\{{}\begin{matrix}x_1+x_2=6\\x_1x_2=8\end{matrix}\right.\)
Theo đề:
\(B=\dfrac{x_1\sqrt{x_1}-x_2\sqrt{x_2}}{x_1-x_2}=\dfrac{\left(\sqrt{x_1}-\sqrt{x_2}\right)\left(x_1+\sqrt{x_1x_2}+x_2\right)}{\left(\sqrt{x_1}-\sqrt{x_2}\right)\left(\sqrt{x_1}+\sqrt{x_2}\right)}\left(x_1,x_2\ge0\right)\)
\(=\dfrac{6+\sqrt{8}}{\sqrt{x_1}+\sqrt{x_2}}\)
Tính: \(\left(\sqrt{x_1}+\sqrt{x_2}\right)^2=x_1+x_2+2\sqrt{x_1x_2}=6+2\sqrt{8}=6+4\sqrt{2}=\left(\sqrt{4}+\sqrt{2}\right)^2\)
\(\Rightarrow\sqrt{x_1}+\sqrt{x_2}=\sqrt{4}+\sqrt{2}\) (thỏa mãn \(x_1,x_2\ge0\))
Khi đó: \(P=\dfrac{6+\sqrt{8}}{\sqrt{4}+\sqrt{2}}=4-\sqrt{2}\)

a. Với m=6 thì phương trình (1) có dạng
x^2 - 5x +4= 0
<=> (x-1)(x-4)=0
<=> x=1 hoặc x=4
Vậy m=6 thì phương trình có nghiệm x=1 hoặc x=4
b. Xét \(\text{ Δ}=\left(-5\right)^2-4\cdot1\cdot\left(m-2\right)=33-4m\)
Để (1) có nghiệm phân biệt khi \(m< \dfrac{33}{4}\)
Theo Vi-et ta có: \(x_1x_2=m-2;x_1+x_2=5\)
Để 2 nghiệm phương trình (1) dương khi m>2
Ta có:
\(\dfrac{1}{\sqrt{x_1}}+\dfrac{1}{\sqrt{x_2}}=\dfrac{3}{2}\Leftrightarrow\dfrac{1}{x_1}+\dfrac{1}{x_2}+\dfrac{2}{\sqrt{x_1x_2}}=\dfrac{9}{4}\\ \Leftrightarrow\dfrac{x_1+x_2}{x_1x_2}+\dfrac{2}{\sqrt{x_1x_2}}=\dfrac{9}{4}\\ \Leftrightarrow\dfrac{5}{m-2}+\dfrac{2}{\sqrt{m-2}}=\dfrac{9}{4}\Leftrightarrow20+8\sqrt{m-2}=9\left(m-2\right)\\ \Leftrightarrow\left(\sqrt{m-2}-2\right)\left(9\sqrt{m-2}+10\right)=0\Leftrightarrow\sqrt{m-2}=2\Leftrightarrow m-2=4\Leftrightarrow m=6\left(t.m\right)\)