Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
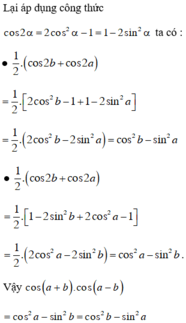
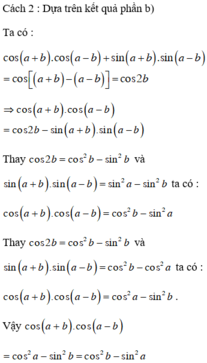
\(sin^6a+cos^6a=\left(sin^2a\right)^3+\left(cos^2a\right)^3\)
\(=\left(sin^2a+cos^2a\right)^3-3sin^2a.cos^2a\left(sin^2a+cos^2a\right)\)
\(=1-3sin^2a.cos^2a\)
a) Ta có: x5 – 1 = (x – 1)(x4 + x3 + x2 + x + 1)
Lại có: x – 1 > 0 ⇒ x > 1 ⇒ x5 > x4 > x3 > x2 > x > 1
⇒ 1 + 1 + 1 + 1 + 1 < x4 + x3 + x2 + x + 1 < x4 + x4 + x4 + x4 + x4
hay 5 < x4 + x3 + x2 + x + 1 < 5x4
⇒ 5.(x – 1) < (x – 1)(x4 + x3 + x2 + x + 1) < 5x4.(x – 1)
hay 5.(x – 1) < x5 – 1 < 5x4.(x – 1) (đpcm)
b) x5 + y5 – x4y – xy4 = (x5 - x4y) - (xy4 - y5)
= x4.(x – y) – y4.(x – y)
= (x4 – y4).(x – y)
= (x2 + y2)(x2 – y2)(x – y)
= (x2 + y2).(x + y)(x – y)(x – y)
= (x2 + y2)(x + y)(x – y)2
Mà x2 + y2 ≥ 0; x + y ≥ 0; (x – y)2 ≥ 0
⇒ x5 + y5 – x4y – xy4 ≥ 0.
c) Ta có: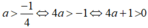 
Tương tự. 4b +1 >0 và 4c +1 > 0
Áp dụng bất đẳng thức Cô-si cho hai số dương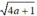 
và 1 ta có:
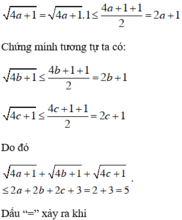
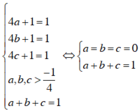
Không có giá trị nào của a, b, c thỏa mãn hệ trên nên dấu “=” của BĐT không xảy ra.
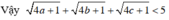
cosα = OH¯; sinα = OK¯
Do tam giác OMK vuông tại K nên:
sin2 α + cos2 α = OK¯2 + OH¯2 = OK2 + MK2 = OM2 = 1.
Vậy sin2 α + cos2 α = 1.
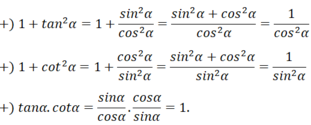
d, \(\dfrac{\left(sinx+cosx\right)^2-1}{2cotx-sin2x}=tan^2x\)
\(\Leftrightarrow\dfrac{sin^2x+cos^2x+2sinx.cosx-1}{2cotx-sin2x}=tan^2x\)
\(\Leftrightarrow2sinx.cosx=tan^2x\left(2cotx-sin2x\right)\)
\(\Leftrightarrow2sinx.cosx=\dfrac{sin^2x}{cos^2x}\left(2\dfrac{cosx}{sinx}-2sinx.cosx\right)\)
\(\Leftrightarrow sinx.cosx=\dfrac{sinx}{cosx}-\dfrac{sin^3x}{cosx}\)
\(\Leftrightarrow sinx.cos^2x=sinx-sin^3x\)
\(\Leftrightarrow sinx.cos^2x=sinx\left(1-sin^2x\right)\)
\(\Leftrightarrow sinx.cos^2x=sinx.cos^2x\)
\(\Rightarrowđpcm\)
a, \(\left(1-sin^2x\right).tan^2x+\left(1-cos^2x\right).cot^2x=1\)
\(\Leftrightarrow cos^2x.\dfrac{sin^2x}{cos^2x}+sin^2x.\dfrac{cos^2x}{sin^2x}=1\)
\(\Leftrightarrow sin^2x+cos^2x=1\)
\(\Rightarrowđpcm\)
b, \(1-sin^2x-sin^2x.cot^2x=0\)
\(\Leftrightarrow cos^2x-cos^2x=0\)
\(\Rightarrowđpcm\)
c, \(cos^4x+sin^2x.cos^2x+sin^2x=1\)
\(\Leftrightarrow\left(cos^2x+sin^2x\right).cos^2x+sin^2x=1\)
\(\Leftrightarrow cos^2x+sin^2x=1\)
\(\Rightarrowđpcm\)
