Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Theo bài ra, ta có: \(x^2-y=y^2-x\Leftrightarrow x^2-y^2=-x+y\)
\(\Leftrightarrow\left(x-y\right)\left(x+y\right)=-\left(x-y\right)\)
\(\Leftrightarrow\left(x+y\right)=-1\)
Ta lại có: \(A=x^2+2xy+y^2-3x-3y=\left(x+y\right)^2-3\left(x+y\right)\)
Thay x+y=-1 vào biểu thức A, ta được: \(A=\left(-1\right)^2-3.\left(-1\right)=1+3=4\)
Vậy A=4

Biến đổi: 4 x 2 − 4 xy + y 2 = 0 ⇔ ( 2 x − y ) 2 = 0 ⇔ 2 x = y
Thay y = 2x vào P ta được P = -3

mk copy trên trang này
https://lazi.vn/edu/exercise/311935/cho-cac-so-thoa-man-2x-3y-13-tim-gia-tri-nho-nhat-cua-q
\(2x+3y=13\Rightarrow y=\dfrac{13-2x}{3}\)
\(Q=x^2+\left(\dfrac{13-2x}{3}\right)^2=\dfrac{13}{9}x^2-\dfrac{52}{9}x+\dfrac{169}{9}\)
\(Q=\dfrac{13}{9}\left(x-2\right)^2+13\ge13\)
\(Q_{min}=13\) khi \(\left\{{}\begin{matrix}x=2\\y=3\end{matrix}\right.\)

a) Ta có: \(M=x^2-2xy+y^2-10x+10y\)
\(=\left(x-y\right)^2-10\left(x-y\right)\)
\(=9^2-10\cdot9=-9\)
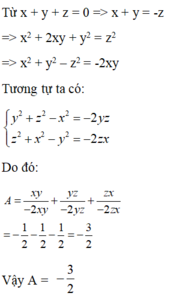
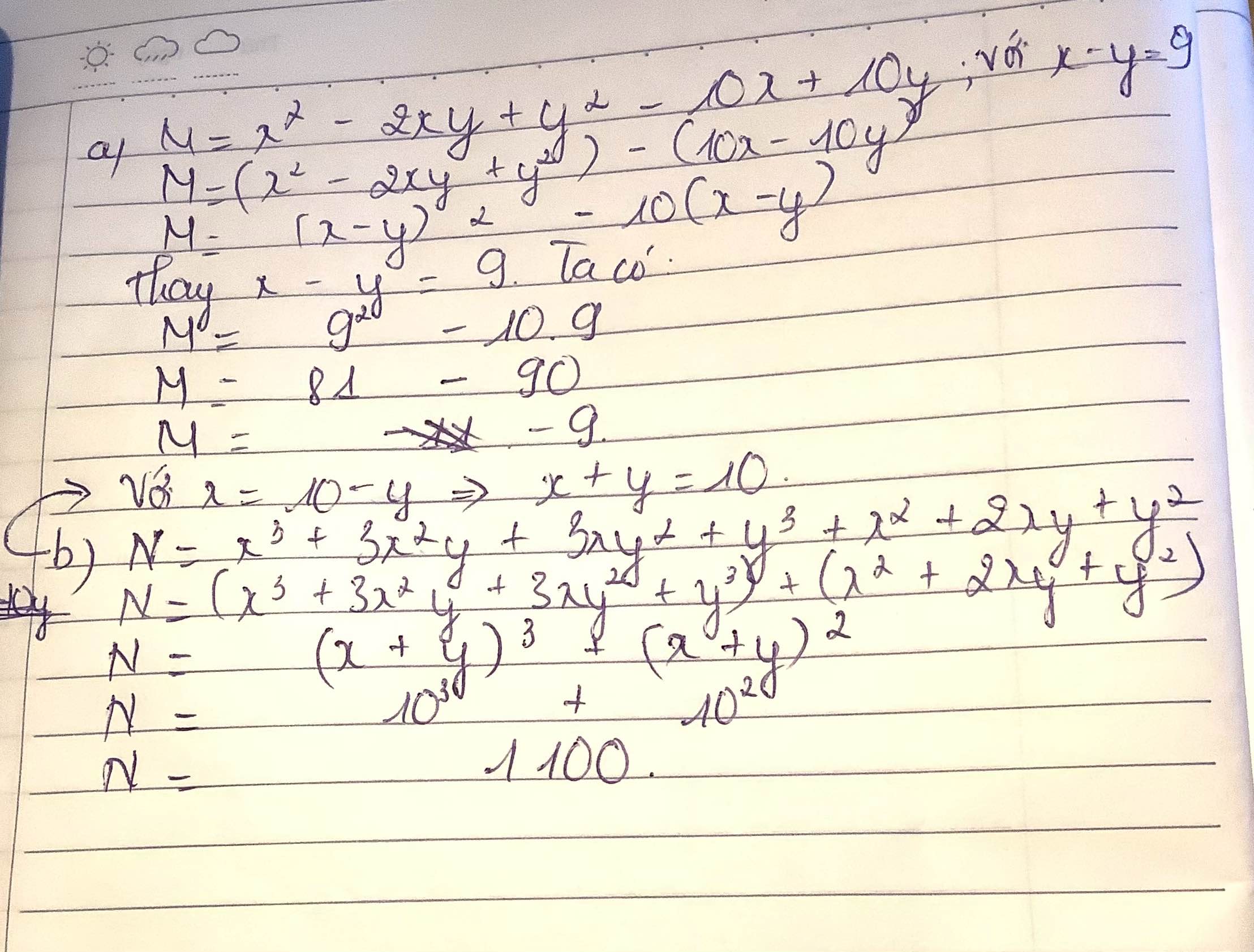

\(x^2-y=y^2-x\)
\(\Rightarrow x^2-y^2+x-y=0\)
\(\Rightarrow\left(x-y\right)\left(x+y\right)+\left(x-y\right)=0\)
\(\Leftrightarrow\left(x-y\right)\left(x+y+1\right)=0\)
Vì \(x\ne y\Rightarrow x-y\ne0\Rightarrow x+y+1=0\)
\(\Rightarrow x+y=-1\)và \(x+y-3=-4\)\(\left(1\right)\)
\(M=x^2+2xy-3x-3y+y^2\)
\(=\left(x+y\right)^2-3\left(x+y\right)\)
\(=\left(x+y\right)\left(x+y-3\right)\)
TThay (1) vào M , ta có :
\(M=\left(-1\right).\left(-4\right)=4\)