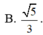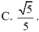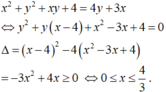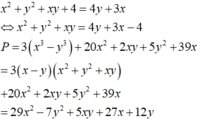Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Một dạng rất uen thuộc của lượng giác là tìm gtnn,ln bằng cách đặt ẩn là sinx và cosx
\(x^2+y^2-2x-4y+4=0\Leftrightarrow\left(x-1\right)^2+\left(y-2\right)^2=1\)
\(\left\{{}\begin{matrix}\sin\alpha=x-1\\\cos\alpha=y-2\end{matrix}\right.\)
\(\Rightarrow\left\{{}\begin{matrix}x=\sin\alpha+1\\y=\cos\alpha+2\end{matrix}\right.\)
\(\Rightarrow P=\left(\sin\alpha+1\right)^2-\left(\cos\alpha+2\right)^2+2\sqrt{3}\left(\sin\alpha+1\right)\left(\cos\alpha+2\right)-2\left(\sin\alpha+1\right)-4\sqrt{3}\left(\sin\alpha+1\right)-4\left(\cos\alpha+2\right)-2\sqrt{3}\left(\cos\alpha+2\right)-3+4\sqrt{3}\)
\(\Leftrightarrow P=\sin^2\alpha-\cos^2\alpha+2\sqrt{3}\sin\alpha\cos\alpha-16\)
Ta đưa về góc 2 alpha để dễ xét
\(\Leftrightarrow P=\frac{1-\cos2\alpha}{2}-\frac{\cos2\alpha+1}{2}+\sqrt{3}\sin2\alpha-16\)
\(\Rightarrow P=\sqrt{3}\sin2\alpha-\cos2\alpha-16\)
\(P=2\sin\left(2\alpha-\frac{\pi}{6}\right)-16\)
\(\Rightarrow2.\left(-1\right)-16\le P\le2.1-16\)
\(\Rightarrow\left\{{}\begin{matrix}P_{min}=-18;"="\Leftrightarrow2\alpha-\frac{\pi}{6}=-\frac{\pi}{2}+k2\pi\\P_{max}=-14;"="\Leftrightarrow2\alpha-\frac{\pi}{6}=\frac{\pi}{2}+k2\pi\end{matrix}\right.\)
Bạn tự thay vô x và y để xét dấu bằng nhé

Đề bài: Giải hệ phương trình:
\(\left\{{}\begin{matrix}y^3-12y-x^3+6x^2-16=0\left(1\right)\\4y^2+2\sqrt{4-y^2}-5\sqrt{4x-x^2}+6=0\left(2\right)\end{matrix}\right.\).
Giải:
ĐKXĐ: \(\left\{{}\begin{matrix}0\le x\le4\\-2\le y\le2\end{matrix}\right.\).
\(\left(1\right)\Leftrightarrow y^3-12y=\left(x-2\right)^3-12\left(x-2\right)\)
\(\Leftrightarrow\left(x-2-y\right)\left[\left(x-2\right)^2+\left(x-2\right)y+y^2-12\right]=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=y+2\\x^2+xy+y^2-4x-2y-8=0\end{matrix}\right.\).
+) TH1: \(x=y+2\): Thay vào (2) ta được:
\(4y^2+2\sqrt{4-y^2}-5\sqrt{4\left(y+2\right)-\left(y+2\right)^2}+6=0\)
\(\Leftrightarrow4y^2+2\sqrt{4-y^2}-5\sqrt{4-y^2}+6=0\)
\(\Leftrightarrow4y^2+6=3\sqrt{4-y^2}\)
\(\Leftrightarrow\left(4y^2+6\right)^2=9\left(4-y^2\right)\)
\(\Leftrightarrow16y^4+57y^2=0\)
\(\Leftrightarrow y=0\Rightarrow x=2\) (TMĐK).
+) TH2: \(x^2+xy+y^2-4x-2y-8=0\):
\(\Leftrightarrow\left(x-2\right)^2+y^2+\left(x-2\right)y=12\).
Do VT \(\le12\) (Đẳng thức xảy ra khi và chỉ khi x = 4; y = 2 hoặc x = 0; y = -2).
Do đó \(\left[{}\begin{matrix}x=4;y=2\\x=0;y=-2\end{matrix}\right.\).
Thử lại không có gt nào thỏa mãn.
Vậy...

1. Áp dụng quy tắc L'Hopital
\(\lim\limits_{x\rightarrow0}\dfrac{\sqrt{x+1}-1}{f\left(0\right)-f\left(x\right)}=\lim\limits_{x\rightarrow0}\dfrac{\dfrac{1}{2\sqrt{x+1}}}{-f'\left(0\right)}=-\dfrac{1}{6}\)
2.
\(g'\left(x\right)=2x.f'\left(\sqrt{x^2+4}\right)=0\Rightarrow\left[{}\begin{matrix}x=0\\f'\left(\sqrt{x^2+4}\right)=0\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=0\\\sqrt{x^2+4}=1\\\sqrt{x^2+4}=-2\end{matrix}\right.\)
2 pt cuối đều vô nghiệm nên \(g'\left(x\right)=0\) có đúng 1 nghiệm

a) √2 cos(x - π/4)
= √2.(cosx.cos π/4 + sinx.sin π/4)
= √2.(√2/2.cosx + √2/2.sinx)
= √2.√2/2.cosx + √2.√2/2.sinx
= cosx + sinx (đpcm)
b) √2.sin(x - π/4)
= √2.(sinx.cos π/4 - sin π/4.cosx )
= √2.(√2/2.sinx - √2/2.cosx )
= √2.√2/2.sinx - √2.√2/2.cosx
= sinx – cosx (đpcm).

Hiển nhiên là cách đầu sai rồi em
Khi đến \(\lim x^2\left(1-1\right)=+\infty.0\) là 1 dạng vô định khác, đâu thể kết luận nó bằng 0 được

ĐKXĐ: ...
Phương trình đầu tương đương:
\(2y^3+y=2\sqrt{1-x}-2x+\sqrt{1-x}\)
\(\Leftrightarrow2y^3+y=2\left(1-x\right)\sqrt{1-x}+\sqrt{1-x}\)
Đặt \(\sqrt{1-x}=a\ge0\)
\(\Rightarrow2y^3+y=2a^3+a\)
Hàm \(f\left(t\right)=2t^3+t\) có \(f'\left(t\right)=6t^2+1>0\) ;\(\forall t\Rightarrow f\left(t\right)\) đồng biến
\(\Rightarrow y=a\Leftrightarrow y=\sqrt{1-x}\Rightarrow y^2=1-x\) (với \(y\ge0\))
Thế xuống pt dưới:
\(\sqrt{4x+5}=2x^2-6x-1\)
Đặt \(\sqrt{4x+5}=2t-3\Rightarrow\left\{{}\begin{matrix}2t-3=2x^2-6x-1\\4x+5=4t^2-12t+9\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}t=x^2-3x+1\\x=t^2-3t+1\end{matrix}\right.\)
Hệ đối xứng, chắc tới đây bạn giải quyết được phần còn lại