Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Gọi G là trọng tâm tam giác ABC
\(x_G=\dfrac{x_A+x_B+x_C}{3}=\dfrac{1}{3};y_G=\dfrac{y_A+y_B+y_C}{3}=\dfrac{1}{3}\)
\(\left|\overrightarrow{MA}+\overrightarrow{MB}+\overrightarrow{MC}\right|=\left|3\overrightarrow{MG}\right|=3MG\)
\(\left|\overrightarrow{MA}+\overrightarrow{MB}+\overrightarrow{MC}\right|\) nhỏ nhất khi \(3MG\) nhỏ nhất
\(\Leftrightarrow M\) là hình chiếu của \(G\) trên trục tung
\(\Leftrightarrow M\left(0;\dfrac{1}{3}\right)\)
\(\Rightarrow\left|\overrightarrow{MA}+\overrightarrow{MB}+\overrightarrow{MC}\right|\le3MG=1\)
Đẳng thức xảy ra khi \(M\left(0;\dfrac{1}{3}\right)\)
\(\Rightarrow\) Tung độ \(y_M=\dfrac{1}{3}\)

* Do đỉnh C thuộc trục Ox nên C(a;0).
G thuộc trục Oy nên G(0; b).
* G là trọng tâm tam giác ABC nên:
x G = x A + x B + x C 3 y G = y A + y B + y C 3 ⇒ 0 = − 2 + 6 + a 3 b = 2 + ( − 4 ) + 0 3 ⇔ a = − 4 b = − 2 3
Tọa độ trọng tâm tam giác ABC là G 0 ; − 2 3
Đáp án B

Tọa độ trọng tâm G x G ; y G là x G = 1 − 2 + 5 3 = 4 3 y G = 3 + 4 + 3 3 = 10 3 .
Chọn D.

38.
Gọi I là trung điểm AB và G là trọng tâm tam giác ABC
\(\Rightarrow\left\{{}\begin{matrix}\overrightarrow{MA}+\overrightarrow{MB}=2\overrightarrow{MI}\\\overrightarrow{GA}+\overrightarrow{GB}+\overrightarrow{GC}=\overrightarrow{0}\end{matrix}\right.\)
\(3\left|\overrightarrow{MA}+\overrightarrow{MB}\right|=2\left|\overrightarrow{MA}+\overrightarrow{MB}+\overrightarrow{MC}\right|\)
\(\Leftrightarrow3.\left|2\overrightarrow{MI}\right|=3\left|\overrightarrow{MG}+\overrightarrow{GA}+\overrightarrow{MG}+\overrightarrow{GB}+\overrightarrow{MG}+\overrightarrow{GC}\right|\)
\(\Leftrightarrow6\left|\overrightarrow{MI}\right|=2.\left|3\overrightarrow{MG}\right|\)
\(\Leftrightarrow6\left|\overrightarrow{MI}\right|=6\left|\overrightarrow{MG}\right|\)
\(\Leftrightarrow\left|\overrightarrow{MI}\right|=\left|\overrightarrow{MG}\right|\)
\(\Leftrightarrow MI=MG\)
\(\Rightarrow\) Tập hợp M là đường trung trực của đoạn thẳng IG

\(\left\{{}\begin{matrix}x_C=3x_G-x_A-x_B=-6\\y_C=3y_G-y_A-y_B=-2\end{matrix}\right.\) \(\Rightarrow C\left(-6;-2\right)\)
Gọi \(M\left(0;m\right)\) \(\Rightarrow\left\{{}\begin{matrix}\overrightarrow{BM}=\left(-1;m-3\right)\\\overrightarrow{CM}=\left(6;m+2\right)\end{matrix}\right.\)
\(\overrightarrow{BM}.\overrightarrow{CM}=0\Leftrightarrow-6+\left(m-3\right)\left(m+2\right)=0\)
\(\Leftrightarrow m^2-m-12=0\Rightarrow\left[{}\begin{matrix}m=-3\\m=4\end{matrix}\right.\)
\(\Rightarrow\left[{}\begin{matrix}M\left(0;-3\right)\\M\left(0;4\right)\end{matrix}\right.\)
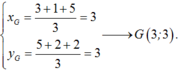



b) Điểm \(M\) thuộc trục tung nên tọa độ điểm \(M\) có dạng \(M\left(0;m\right)\).
\(N\) là trung điểm của \(AB\) suy ra \(N\left(1;4\right)\).
\(\left|\overrightarrow{MA}+\overrightarrow{MB}\right|=\left|2\overrightarrow{MN}\right|=2\sqrt{1^2+\left(m-4\right)^2}\ge2\sqrt{1}=2\)
Dấu \(=\) xảy ra khi \(m-4=0\Leftrightarrow m=4\).
Vậy \(M\left(0;4\right)\).
a) Trọng tâm \(G\) của tam giác \(ABC\):
\(x_G=\dfrac{x_A+x_B+x_C}{3}=\dfrac{4+2-2}{3}=\dfrac{4}{3},y_G=\dfrac{y_A+y_B+y_C}{3}=\dfrac{3-1+5}{3}=\dfrac{7}{3}\).
Vậy \(G\left(\dfrac{4}{3};\dfrac{7}{3}\right)\) là trọng tâm tam giác \(ABC\).