LN
1
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
LN
1
AH
Akai Haruma
Giáo viên
28 tháng 1 2023
Lời giải:
Để pt có 2 nghiệm thì $\Delta=(m-3)^2-4(-m+2)\geq 0$
$\Leftrightarrow (m-1)^2\geq 0$ (luôn đúng với mọi $m\in\mathbb{R}$)
Áp dụng định lý Viet:
$x_1+x_2=m-3$
$x_1x_2=-m+2$
$\Rightarrow x_1+x_2+x_1x_2=-1$
$\Leftrightarrow x_1+x_2+x_1x_2+1=0$
$\Leftrightarrow (x_1+1)(x_2+1)=0$
$\Leftrightarrow x_1=-1$ hoặc $x_2=-1$
TH1: $x_1=-1$
$x_2=\frac{-m+2}{x_1}=m-2$. Khi đó:
$x_1^2+x_2=8$
$\Leftrightarrow (-1)^2+(m-2)=8$
$\Leftrightarrow m=9$
TH2: $x_2=-1$
$x_1=\frac{-m+2}{x_2}=m-2$. Khi đó:
$x_1^2+x_2=8$
$\Leftrightarrow (m-2)^2-1=8$
$\Leftrightarrow (m-2)^2=9$
$\Leftrightarrow m-2=\pm 3\Leftrightarrow m=5$ hoặc $m=-1$
4 tháng 8 2021
\(a,m=3=>x^2+3x-2=0\)
\(\Delta=3^2-4\left(-2\right)=17>0\)
pt có 2 nghiệm pb \(\left[{}\begin{matrix}x1=\dfrac{-3+\sqrt{17}}{2}\\x2=\dfrac{-3-\sqrt{17}}{2}\end{matrix}\right.\)
b,\(\Delta=m^2-4\left(-2\right)=m^2+8>0\)
=> pt đã cho luôn có 2 nghiệm phân biệt x1,x2 với mọi m
theo vi ét \(\left\{{}\begin{matrix}x1+x2=-m\\x1x2=-2\end{matrix}\right.\)
có \(x1^2x2+x2^2x1=2014< =>x1x2\left(x1+x2\right)=2014\)
\(< =>-2\left(-m\right)=2014< =>m=1007\)
4 tháng 8 2021
a) Thay m=3 vào phương trình, ta được:
\(x^2+3x-2=0\)
\(\Delta=3^2-4\cdot1\cdot\left(-2\right)=9+8=17\)
Vì Δ>0 nên phương trình có hai nghiệm phân biệt là:
\(\left\{{}\begin{matrix}x_1=\dfrac{-3-\sqrt{17}}{2}\\x_2=\dfrac{-3+\sqrt{17}}{2}\end{matrix}\right.\)
AH
Akai Haruma
Giáo viên
17 tháng 3 2021
Lời giải:
Để PT có 2 nghiệm $x_1,x_2$ thì:
$\Delta'=(m+1)^2-(m^2-1)>0\Leftrightarrow 2m+2>0\Leftrightarrow m>-1$
Áp dụng định lý Viet:
$x_1+x_2=2(m+1)$ và $x_1x_2=m^2-1$
Khi đó, để $x_1^2+x_2^2=x_1x_2+8$
$\Leftrightarrow (x_1+x_2)^2-2x_1x_2=x_1x_2+8$
$\Leftrightarrow (x_1+x_2)^2=3x_1x_2+8$
$\Leftrightarrow 4(m+1)^2=3(m^2-1)+8$
$\Leftrightarrow m^2+8m-1=0$
$\Leftrightarrow m=-4\pm \sqrt{17}$. Vì $m>-1$ nên $m=-4+\sqrt{17}$
NV
Nguyễn Việt Lâm
Giáo viên
16 tháng 3 2022
\(\Delta'=\left(m-5\right)^2+2m-9=m^2-8m+16=\left(m-4\right)^2\ge0;\forall m\)
Pt đã cho luôn luôn có nghiệm
Theo hệ thức Viet: \(\left\{{}\begin{matrix}x_1+x_2=2\left(m-5\right)\\x_1x_2=-2m+9\end{matrix}\right.\)
Do \(x_1\) là nghiệm của pt nên:
\(x_1^2-2\left(m-5\right)x_1-2m+9=0\Rightarrow x_1^2=2\left(m-5\right)x_1+2m-9\)
Thay vào bài toán:
\(2\left(m-5\right)x_1+2m-9+2\left(m-5\right)x_2=4m^2\)
\(\Leftrightarrow2\left(m-5\right)\left(x_1+x_2\right)+2m-9=4m^2\)
\(\Leftrightarrow2\left(m-5\right).2\left(m-5\right)+2m-9=4m^2\)
\(\Leftrightarrow-38m+91=0\)
\(\Rightarrow m=\dfrac{91}{38}\)
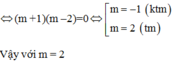
Ta thấy các hệ số \(a,b,c\) của phương trình đã cho thỏa mãn \(a-b+c=1-\left[-\left(m-3\right)\right]-m+2=1+m-3-m+2=0\)
nên phương trình đã cho sẽ có một nghiệm là \(-1\) và nghiệm kia là \(m-2\).
Trong hệ thức \(x_1^2+x_2=8\), vai trò của \(x_1,x_2\) không như nhau nên ta xét 2 trường hợp:
TH1: Nếu \(x_1=-1\) thì \(x_1^2+x_2=8\Leftrightarrow\left(-1\right)^2+m-2=8\Leftrightarrow m=9\).
TH2: Nếu \(x_2=-1\) thì \(x_1^2+x_2=8\Leftrightarrow\left(m-2\right)^2-1=8\Leftrightarrow\left(m-2\right)^2=9\) \(\Leftrightarrow m-2=\pm3\) \(\Leftrightarrow\left[{}\begin{matrix}m=5\\m=-1\end{matrix}\right.\).
Vậy để phương trình đã cho có 2 nghiệm thỏa điều kiện đề cho thì \(\left[{}\begin{matrix}m=-1\\m=5\\m=9\end{matrix}\right.\)