Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

1.
\(cos\left(\widehat{\overrightarrow{u};\overrightarrow{v}}\right)=\dfrac{\overrightarrow{u}.\overrightarrow{v}}{\left|\overrightarrow{u}\right|.\left|\overrightarrow{v}\right|}=\dfrac{10}{10.\sqrt{2}}=\dfrac{1}{\sqrt{2}}\)
\(\Rightarrow\left(\widehat{\overrightarrow{u};\overrightarrow{v}}\right)=45^0\)
2.
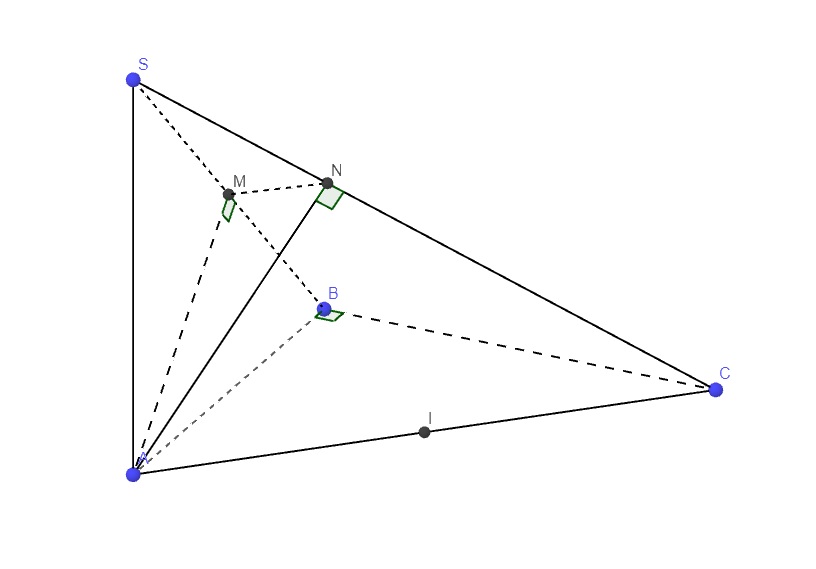
a.
\(\left\{{}\begin{matrix}SA\perp\left(ABC\right)\Rightarrow SA\perp BC\\AB\perp BC\left(gt\right)\end{matrix}\right.\) \(\Rightarrow BC\perp\left(SAB\right)\) (1)
Mà \(BC=\left(SBC\right)\cap\left(ABC\right)\Rightarrow\widehat{SBA}\) là góc giữa (SBC) và (ABC)
\(tan\widehat{SBA}=\dfrac{SA}{AB}=1\Rightarrow\widehat{SBA}=45^0\)
b.
Từ (1) \(\Rightarrow BC\perp AM\)
Mà \(AM\perp SB\left(gt\right)\) \(\Rightarrow AM\perp\left(SBC\right)\) (2)
\(\Rightarrow AM\perp MN\Rightarrow\Delta AMN\) vuông tại M
Từ (2) \(\Rightarrow AM\perp SC\), mà \(SC\perp AN\left(gt\right)\)
\(\Rightarrow SC\perp\left(AMN\right)\) (3)
Lại có \(SA\perp\left(ABC\right)\) theo giả thiết
\(\Rightarrow\) Góc giữa (AMN) và (ABC) bằng góc giữa SA và SC hay là góc \(\widehat{ASC}\)
\(AC=\sqrt{AB^2+BC^2}=a\sqrt{2}\)
\(\Rightarrow tan\widehat{ASC}=\dfrac{AC}{SA}=\sqrt{2}\Rightarrow\widehat{ASC}\approx54^044'\)
Từ (3) \(\Rightarrow AN\) là hình chiếu vuông góc của AC lên (AMN)
\(\Rightarrow\widehat{CAN}\) là góc giữa AC và (AMN)
Mà \(\widehat{CAN}=\widehat{ASC}\) (cùng phụ \(\widehat{ACS}\)) \(\Rightarrow\widehat{CAN}=...\)
c.
\(\left\{{}\begin{matrix}IC=\dfrac{1}{2}AC\left(gt\right)\\AI\cap\left(SBC\right)=C\end{matrix}\right.\) \(\Rightarrow d\left(I;\left(SBC\right)\right)=\dfrac{1}{2}d\left(A;\left(SBC\right)\right)\)
Mà từ (2) ta có \(AM\perp\left(SBC\right)\Rightarrow AM=d\left(A;\left(SBC\right)\right)\)
\(SA=AB\left(gt\right)\Rightarrow\Delta SAB\) vuông cân tại A
\(\Rightarrow AM=\dfrac{1}{2}SB=\dfrac{a\sqrt{2}}{2}\Rightarrow d\left(I;\left(SBC\right)\right)=\dfrac{1}{2}AM=\dfrac{a\sqrt{2}}{4}\)

\(\overrightarrow{BD'}=\overrightarrow{BA}+\overrightarrow{AD}+\overrightarrow{DD'}=-\overrightarrow{AB}+\overrightarrow{AD}+\overrightarrow{AA'}\)
\(=-\overrightarrow{a}+\overrightarrow{b}+\overrightarrow{c}\)

1/ \(\overrightarrow{AB}^2-\overrightarrow{AD}^2=\overrightarrow{BC}^2-\overrightarrow{CD}^2\)
\(\Leftrightarrow\left(\overrightarrow{AB}+\overrightarrow{AD}\right)\left(\overrightarrow{AB}-\overrightarrow{AD}\right)=\left(\overrightarrow{BC}+\overrightarrow{CD}\right)\left(\overrightarrow{BC}-\overrightarrow{CD}\right)\)
\(\Leftrightarrow\left(\overrightarrow{AB}+\overrightarrow{AD}\right).\overrightarrow{DB}=\overrightarrow{BD}\left(\overrightarrow{BC}-\overrightarrow{CD}\right)=\overrightarrow{DB}\left(\overrightarrow{CB}+\overrightarrow{CD}\right)\)
Gọi M là trung điểm BD
\(\Rightarrow2\overrightarrow{AM}.\overrightarrow{DB}=2\overrightarrow{CM}.\overrightarrow{DB}\)
\(\Leftrightarrow\overrightarrow{DB}.\left(\overrightarrow{AM}-\overrightarrow{CM}\right)=0\)
\(\Leftrightarrow\overrightarrow{BD}.\overrightarrow{AC}=0\)
2/ \(A=\left|\overrightarrow{a}-\overrightarrow{b}\right|\Rightarrow A^2=\overrightarrow{a}^2-2\overrightarrow{a}.\overrightarrow{b}+\overrightarrow{b}^2\)
\(=a^2+b^2-2ab.cos\left(\overrightarrow{a};\overrightarrow{b}\right)=4^2+5^2-2.4.5.cos120^0=61\)
\(\Rightarrow A=\sqrt{61}\)
b/ \(B=\left|2\overrightarrow{a}+\overrightarrow{b}\right|\Rightarrow B^2=4a^2+b^2+4\overrightarrow{a}.\overrightarrow{b}\)
\(=4a^2+b^2+4ab.cos120^0=49\)
\(\Rightarrow B=7\)
3/ \(\left|\overrightarrow{x}\right|=\left|\overrightarrow{a}-2\overrightarrow{b}\right|\Rightarrow\left|\overrightarrow{x}\right|^2=a^2+4b^2-4\overrightarrow{a}.\overrightarrow{b}=12\)
\(\Rightarrow\left|\overrightarrow{x}\right|=2\sqrt{3}\)
\(\left|\overrightarrow{y}\right|^2=a^2+b^2-2\overrightarrow{a}.\overrightarrow{b}=5\Rightarrow\left|\overrightarrow{y}\right|=\sqrt{5}\)
\(\overrightarrow{x}.\overrightarrow{y}=\left(\overrightarrow{a}-2\overrightarrow{b}\right)\left(\overrightarrow{a}-\overrightarrow{b}\right)=a^2+2b^2-3\overrightarrow{a}.\overrightarrow{b}=4\)
\(\Rightarrow cos\alpha=\frac{\overrightarrow{x}.\overrightarrow{y}}{\left|\overrightarrow{x}\right|.\left|\overrightarrow{y}\right|}=\frac{4}{2\sqrt{15}}=\frac{2\sqrt{15}}{15}\)

Đáp án B và D giống nhau nên chắc chắn cả 2 đều đúng
Kiểm tra 2 đáp án A và C:
\(\overrightarrow{MN}=\frac{1}{2}\left(\overrightarrow{MC}+\overrightarrow{MD}\right)=\frac{1}{2}\left(\overrightarrow{MA}+\overrightarrow{AC}+\overrightarrow{MB}+\overrightarrow{BD}\right)=\frac{1}{2}\left(\overrightarrow{AC}+\overrightarrow{BD}\right)\)
Vậy đáp án A đúng nên đáp án C sai

\(\overrightarrow{A'C}=\overrightarrow{A'A}+\overrightarrow{AC}=-\overrightarrow{AA'}+\overrightarrow{AB}+\overrightarrow{AD}=\overrightarrow{a}+\overrightarrow{b}-\overrightarrow{c}\)


D
\(\overrightarrow{AC'}+\overrightarrow{CA'}+\overrightarrow{BD'}+\overrightarrow{DB'}\)
\(=2\left(\overrightarrow{OC'}+\overrightarrow{OA'}\right)+2\left(\overrightarrow{OD'}+\overrightarrow{OB'}\right)\)
\(=2.\left(-2\overrightarrow{OI}\right)+2.\left(-2\overrightarrow{OI}\right)\)
\(=-4.2\overrightarrow{OI}\)
\(\Rightarrow2\overrightarrow{OI}=-\dfrac{1}{4}\left(\overrightarrow{u}+\overrightarrow{v}+\overrightarrow{x}+\overrightarrow{y}\right)\)