Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

vậy f(1/2)+3.f(2)=1/4 hay 3f(1/2)+9.f(2)=3/4
và f(2)+3.f(1/2)=4
trừ vế theo vế ta đc
8.f(2)=-13/4
suy ra f(2)=-13/32
mình ko biết xin lỗi bạn nha!
mình ko biết xin lỗi bạn nha!
mình ko biết xin lỗi bạn nha!
mình ko biết xin lỗi bạn nha!
mình ko biết xin lỗi bạn nha!

Đồ thị hàm số đi qua O (0; 0)
Cho x = 2 ⇒ y = 1,5. 2 = 3
Ta có: A(2; 3)
Vẽ đường thẳng OA ta có đồ thị hàm số.
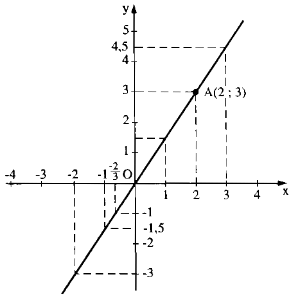
a) f(1) = 1,5. 1 = 1,5
f(-1) = 1,5. (-1) = -1,5
f(-2) = 1,5. (-2) = -3
f(2) = 1,5. 2 = 3
f(0) =0
b)\(y=-1\Rightarrow x=\dfrac{-1}{1,5}=-\dfrac{2}{3}\)
\(y=0\Rightarrow x=\dfrac{0}{1,5}=0\)
\(y=4,5\Rightarrow x=\dfrac{4,5}{1,5}=3\)
c) y > 0 ⇒1,5x > 0 ⇒x > 0
y < 0 ⇒ 1,5x < 0 ⇒ x < 0
Đồ thị hàm số đi qua O (0; 0)
Cho x = 2 ⇒⇒ y = 1,5. 2 = 3
Ta có: A(2; 3)
Vẽ đường thẳng OA ta có đồ thị hàm số.

a) f(1) = 1,5. 1 = 1,5
f(-1) = 1,5. (-1) = -1,5
f(-2) = 1,5. (-2) = -3
f(2) = 1,5. 2 = 3
f(0) = 0
b)y=−1⇒x=\(\dfrac{-1}{1,5}=-\dfrac{2}{3}\)
b)y=0⇒x==\(\dfrac{0}{1,5}=0\)
y=4,5⇒x=\(\dfrac{4,5}{1,5}=3\)
c) y > 0 ⇒1,5x > 0 ⇒x > 0
y < 0 ⇒ 1,5x < 0 ⇒ x < 0

Áp dụng tính chất của dãy tỉ số bằng nhau, ta có:
\(\frac{\left|x-5\right|}{\left|x-3\right|}=\frac{\left|x-1\right|}{\left|x-3\right|}=\frac{\left|x-5\right|-\left|x-1\right|}{\left|x-3\right|-\left|x-3\right|}=\frac{\left|x-5\right|-\left|x-1\right|}{0}\)
Do đó không tồn tại x thỏa mãn.

\(f\left(-1\right)=2\Rightarrow-a+b-c+d=2\\ f\left(0\right)=1\Rightarrow d=1\\ f\left(1\right)=7\Rightarrow a+b+c+d=7\\ f\left(\dfrac{1}{2}\right)=3\Rightarrow\dfrac{1}{8}a+\dfrac{1}{4}b+\dfrac{1}{2}c+d=3\)
\(d=1\Rightarrow-a+b-c=1;a+b+c=6\\ \Rightarrow2b=7\\ \Rightarrow b=\dfrac{7}{2}\\ \Rightarrow\dfrac{1}{8}a+\dfrac{7}{8}+\dfrac{1}{2}c=2\\ \Rightarrow\dfrac{1}{2}\left(\dfrac{1}{4}a+\dfrac{7}{4}+c\right)=2\\ \Rightarrow\dfrac{1}{4}a+\dfrac{7}{4}+c=4\\ \Rightarrow a+7+4c=16\\ \Rightarrow a+4c=9;a+c=6-\dfrac{7}{2}=\dfrac{5}{2}\\ \Rightarrow3c=\dfrac{13}{2}\Rightarrow c=\dfrac{13}{6}\\ \Rightarrow a=\dfrac{5}{2}-\dfrac{13}{6}=\dfrac{1}{3}\)
Vậy \(\left(a;b;c;d\right)=\left(\dfrac{1}{3};\dfrac{7}{2};\dfrac{13}{6};1\right)\)

a) f(-2)=5 – 2. (-2) = 5 + 4 = 9;
f(-1) = 5 – 2.(-1) = 5 + 2 = 7;
f(0) = 5 – 2.0 = 5;
f(3) = 5 – 2.3 = 5 – 6 = -1.
b)\(y=5-2x\Rightarrow x=\dfrac{5y}{2}\)
\(y=5\Rightarrow x=\dfrac{5-5}{2}=0\)
\(y=3\Rightarrow x=\dfrac{5-3}{2}=1\)
\(y=-1\Rightarrow x=\dfrac{5-\left(-1\right)}{2}=\dfrac{5+1}{2}=3\)
Ta có: \(f\left(x\right)=x^2-1\)
\(\Rightarrow f\left(1-x_0\right)=\left(1-x_0\right)^2-1\)
\(=x_0^2-2x_0+1-1=x_0^2-2x_0\)
\(=x_0\left(x_0-2\right)\)
\(f\left(1-x_0\right)< 0\Leftrightarrow\)\(x_0\left(x_0-2\right)< 0\)
Mà \(x_0>x_0-2\)nên \(\hept{\begin{cases}x_0>0\\x_0-2< 0\end{cases}}\Leftrightarrow0< x_0< 2\)
Vậy \(0< x_0< 2\)thì \(f\left(1-x_0\right)\)đạt giá trị âm