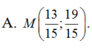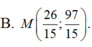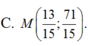Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

\(M\in\left(d\right)\Rightarrow M\left(a;a+6\right)\Rightarrow\left\{{}\begin{matrix}MA=\sqrt{\left(a-2\right)^2+\left(a+4\right)^2}=\sqrt{2\left(a+1\right)^2+18}\\MB=\sqrt{\left(a-3\right)^2+\left(a+6\right)^2}=\sqrt{2\left(a+\dfrac{3}{2}\right)^2+\dfrac{81}{2}}=\sqrt{2\left(-\dfrac{3}{2}-a\right)^2+\dfrac{81}{2}}\end{matrix}\right.\)
\(\Rightarrow MA+MB=\sqrt{\sqrt{2}^2\left(a+1\right)^2+18}+\sqrt{\sqrt{2}^2\left(-\dfrac{3}{2}-a\right)^2+\dfrac{81}{2}}\ge\sqrt{\left(\sqrt{2}.a+\sqrt{2}-\dfrac{3}{2}.\sqrt{2}-\sqrt{2}.a\right)^2+\left(\sqrt{18}+\sqrt{\dfrac{81}{2}}\right)^2}=\sqrt{\dfrac{1}{2}+\dfrac{225}{2}}=\sqrt{133}\)
\(dấu"="xayra\Leftrightarrow\dfrac{\sqrt{2}\left(a+1\right)}{\sqrt{18}}=\dfrac{\sqrt{2}\left(-\dfrac{3}{2}-a\right)}{\sqrt{\dfrac{81}{2}}}\Leftrightarrow a=-\dfrac{6}{5}\Rightarrow M\left(-\dfrac{6}{5};\dfrac{24}{5}\right)\)

M thuộc d nên: \(a-2b-2=0\Rightarrow2b=a-2\)
\(\left\{{}\begin{matrix}\overrightarrow{MA}=\left(-a;1-b\right)\\\overrightarrow{MB}=\left(3-a;4-b\right)\end{matrix}\right.\) \(\Rightarrow\overrightarrow{MA}+\overrightarrow{MB}=\left(3-2a;5-2b\right)=\left(3-2a;9-2a\right)\)
Đặt \(T=\left|\overrightarrow{MA}+\overrightarrow{MB}\right|=\sqrt{\left(3-2a\right)^2+\left(9-2a\right)^2}=\sqrt{8a^2-48a+90}=\sqrt{8\left(a-3\right)^2+18}\ge\sqrt{18}\)
Dấu "=" xảy ra khi \(a-3=0\Leftrightarrow a=3\Rightarrow b=\dfrac{1}{2}\)

Đáp án D
Do M thuộc d nên M( x; 2x+ 3)
Suy ra:
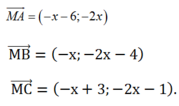
Do đó:
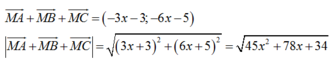
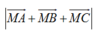
nhỏ nhất khi và chỉ khi: f(x) = 45x2+ 78x + 34 nhỏ nhất
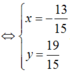


Đường thẳng đi qua hai điểm A và B nhận \(\overrightarrow{AB}=\left(-2;-4\right)\) làm vecto chỉ phương.
Phương trình đường thẳng AB là \(\dfrac{x-1}{-2}=\dfrac{y-3}{-4}\Leftrightarrow2x-y+1=0\)
\(P=MA+MB\) đạt giá trị nhỏ nhất khi M, A, B thẳng hàng
\(\Leftrightarrow M\) là giao điểm của đường thẳng AB và d
\(\Leftrightarrow M\) có tọa độ nghiệm của hệ \(\left\{{}\begin{matrix}x-2y+3=0\\2x-y+1=0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=\dfrac{1}{3}\\y=\dfrac{5}{3}\end{matrix}\right.\)
\(\Rightarrow M\left(\dfrac{1}{3};\dfrac{5}{3}\right)\)

1.
Lấy điểm A' đối xứng với A qua Ox \(\Rightarrow A\left(-2;-1\right)\)
M có tọa độ \(M\left(x;0\right)\)
Ta có \(AM+MB=A'M+MB\ge AB=\sqrt{4^2+5^2}=\sqrt{41}\)
\(min=41\Leftrightarrow M,A',B\) thẳng hàng
\(\Leftrightarrow\overrightarrow{A'M}=k\overrightarrow{A'B}\)
\(\Leftrightarrow\left\{{}\begin{matrix}x+2=k.4\\1=k.5\end{matrix}\right.\Rightarrow x=-\dfrac{6}{5}\Rightarrow M\left(-\dfrac{6}{5};0\right)\)
2.
Gọi N là trung điểm BC
\(\overrightarrow{MA}.\left(\overrightarrow{MB}+\overrightarrow{MC}\right)=0\)
\(\Leftrightarrow2\overrightarrow{MA}.\overrightarrow{MN}=0\)
\(\Leftrightarrow2MA.MN.cosAMN=0\)
\(\Leftrightarrow\left[{}\begin{matrix}MA=0\\MN=0\\cosAMN=0\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}M\equiv A\\M\equiv N\\\widehat{AMN}=90^o\end{matrix}\right.\)
\(\Rightarrow M\) thuộc đường tròn đường kính AN