Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

a. Đề bài em ghi sai thì phải
Vì:
\(x+y=2\left(\sqrt{x-3}+\sqrt{y-3}\right)\)
\(\Leftrightarrow\left(x-3-2\sqrt{x-3}+1\right)+\left(y-3-2\sqrt{y-3}+1\right)+4=0\)
\(\Leftrightarrow\left(\sqrt{x-3}-1\right)^2+\left(\sqrt{y-3}-1\right)^2+4=0\) (vô lý)
b.
Xét hàm \(f\left(x\right)=x^3+ax^2+bx+c\)
Hàm đã cho là hàm đa thức nên liên tục trên mọi khoảng trên R
Hàm bậc 3 nên có tối đa 3 nghiệm
\(f\left(-2\right)=-8+4a-2b+c>0\)
\(f\left(2\right)=8+4a+2b+c< 0\)
\(\Rightarrow f\left(-2\right).f\left(2\right)< 0\Rightarrow f\left(x\right)\) luôn có ít nhất 1 nghiệm thuộc (-2;2)
\(\lim\limits_{x\rightarrow+\infty}f\left(x\right)=x^3\left(1+\dfrac{a}{x}+\dfrac{b}{x^2}+\dfrac{c}{x^3}\right)=+\infty.\left(1+0+0+0\right)=+\infty\)
\(\Rightarrow\) Luôn tồn tại 1 số thực dương n đủ lớn sao cho \(f\left(n\right)>0\)
\(\Rightarrow f\left(2\right).f\left(n\right)< 0\Rightarrow f\left(x\right)\) luôn có ít nhất 1 nghiệm thuộc \(\left(2;n\right)\) hay \(\left(2;+\infty\right)\)
Tương tự \(\lim\limits_{x\rightarrow-\infty}f\left(x\right)=-\infty\Rightarrow f\left(-2\right).f\left(m\right)< 0\Rightarrow f\left(x\right)\) luôn có ít nhất 1 nghiệm thuộc \(\left(-\infty;-2\right)\)
\(\Rightarrow f\left(x\right)\) có đúng 3 nghiệm pb \(\Rightarrow\) hàm cắt Ox tại 3 điểm pb

Đáp án B
P T ⇔ log 2 2 x 2 - x + 2 m - 4 m 2 + log 2 x 2 + m x - 2 m 2 = 0
⇔ 2 x 2 - x + 2 m - 4 m 2 = x 2 + m x - 2 m 2 > 0
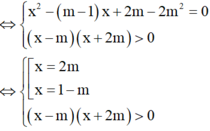
Điều kiện để pt đã cho có 2 nghiệm
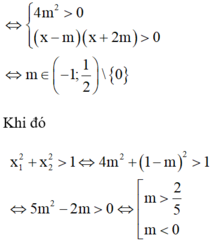
Do đó
S = - 1 ; 0 ∪ 2 5 ; 1 2 ⇒ A = - 1 + 2 + 1 = 2

Cách 1 :
Phương trình a z 2 + bz + c = 0 có Δ = b 2 - 4ac
+ TH1 : Δ < 0, phương trình có hai nghiệm phức 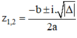
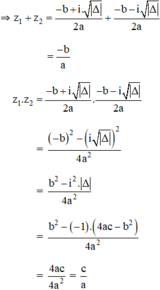
+ TH2: Δ ≥ 0, theo định lý Vi-et ta có:
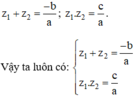
Cách 2 :

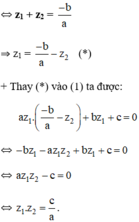

\(\Delta'=m^2-8m+12\)
TH1: \(\Delta'< 0\Rightarrow\) phương trình có 2 nghiệm phức \(z_1;z_2\)
Do \(z_1=m-\sqrt[]{\Delta'};z_2=m+\sqrt{\Delta'}\Rightarrow z_1;z_2\) luôn luôn là 2 số phức liên hợp
\(\Rightarrow\left|z_1\right|=\left|z_2\right|\) luôn đúng khi \(m^2-8m+12< 0\)
\(\Rightarrow2< m< 6\Rightarrow m=\left\{3;4;5\right\}\)
TH2: \(\Delta'=0\Rightarrow m^2-8m+12=0\Rightarrow m=\left\{2;6\right\}\) pt có nghiệm kép (ktm)
TH3: \(\Delta'>0\Rightarrow\left[{}\begin{matrix}m>6\\m< 2\end{matrix}\right.\)
Pt có 2 nghiệm thực phân biệt, để \(\left|z_1\right|=\left|z_2\right|\Rightarrow\left[{}\begin{matrix}z_1=z_2\left(loại\right)\\z_1=-z_2\end{matrix}\right.\)
\(\Rightarrow z_1+z_2=0\Rightarrow2m=0\Rightarrow m=0\)
Vậy \(m=\left\{0;3;4;5\right\}\) có 4 giá trị nguyên của m







Đáp án B