Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

1, Với x >= 0 ; x khác 1
\(P=\dfrac{\sqrt{x}\left(x-1\right)+2\sqrt{x}\left(\sqrt{x}-1\right)-\left(3x+1\right)\left(\sqrt{x}+1\right)}{\left(x-1\right)\left(\sqrt{x}+1\right)}\)
\(=\dfrac{x\sqrt{x}+2x-3\sqrt{x}-3x\sqrt{x}-3x-\sqrt{x}-1}{\left(x-1\right)\left(\sqrt{x}+1\right)}\)
\(=\dfrac{-2x\sqrt{x}-x-4\sqrt{x}-1}{\left(x-1\right)\left(\sqrt{x}+1\right)}\)
mình sửa đề câu 2 nhé
a, \(x^2+mx-1=0\)
\(\Delta=m^2-4\left(-1\right)=m^2+4>0\)
Vậy pt luôn có 2 nghiệm pb
b, Theo Vi et : \(\left\{{}\begin{matrix}x_1+x_2=-m\\x_1x_2=-1\end{matrix}\right.\)
Ta có : \(\left(x_1+x_2\right)^2-2x_1x_2=7\)
Thay vào ta được : \(m^2+2=7\Leftrightarrow m^2=5\Leftrightarrow m=\pm\sqrt{5}\)

a; \(A=\left(\dfrac{1}{x-1}-\dfrac{2x}{\left(x^2+1\right)\left(x-1\right)}\right):\left(1-\dfrac{2x}{x^2+1}\right)\)
\(=\dfrac{x^2-2x+1}{\left(x-1\right)\left(x^2+1\right)}:\dfrac{x^2+1-2x}{x^2+1}=\dfrac{1}{x-1}\)
b: Để A<0 thì x-1<0
hay x<1
c: Để A nguyên thì \(x-1\in\left\{1;-1\right\}\)
hay \(x\in\left\{2;0\right\}\)

a: Thay x=2 vào B, ta được:
\(B=\dfrac{2}{\sqrt{2}-1}=2\sqrt{2}+2\)
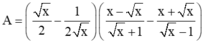
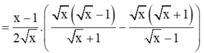
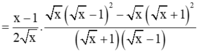
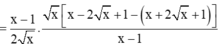
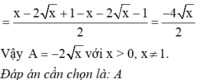
a ) Rút gọn biểu thức :
\(P=x\left(\dfrac{x+1}{x^2+x+1}+\dfrac{1}{1-x}+\dfrac{x^2+2}{x^3-1}\right)\)
\(=\dfrac{x^2-1-x^2-x-1+x^2+2}{x^3-1}\)
\(=\dfrac{x^2-x}{\left(x-1\right)\left(x^2+x+1\right)}=\dfrac{x\left(x-1\right)}{\left(x-1\right)\left(x^2+x+1\right)}=\dfrac{x}{x^2+x+1}\) ( 1 )
b ) Tìm x để P = 7 .
Thay P = 7 vào biểu thức ( 1 ) ta có :
\(\dfrac{x}{x^2+x+1}=7\)
\(\Leftrightarrow x=7\left(x^2+x+1\right)\)
\(\Leftrightarrow\)\(7\left(x^2+1\right)=0\)
Vì \(x^2\ge0\) nên suy ra \(x^2+1\ge1\)
Vậy không có x thỏa mãn để P = 7 .