Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Đáp án C.
Ta có:
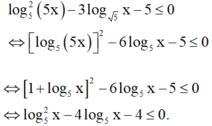
Đặt t = log5 x thì bất phương trình trở thành t 2 - 4 t - 4 ≤ 0 .

Tính \(I=\int_0^{\dfrac{\pi}{2}}\dfrac{cos^{2017}x}{sin^{2017}x+cos^{2017}}dx\left(1\right)\)
Đặt \(t=cosx\Rightarrow sinx=\sqrt{1-cos^2x}\)
\(\Rightarrow dt=-sinx.dx\)
\(\Rightarrow I=\int_0^1\dfrac{t^{2017}.}{\sqrt{1-t^2}.\left(\left(\sqrt{1-t^2}\right)^{2017}+t^{2017}\right)}dt\)
Đặt: \(t=siny\Rightarrow\sqrt{1-t^2}=cosy\)
\(\Rightarrow dt=cosy.dy\)
\(\Rightarrow I=\int_0^{\dfrac{\pi}{2}}\dfrac{sin^{2017}y.cosy}{cosy\left(cos^{2017}y+sin^{2017}y\right)}dy=\int_0^{\dfrac{\pi}{2}}\dfrac{sin^{2017}y}{\left(cos^{2017}y+sin^{2017}y\right)}\)
\(\Rightarrow I=\int_0^{\dfrac{\pi}{2}}\dfrac{sin^{2017}x}{\left(cos^{2017}x+sin^{2017}x\right)}\left(2\right)\)
Cộng (1) và (2) ta được
\(2I=\int_0^{\dfrac{\pi}{2}}\dfrac{sin^{2017}x+cos^{2017}x}{sin^{2017}x+cos^{2017}x}dx=\int_0^{\dfrac{\pi}{2}}1dx\)
\(=x|^{\dfrac{\pi}{2}}_0=\dfrac{\pi}{2}\)
\(\Rightarrow I=\dfrac{\pi}{4}\)
Thế lại bài toán ta được
\(\dfrac{\pi}{4}+t^2-6t+9-\dfrac{\pi}{4}=0\)
\(\Leftrightarrow t^2-6t+9=0\)
\(\Leftrightarrow t=3\)
Chọn đáp án C
mỗi trắc nghiệm thoy mà lm dài ntn s @@
chắc lên đó khó lắm ag




Đáp án C