Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

bn tham khảo nha
https://hoc24.vn/cau-hoi/cho-ba-so-thuc-abc-duong-chung-minh-rangsqrtdfraca3a3leftbcright3sqrtdfracb3b3leftcaright3sqrtdfracc3c.5222680437292

a.
\(\dfrac{x}{x+\sqrt{3x+yz}}=\dfrac{x}{x+\sqrt{x\left(x+y+z\right)+yz}}=\dfrac{x}{x+\sqrt{\left(x+y\right)\left(z+x\right)}}\le\dfrac{x}{x+\sqrt{\left(\sqrt{xz}+\sqrt{xy}\right)^2}}\)
\(\Rightarrow\dfrac{x}{x+\sqrt{3x+yz}}\le\dfrac{x}{x+\sqrt{xy}+\sqrt{xz}}=\dfrac{\sqrt{x}}{\sqrt{x}+\sqrt{y}+\sqrt{z}}\)
Tương tự:
\(\dfrac{y}{y+\sqrt{3y+xz}}\le\dfrac{\sqrt{y}}{\sqrt{x}+\sqrt{y}+\sqrt{z}}\) ; \(\dfrac{z}{z+\sqrt{3z+xy}}\le\dfrac{\sqrt{z}}{\sqrt{x}+\sqrt{y}+\sqrt{z}}\)
Cộng vế:
\(VT\le\dfrac{\sqrt{x}}{\sqrt{x}+\sqrt{y}+\sqrt{z}}+\dfrac{\sqrt{y}}{\sqrt{x}+\sqrt{y}+\sqrt{z}}+\dfrac{\sqrt{z}}{\sqrt{x}+\sqrt{y}+\sqrt{z}}=1\) (đpcm)
Dấu "=" xảy ra khi \(x=y=z=1\)
b.
\(VP=\dfrac{4\left(a+b+c\right)}{2\sqrt{4a\left(a+3b\right)}+2\sqrt{4b\left(b+3c\right)}+2\sqrt{4c\left(c+3a\right)}}\)
\(VP\ge\dfrac{4\left(a+b+c\right)}{4a+a+3b+4b+b+3c+4c+c+3a}\)
\(VP\ge\dfrac{4\left(a+b+c\right)}{8\left(a+b+c\right)}=\dfrac{1}{2}\) (đpcm)
Dấu "=" xảy ra khi \(a=b=c\)
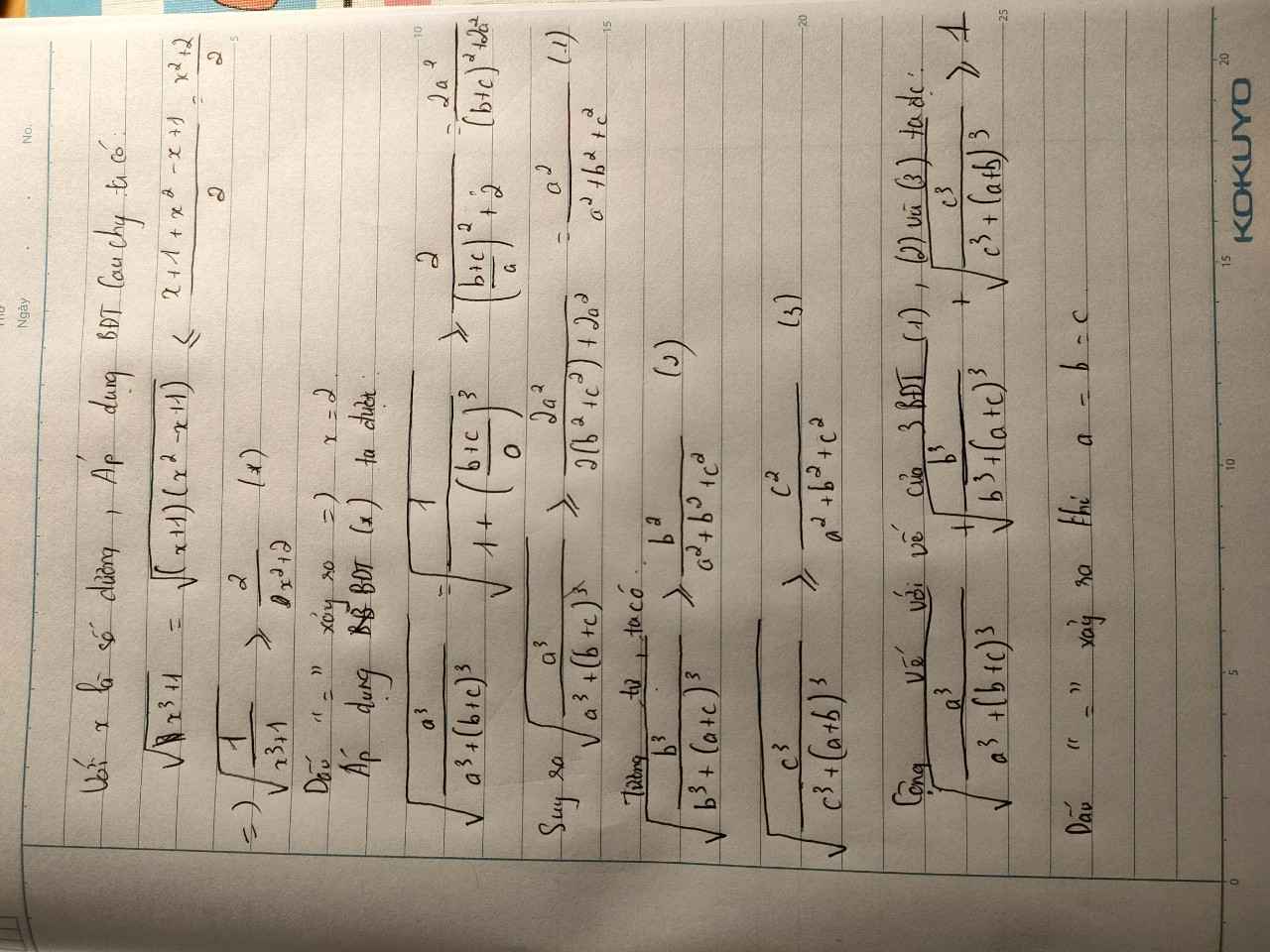

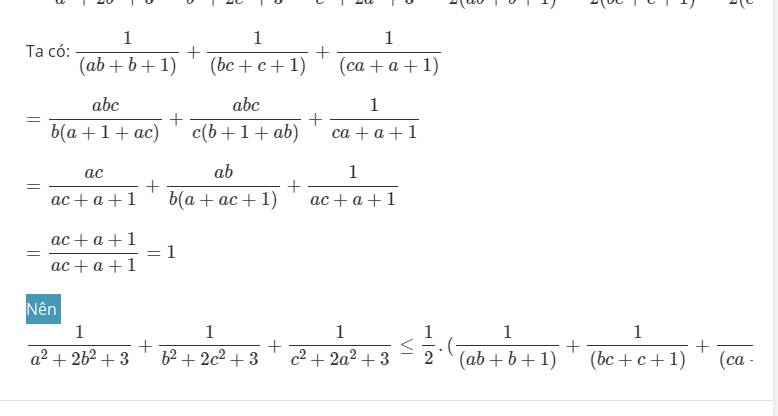


\(\Sigma\sqrt{\dfrac{a^3}{a^3+\left(b+c\right)^3}}=\Sigma\sqrt{\dfrac{1}{1+\left(\dfrac{b+c}{a}\right)^3}}\)\(\left(1\right)\)
\(đặt:\left(\left(\dfrac{b+c}{a}\right)^{ };\left(\dfrac{c+a}{b}\right)^{ };\left(\dfrac{a+b}{c}\right)^{ }\right)=\left(x;y;z\right)\)
\(\left(1\right)\Leftrightarrow\sqrt{\dfrac{1}{1+x^3}}+\sqrt{\dfrac{1}{1+y^3}}+\sqrt{\dfrac{1}{1+z^3}}=\sqrt{\dfrac{1}{\left(x+1\right)\left(x^2-x+1\right)}}+\sqrt{\dfrac{1}{\left(y+1\right)\left(y^2-y+1\right)}}+\sqrt{\left(z+1\right)\left(z^2-z+1\right)}\)
\(\sqrt{\dfrac{1}{\left(x+1\right)\left(x^2-x+1\right)}}\ge\dfrac{1}{\dfrac{x+1+x^2-x+1}{2}}=\dfrac{2}{x^2+2}\)
\(tương\) \(tự\Rightarrow\left(1\right)\ge\dfrac{2}{x^2+2}+\dfrac{2}{y^2+2}+\dfrac{2}{z^2+2}\)
\(=\dfrac{2}{\left(\dfrac{b+c}{a}\right)^2+2}+\dfrac{2}{\left(\dfrac{c+a}{b}\right)^2+2}+\dfrac{2}{\left(\dfrac{a+b}{c}\right)^2+2}=\dfrac{2a^2}{\left(b+c\right)^2+2a^2}+\dfrac{2b^2}{\left(c+a\right)^2+2b^2}+\dfrac{2c^2}{\left(a+b\right)^2+2c^2}\)
\(bunhia\Rightarrow\left(b+c\right)^2\le2\left(b^2+c^2\right)\Rightarrow\dfrac{2a^2}{\left(b+c\right)^2+2a^2}\ge\dfrac{2a^2}{2\left(a^2+b^2\right)+2a^2}=\dfrac{a^2}{a^2+b^2+c^2}\)
\(tương\) \(tự\Rightarrow\left(1\right)\ge\dfrac{a^2+b^2+c^2}{a^2+b^2+c^2}=1\left(đpcm\right)\)