Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

đk x > 0
\(\dfrac{A}{B}=\dfrac{\dfrac{x+2\sqrt{x}}{x}}{\dfrac{\sqrt{x}+2}{\sqrt{x}+1}}=\dfrac{\dfrac{\sqrt{x}+2}{\sqrt{x}}}{\dfrac{\sqrt{x}+2}{\sqrt{x}+1}}=\dfrac{\sqrt{x}+1}{\sqrt{x}}-\dfrac{7}{4}< 0\)
\(\Leftrightarrow\dfrac{4\sqrt{x}+4-7\sqrt{x}}{4\sqrt{x}}< 0\Leftrightarrow\dfrac{-3\sqrt{x}+4}{4\sqrt{x}}< 0\)
\(\Leftrightarrow\left\{{}\begin{matrix}-3\sqrt{x}+4\ne0\\-3\sqrt{x}+4< 0\\4\sqrt{x}\ne0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x\ne\dfrac{16}{9}\\x< \dfrac{16}{9}\\x\ne0\end{matrix}\right.\)

đk x >= 0 ; x khác 1/4
Ta có \(^{P=\dfrac{5\sqrt{x}}{2\sqrt{x}+1}+\dfrac{1}{2\sqrt{x}+1}}=\dfrac{5\sqrt{x}+1}{2\sqrt{x}+1}\)
\(\Rightarrow5\sqrt{x}+1⋮2\sqrt{x}+1\Leftrightarrow10\sqrt{x}+2⋮2\sqrt{x}+1\)
\(\Leftrightarrow5\left(2\sqrt{x}+1\right)-3⋮2\sqrt{x}+1\Rightarrow2\sqrt{x}+1\inƯ\left(-3\right)=\left\{\pm1;\pm3\right\}\)
| \(2\sqrt{x}+1\) | 1 | -1 | 3 | -3 |
| x | 0 | loại | 1 | loại |

Lời giải:
$5A+B=\frac{5\sqrt{x}+1}{2\sqrt{x}+1}$
$2(5A+B)=\frac{10\sqrt{x}+2}{2\sqrt{x}+1}=\frac{5(2\sqrt{x}+1)-3}{2\sqrt{x}+1}=5-\frac{3}{2\sqrt{x}+1}$
$5A+B$ nguyên
$\Rightarrow 2(5A+B)$ nguyên
$\Leftrightarrow 5-\frac{3}{2\sqrt{x}+1}$ nguyên
$\Leftrightarrow \frac{3}{2\sqrt{x}+1}$ nguyên
Ta thấy: $\frac{3}{2\sqrt{x}+1}\leq 3$ với mọi $x\geq 0$ và $\frac{3}{2\sqrt{x}+1}>0$ với mọi $x\geq 0$
Do đó $\frac{3}{2\sqrt{x}+1}$ nguyên thì nhận các giá trị $1,2,3$
$\Leftrightarrow x=0; \frac{1}{16}; 1$

Ta có: \(A=\left(\dfrac{x\sqrt{x}-1}{x-\sqrt{x}}-\dfrac{x\sqrt{x}+1}{x+\sqrt{x}}\right):\left(\dfrac{2\left(x-2\sqrt{x}+1\right)}{x-1}\right)\)
\(=\dfrac{x+\sqrt{x}+1-x+\sqrt{x}-1}{\sqrt{x}}:\dfrac{2\left(\sqrt{x}-1\right)}{\sqrt{x}+1}\)
\(=\dfrac{\sqrt{x}+1}{\sqrt{x}-1}\)
Để A nguyên thì \(\sqrt{x}-1\in\left\{-1;1;2\right\}\)
\(\Leftrightarrow\sqrt{x}\in\left\{0;2;3\right\}\)
hay \(x\in\left\{0;4;9\right\}\)

ĐKXĐ: \(x>0;x\ne9\)
\(P=\left(\dfrac{x+7}{\left(\sqrt{x}+1\right)\left(\sqrt{x}-3\right)}-\dfrac{4\left(\sqrt{x}+1\right)}{\left(\sqrt{x}+1\right)\left(\sqrt{x}-3\right)}+\dfrac{\sqrt{x}-3}{\left(\sqrt{x}+1\right)\left(\sqrt{x}-3\right)}\right)\left(\dfrac{\sqrt{x}+6}{\sqrt{x}}\right)\)
\(=\left(\dfrac{x+7-4\sqrt{x}-4+\sqrt{x}-3}{\left(\sqrt{x}+1\right)\left(\sqrt{x}-3\right)}\right)\left(\dfrac{\sqrt{x}+6}{\sqrt{x}}\right)\)
\(=\left(\dfrac{x-3\sqrt{x}}{\left(\sqrt{x}+1\right)\left(\sqrt{x}-3\right)}\right).\left(\dfrac{\sqrt{x}+6}{\sqrt{x}}\right)\)
\(=\dfrac{\sqrt{x}\left(\sqrt{x}-3\right)}{\left(\sqrt{x}+1\right)\left(\sqrt{x}-3\right)}.\dfrac{\left(\sqrt{x}+6\right)}{\sqrt{x}}\)
\(=\dfrac{\sqrt{x}+6}{\sqrt{x}+1}\)
b.
Ta có \(P=\dfrac{\sqrt{x}+1+5}{\sqrt{x}+1}=1+\dfrac{5}{\sqrt{x}+1}\)
Do \(\sqrt{x}+1>0\Rightarrow\dfrac{5}{\sqrt{x}+1}>0\Rightarrow P>1\)
\(P=\dfrac{6\left(\sqrt{x}+1\right)-5\sqrt{x}}{\sqrt{x}+1}=6-\dfrac{5\sqrt{x}}{\sqrt{x}+1}\)
Do \(\left\{{}\begin{matrix}5\sqrt{x}>0\\\sqrt{x}+1>0\end{matrix}\right.\) ;\(\forall x>0\Rightarrow\dfrac{5\sqrt{x}}{\sqrt{x}+1}>0\)
\(\Rightarrow P< 6\Rightarrow1< P< 6\)
Mà P nguyên \(\Rightarrow P=\left\{2;3;4;5\right\}\)
- Để \(P=2\Rightarrow\dfrac{\sqrt{x}+6}{\sqrt{x}+1}=2\Rightarrow\sqrt{x}+6=2\sqrt{x}+2\Rightarrow x=16\)
- Để \(P=3\Rightarrow\dfrac{\sqrt{x}+6}{\sqrt{x}+1}=3\Rightarrow\sqrt{x}+6=3\sqrt{x}+3\Rightarrow\sqrt{x}=\dfrac{3}{2}\Rightarrow x=\dfrac{9}{4}\)
- Để \(P=4\Rightarrow\dfrac{\sqrt{x}+6}{\sqrt{x}+1}=4\Rightarrow\sqrt{x}+6=4\sqrt{x}+4\Rightarrow\sqrt{x}=\dfrac{2}{3}\Rightarrow x=\dfrac{4}{9}\)
- Để \(P=5\Rightarrow\dfrac{\sqrt{x}+6}{\sqrt{x}+1}=5\Rightarrow\sqrt{x}+6=5\sqrt{x}+5\Rightarrow\sqrt{x}=\dfrac{1}{4}\Rightarrow x=\dfrac{1}{16}\)

a. \(N=\left(\dfrac{x+2}{x\sqrt{x}+1}-\dfrac{1}{\sqrt{x}+1}\right).\dfrac{4\sqrt{x}}{3}\) \(\left(ĐKXĐ:x\ge0\right)\)
\(N=\left(\dfrac{x+2}{x\sqrt{x}+1}-\dfrac{x-\sqrt{x}+1}{x\sqrt{x}+1}\right).\dfrac{4\sqrt{x}}{3}\)
\(\text{}\text{}N=\dfrac{\sqrt{x}+1}{x\sqrt{x}+1}.\dfrac{4\sqrt{x}}{3}\)
\(N=\dfrac{4\sqrt{x}}{3\left(x-\sqrt{x}+1\right)}\)
b.\(N=\dfrac{8}{9}\Leftrightarrow\dfrac{4\sqrt{x}}{3\left(x-\sqrt{x}+1\right)}=\dfrac{8}{9}\)
\(\Leftrightarrow3\sqrt{x}=2x-2\sqrt{x}+2\)
\(\Leftrightarrow\left(2\sqrt{x}-1\right)\left(\sqrt{x}-2\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{1}{4}\\x=4\end{matrix}\right.\)
c.\(\dfrac{1}{N}>\dfrac{3\sqrt{x}}{4}\Leftrightarrow\dfrac{3\left(x-\sqrt{x}+1\right)}{4\sqrt{x}}>\dfrac{3\sqrt{x}}{4}\)
\(\Leftrightarrow x-\sqrt{x}+1>x\)
\(\Leftrightarrow x< 1\)
a: ĐKXĐ: \(x\ge0\)
Ta có: \(N=\left(\dfrac{x+2}{x\sqrt{x}+1}-\dfrac{1}{\sqrt{x}+1}\right)\cdot\dfrac{4\sqrt{x}}{3}\)
\(=\dfrac{x+2-x+\sqrt{x}-1}{\left(\sqrt{x}+1\right)\left(x-\sqrt{x}+1\right)}\cdot\dfrac{4\sqrt{x}}{3}\)
\(=\dfrac{4\sqrt{x}}{3x-3\sqrt{x}+3}\)

ĐKXĐ: \(\left\{{}\begin{matrix}x\ge0\\x\ne2\end{matrix}\right.\)
\(M=\dfrac{\left(\sqrt{x}-2\right)^2}{\left(\sqrt{x}+2\right)\left(\sqrt{x}-2\right)}-\dfrac{\left(\sqrt{x}+2\right)^2}{\left(\sqrt{x}+2\right)\left(\sqrt{x}-2\right)}\)
\(M=\dfrac{-8\sqrt{x}}{x-4}\)
\(M< 0\Leftrightarrow-\dfrac{8\sqrt{x}}{x-4}< 0\Leftrightarrow x-4>0\Leftrightarrow x>4\)

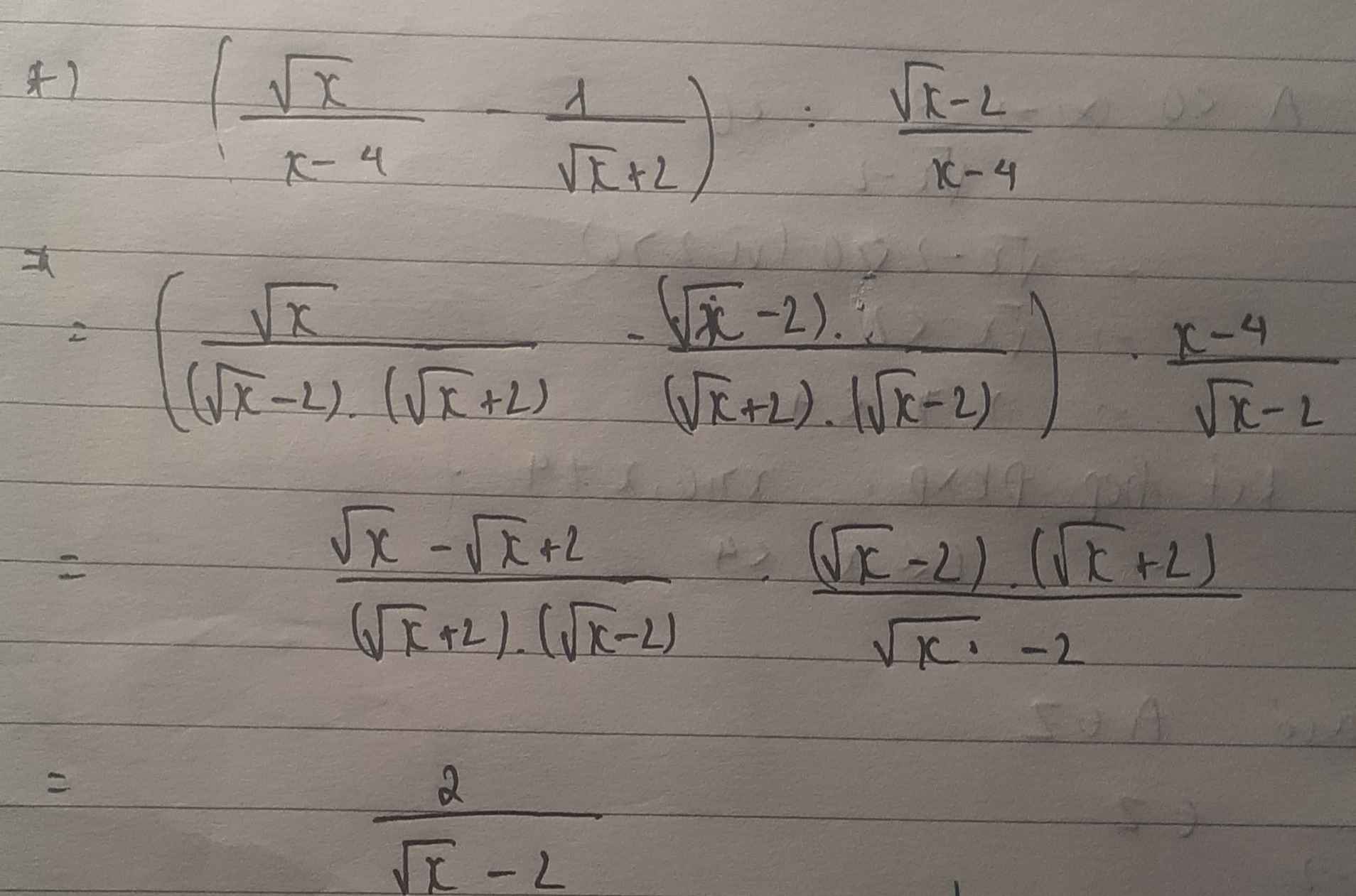
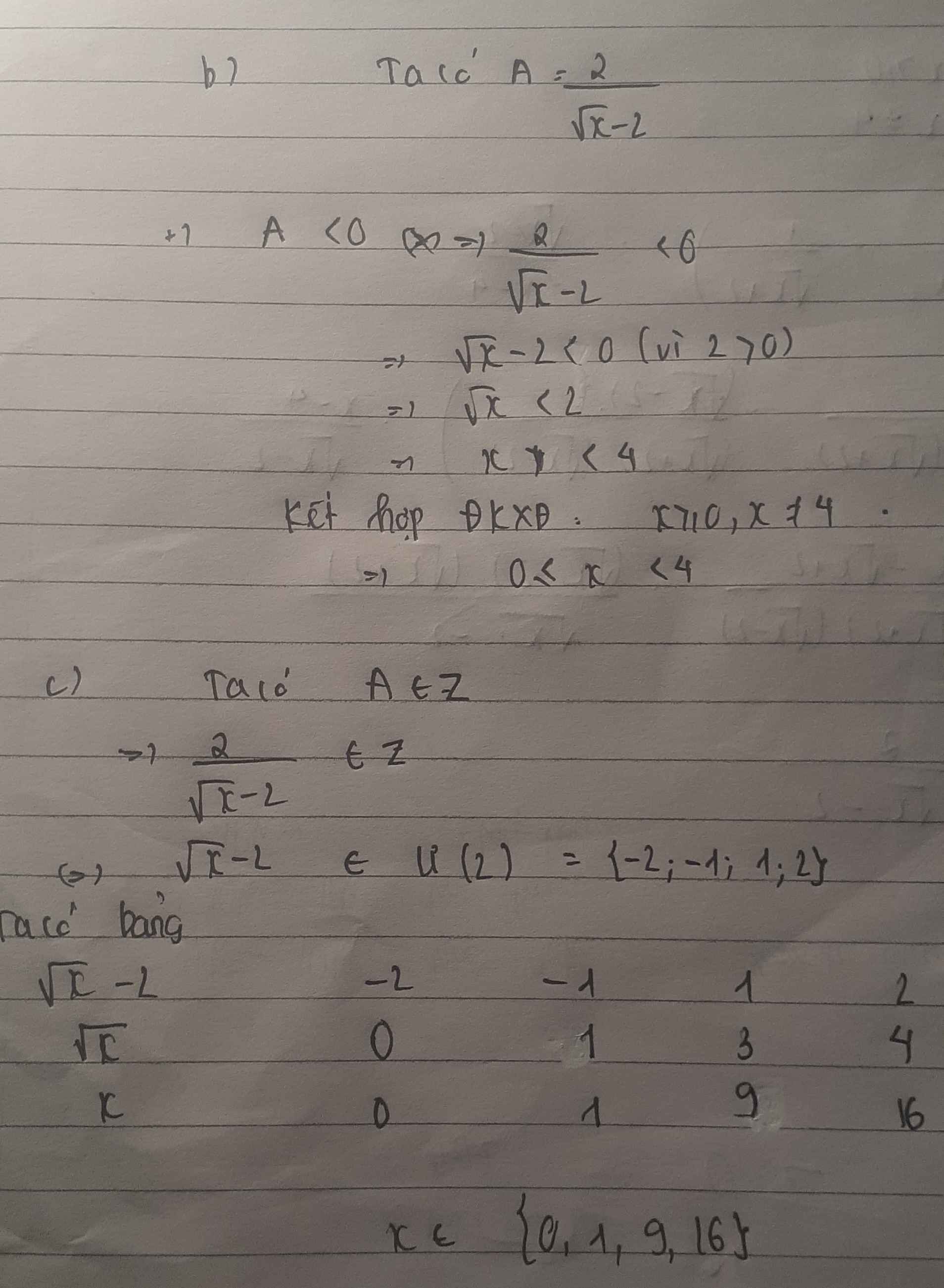
\(P=\dfrac{A}{B}=\sqrt{x}+1\)
P<7/4
=>căn x<3/4
=>0<x<9/16