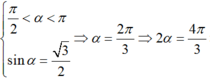Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Vì \(\dfrac{\pi}{2}< \alpha< \pi\) \(\Rightarrow\) cos \(\alpha\) < 0
\(\Rightarrow\) cos \(\alpha\) = \(-\sqrt{1-sin^2\alpha}\) = \(-\dfrac{2\sqrt{2}}{3}\)
\(\Rightarrow\) tan \(\alpha\) = \(\dfrac{sin\alpha}{cos\alpha}=\dfrac{-\sqrt{2}}{4}\)
\(\Rightarrow\) cot \(\alpha\) = \(\dfrac{1}{tan\alpha}\) = \(-2\sqrt{2}\)
Chúc bn học tốt!

Bài 1 :
Ta có : a thuộc góc phần tư thứ II .
=> Cos a < 0
- Ta lại có : \(\left\{{}\begin{matrix}sina=\dfrac{1}{3}\\sin^2a+cos^2a=1\end{matrix}\right.\)
\(\Rightarrow cosa=\sqrt{1-\left(\dfrac{1}{3}\right)^2}=-\dfrac{2\sqrt{2}}{3}\)
Bài 2 :
Ta có : \(F=\dfrac{\cos x.\tan x}{\sin^2x-\cot x.\cos x}=\dfrac{\cos x.\dfrac{\sin x}{\cos x}}{\sin^2x-\dfrac{\cos x}{\sin x}.\cos x}\)
\(=\dfrac{\sin x}{\sin^2x-\dfrac{\cos^2x}{\sin x}}=\dfrac{1}{\sin x-\cot^2x}\)

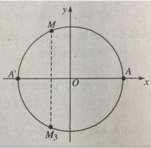
-π = -3,14; -2π = -6,28; (-5π)/2 = -7,85.
Vậy (-5π)/2 < -6,32 < -2π.
Do đó điểm M nằm ở góc phần tư thứ II.
Đáp án: B

Lời giải:
$\cos^2 a=1-\sin^2a=1-(\frac{3}{5})^2=\frac{16}{25}$
$\Rightarrow \cos a=\pm \frac{4}{5}$
Ta có:
\(\cos (a-\frac{\pi}{3})=\cos a\cos \frac{\pi}{3}-\sin a\sin \frac{\pi}{3}\)
\(=\frac{1}{2}\cos a-\frac{3\sqrt{3}}{10}=\frac{1}{2}.\pm \frac{4}{5}-\frac{3\sqrt{3}}{10}\)

Chọn B.
Theo giả thiết ta có: