TP
1
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
12 tháng 12 2021
Bài 1:
\(c,\text{PT có 2 }n_0\text{ phân biệt }\Leftrightarrow\Delta'=2^2-2m>0\Leftrightarrow2m< 4\Leftrightarrow m< 2\)
AH
Akai Haruma
Giáo viên
17 tháng 8 2021
Lời giải:
a. ĐKXĐ: $x^3-x\neq 0$
$\Leftrightarrow x(x-1)(x+1)\neq 0$
$\Leftrightarrow x\neq 0;\pm 1$
Vậy TXĐ: \(D=\mathbb{R}\setminus \left\{0;\pm 1\right\}\)
b.
ĐKXĐ: \(\left\{\begin{matrix} x\geq 0\\ |x|-1\neq 0\end{matrix}\right.\Leftrightarrow \left\{\begin{matrix} x\geq 0\\ x\neq \pm 1\end{matrix}\right.\Leftrightarrow \left\{\begin{matrix} x\geq 0\\ x\neq 1\end{matrix}\right.\)
TXĐ:
\([0;+\infty)\setminus \left\{1\right\}\)
c.
ĐKXĐ: \(x^2-1\neq 0\Leftrightarrow x\neq \pm 1\)
TXĐ: \(\mathbb{R}\setminus \left\{\pm 1\right\}\)
TN
1
AH
Akai Haruma
Giáo viên
29 tháng 1 2023
Lời giải:
$y=x^2-4x+m+5=x(x-3)-(x-3)+m+2$
$=(x-1)(x-3)+m+2$
Với $x\in [3;7]$ thì $(x-1)(x-3)\geq 0$
$\Rightarrow y\geq m+2$
Vậy $y_{\min}=m+2=10$
$\Leftrightarrow m=8$
23 tháng 10 2021
Câu 94: B
Câu 95: \(A=\left(-\dfrac{b}{2a};-\dfrac{b^2-4ac}{4a}\right)\)
\(\Leftrightarrow A\left(\dfrac{-2}{2\cdot\left(-1\right)};\dfrac{-\left(2^2-4\cdot\left(-1\right)\cdot3\right)}{4\cdot\left(-1\right)}\right)\)
\(\Leftrightarrow A\left(1;4\right)\)
NV
Nguyễn Việt Lâm
Giáo viên
7 tháng 1 2021
a.
\(y=\dfrac{4}{x}+\dfrac{1}{1-x}-1\ge\dfrac{\left(2+1\right)^2}{x+1-x}-1=8\)
\(y_{min}=8\) khi \(x=\dfrac{4}{5}\)
b.
\(y=\dfrac{1}{x}+\dfrac{1}{1-x}\ge\dfrac{4}{x+1-x}=4\)
\(y_{min}=4\) khi \(x=\dfrac{1}{2}\)
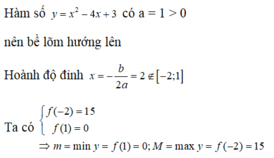
đề có sai không bạn,tại một trong hai thì phải có một cái không âm,một cái âm trên cái khoảng chứ phải hôn:<
còn chỉ tìm gtnn hay gtln thì chỉ tìm x = -b/2a rồi thế vào được nha