Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

b) Ta có: \(B=5x\left(x-4y\right)-4y\left(y-5x\right)\)
\(=5x^2-20xy-4y^2+20xy\)
\(=5x^2-4y^2\)
\(=5\cdot\left(-\dfrac{1}{5}\right)^2-4\cdot\left(-\dfrac{1}{2}\right)^2\)
\(=5\cdot\dfrac{1}{25}-4\cdot\dfrac{1}{4}\)
\(=\dfrac{1}{5}-1=\dfrac{-4}{5}\)

b) Tại x=14 thì:\(B\left(x\right)=x^5-15x^4+16x^3-29x^2+13x\)
\(=x^5-\left(x+1\right)x^4+\left(x+2\right)x^3-\left(2x+1\right)x^2+x\left(x-1\right)\)
\(=x^5-x^5-x^4+x^4+2x^3-2x^3-x^2+x^2-x=-x=-14\)
a) A(x)=1

a) Thu gọn M = - 5 a 2 từ đó tính được M = -125.
b) Gợi ý 15 = x + 1; 16 = x + 2; 29 = 2x + 1; 13 = x – 1.
Rút gọn N = -x, từ đó tính được N = -14.

a: Ta có: x=31
nên x-1=30
Ta có: \(A=x^3-30x^2-31x+1\)
\(=x^3-x^2\left(x-1\right)-x^2+1\)
\(=x^3-x^3+x^2-x^2+1\)
=1
c: Ta có: x=16
nên x+1=17
Ta có: \(C=x^4-17x^3+17x^2-17x+20\)
\(=x^4-x^3\left(x+1\right)+x^2\left(x+1\right)-x\left(x+1\right)+20\)
\(=x^4-x^4-x^3+x^3+x^2-x^2-x+20\)
\(=20-x=4\)
d: Ta có: x=12
nên x+1=13
Ta có: \(D=x^{10}-13x^9+13x^8-13x^7+...+13x^2-13x+10\)
\(=x^{10}-x^9\left(x+1\right)+x^8\left(x+1\right)-x^7\left(x+1\right)+...+x^2\left(x+1\right)-x\left(x+1\right)+10\)
\(=10-x\)
=-2

d: Ta có: x=12
nên x+1=13
Ta có: \(D=x^{10}-13x^9+13x^8-13x^7+...+13x^2-13x+10\)
\(=x^{10}-x^9\left(x+1\right)+x^8\left(x+1\right)-x^7\left(x+1\right)+...+x^2\left(x+1\right)-x\left(x+1\right)+10\)
\(=x^{10}-x^{10}-x^9+x^9+x^8-x^8-x^7+...+x^3+x^2-x^2-x+1+9\)
\(=-x+10=-2\)
các bạn giải hộ mình với :
a,X3 - 30X2 - 31X + 1 với X = 31
b,X5 - 15X4 + 16X3 - 29X2 + 13X với X = 14


Bài 2:
a: \(\Leftrightarrow\left(x-2\right)\left(x+2\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=2\\x=-2\end{matrix}\right.\)

1/
a/ \(D=2x\left(10x^2-5x-2\right)-5x\left(4x^2-2x-1\right)\)
\(D=2x\left[10\left(x^2-\frac{1}{2}x-\frac{1}{5}\right)\right]-5x\left[4\left(x^2-\frac{1}{2}x-\frac{1}{4}\right)\right]\)
\(D=20x\left(x^2-\frac{1}{2}x-\frac{1}{5}\right)-20x\left(x^2-\frac{1}{2}x-\frac{1}{4}\right)\)
\(D=20x^3-10x^2-4x-20x^3+10x^2+5x\)
\(D=x\)
b/ Mình xin sửa lại đề:
Tính giá trị biểu thức \(E\left(x\right)=x^5-13x^4+13x^3-13x^2+13x+2012\)
Tại x = 12
\(E\left(x\right)=x^5-\left(x+1\right)x^4+\left(x+1\right)x^3-\left(x+1\right)x^2+\left(x-1\right)x+2012\)
\(E\left(x\right)=x^5-x^5-x^4+x^4+x^3-x^3-x^2+x^2-x+2012\)
\(E\left(x\right)=2012-x\)
\(E\left(x\right)=2000\)
2/
a/ \(2x\left(x-5\right)-x\left(3+2x\right)=26\)
<=> \(2x^2-10x-3x-2x^2=26\)
<=> \(-13x=26\)
<=> \(x=-2\)
b/ Bạn vui lòng coi lại đề.
3a/ Ta có \(D=x\left(5x-3\right)-x^2\left(x-1\right)+x\left(x^2-6x\right)-10+3x\)
\(D=5x^2-3x-x^3+x^2+x^3-6x^2-10+3x\)
\(D=-10\)
Vậy giá trị của D không phụ thuộc vào x (đpcm)
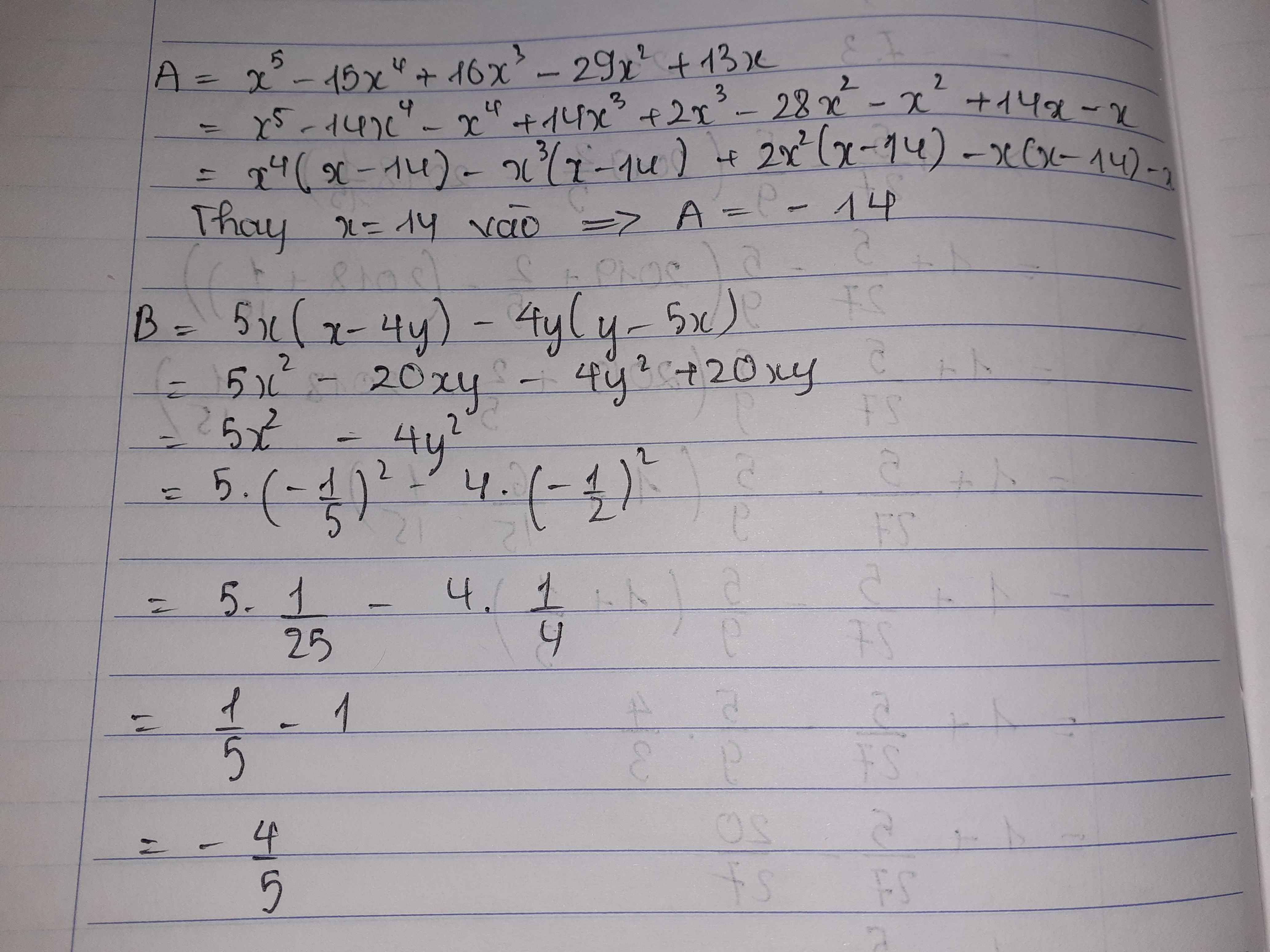


1. \(< =>\left(6x^2+31x+18\right)-\left(6x^2+13x+2\right)=x+1-a+6\)
\(< =>6x^2+31x+18-6x^2-13x-2=7\)
\(< =>18x+16=7\)
\(< =>18x=7-16\)
\(< =>18x=-9\)
\(< =>x=-\frac{9}{18}=-\frac{1}{2}\)
2. vì x=14 nên x+1=15 ; x+2 = 16; 2x + 1 =29; x-1=13
thay vào A ta được
\(A=x^5-\left(x+1\right)x^4+\left(x+2\right)x^3-\left(2x+1\right)x^2+\left(x-1\right)x\)
\(A=x^5-x^5-x^4+x^4+2x^3-2x^3-x^2+x^2-x\)
\(A=-x=-14\)