Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

\(c,\Rightarrow\left[{}\begin{matrix}-2\left(x+2\right)+\left(4-x\right)=11\left(x< -2\right)\\2\left(x+2\right)+\left(4-x\right)=11\left(-2\le x\le4\right)\\2\left(x+2\right)+\left(x-4\right)=11\left(x>4\right)\end{matrix}\right.\\ \Rightarrow\left[{}\begin{matrix}x=-\dfrac{11}{3}\left(tm\right)\\x=3\left(tm\right)\\x=\dfrac{11}{3}\left(ktm\right)\end{matrix}\right.\Rightarrow\left[{}\begin{matrix}x=3\\x=-\dfrac{11}{3}\end{matrix}\right.\)
\(a,\Rightarrow\left[{}\begin{matrix}x+\dfrac{5}{2}=3x+1\\x+\dfrac{5}{2}=-3x-1\end{matrix}\right.\Rightarrow\left[{}\begin{matrix}x=\dfrac{3}{4}\\x=-\dfrac{7}{8}\end{matrix}\right.\)

a: \(=-2x^2\cdot3x+2x^2\cdot4X^3-2x^2\cdot7+2x^2\cdot x^2\)
\(=8x^5+2x^4-6x^3-14x^2\)
b: \(=2x^3-3x^2-5x+6x^2-9x-15\)
\(=2x^3+3x^2-14x-15\)
c: \(=\dfrac{-6x^5}{3x^3}+\dfrac{7x^4}{3x^3}-\dfrac{6x^3}{3x^3}=-2x^2+\dfrac{7}{3}x-2\)
d: \(=\dfrac{\left(3x-2\right)\left(3x+2\right)}{3x+2}=3x-2\)
e: \(=\dfrac{2x^4-8x^3-6x^2-5x^3+20x^2+15x+x^2-4x-3}{x^2-4x-3}\)
=2x^2-5x+1

a) Ta có: \(5x^2-3x\left(x+2\right)\)
\(=5x^2-3x^2-6x\)
\(=2x^2-6x\)
b) Ta có: \(3x\left(x-5\right)-5x\left(x+7\right)\)
\(=3x^2-15x-5x^2-35x\)
\(=-2x^2-50x\)
c) Ta có: \(3x^2y\left(2x^2-y\right)-2x^2\left(2x^2y-y^2\right)\)
\(=3x^2y\left(2x^2-y\right)-2x^2y\left(2x^2-y\right)\)
\(=x^2y\left(2x^2-y\right)=2x^4y-x^2y^2\)
d) Ta có: \(3x^2\left(2y-1\right)-\left[2x^2\cdot\left(5y-3\right)-2x\left(x-1\right)\right]\)
\(=6x^2y-3x^2-\left[10x^2y-6x^2-2x^2+2x\right]\)
\(=6x^2y-3x^2-10x^2y+6x^2+2x^2-2x\)
\(=-4x^2y+5x^2-2x\)
e) Ta có: \(4x\left(x^3-4x^2\right)+2x\left(2x^3-x^2+7x\right)\)
\(=4x^4-16x^3+4x^4-2x^3+14x^2\)
\(=8x^4-18x^3+14x^2\)
f) Ta có: \(25x-4\left(3x-1\right)+7x\left(5-2x^2\right)\)
\(=25x-12x+4+35x-14x^3\)
\(=-14x^3+48x+4\)

\(F\left(x\right)=3x-6;x=\dfrac{6}{3}=2\)
\(H\left(x\right)=-5x+30;x=-\dfrac{30}{5}=-6\)
\(G\left(x\right)=\left(x-3\right)\left(16-4x\right)\Leftrightarrow\left[{}\begin{matrix}x-3=0;x=3\\16-4x=0;x=4\end{matrix}\right.\)
\(K\left(x\right)=x^2-81=\left(x-9\right)\left(x+9\right)\Leftrightarrow\left[{}\begin{matrix}x=-9\\x=9\end{matrix}\right.\)
\(M\left(x\right)=x^2+7x-8=\left(x-1\right)\left(x+8\right);\left[{}\begin{matrix}x=1\\x=-8\end{matrix}\right.\)
\(N\left(x\right)=5x^2+9x+4\)
\(N\left(x\right)=5x^2+5x+4x+4=5x\left(x+1\right)+4\left(x+1\right)\)
\(N\left(x\right)=\left(x+1\right)\left(5x+4\right)=0\Leftrightarrow\left[{}\begin{matrix}x=-1\\x=-\dfrac{4}{5}\end{matrix}\right.\)
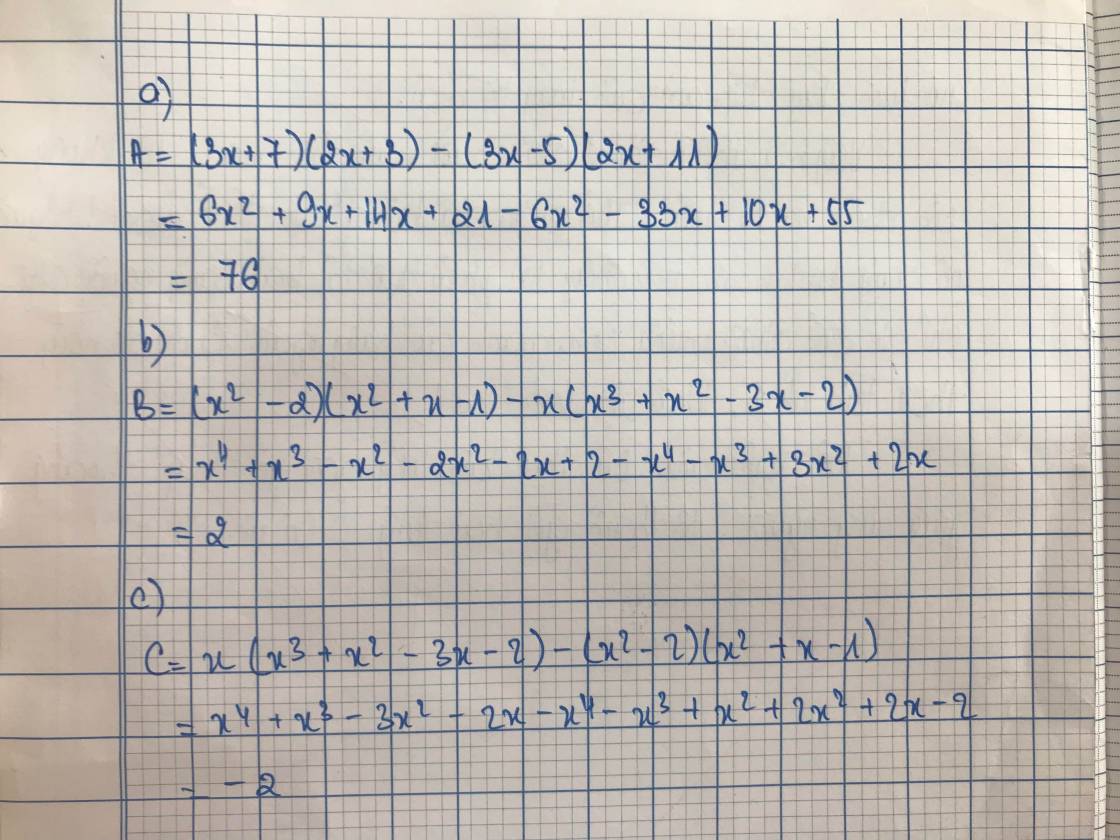
A=(x+1)(x+2)(x+3)(x+4)-24
=(x2+5x+4)(x2+5x+6)-24
Đặt t=(x2+5x+4) ta có:
t(t+2)-24=t2+6t-2t-24
=t(t+6)-4(t+6)
=(t-4)(t+6).Thay vào ta đc:
(x2+5x+4-4)(x2+5x+4+6)=(x2+5x)(x2+5x+10)
=x(x+5)(x2+5x+10)
B=(x2+3x+2)(x2+7x+120-24)
=(x2+3x+2)(x2+7x+96)
=(x2+2x+x+2)(x2+7x+96)
=[x(x+2)+(x+2)](x2+7x+96)
=(x+1)(x+2)(x2+7x+96)
C và D bn cx lm tương tự