Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Câu 2. Đặt A=x2+y2+1
Nhập \(2^A=\left(A-2x+1\right)4^x\) vào máy tính Casio. Cho x=0.01, tìm A
Máy sẽ giải ra, A=1.02=1+2x
\(\Leftrightarrow x^2+y^2+1=1+2x\)
\(\Leftrightarrow x^2+y^2-2x=1\)
\(\Leftrightarrow\left(x-1\right)^2+y^2=1\) (C)
Có (C) là đường tròn tâm (1,0) bán kính R=1
Lại có: P=\(\frac{8x+4}{2x-y+1}\)
\(\Leftrightarrow x\left(2P-8\right)-yP+P-4=0\) (Q)
Có (Q) là phương trình đường thẳng.
Để x,y có nghiệm thì đường thẳng và đường tròn giao nhau nghĩa là d(I,(Q))\(\le R\)
\(\Leftrightarrow\frac{\left|x\left(2P-8\right)-yP+P-4\right|}{\sqrt{\left(2P-8\right)^2+P^2}}\le1\)
\(\Leftrightarrow\frac{\left|2P-8+P-4\right|}{\sqrt{\left(2P-8\right)^2+1}}\le1\)
\(\Leftrightarrow\left(3P-12\right)^2\le5P^2-32P+64\)
\(\Leftrightarrow4P^2-40P+80\le0\)
\(\Leftrightarrow5-\sqrt{5}\le P\le5+\sqrt{5}\)
Vậy GTNN của P gần số 3 nhất. Chọn C

4.
\(xy+y=2\Leftrightarrow xy=2-y\Rightarrow x=\frac{2-y}{y}=\frac{2}{y}-1\)
\(\Rightarrow P=x+y^2=y^2+\frac{2}{y}-1\)
\(\Rightarrow P=y^2+\frac{1}{y}+\frac{1}{y}-1\ge3\sqrt[3]{\frac{y^2}{y.y}}-1=2\)
\(\Rightarrow P_{min}=2\) khi \(x=y=1\)

ĐKXĐ: \(-1< x< 2\)
Khi đó:
\(\Leftrightarrow log_2\left(2-x\right)\left(2x+2\right)-2log_2\left(m-\frac{x}{2}+4\left(\sqrt{2-x}+\sqrt{2x+2}\right)\right)\le0\)
\(\Leftrightarrow log_2\frac{\sqrt{\left(2-x\right)\left(2x+2\right)}}{m-\frac{x}{2}+4\left(\sqrt{2-x}+\sqrt{2x+2}\right)}\le0\)
\(\Rightarrow\frac{\sqrt{\left(2-x\right)\left(2x+2\right)}}{m-\frac{x}{2}+4\left(\sqrt{2-x}+\sqrt{2x+2}\right)}\le1\)
\(\Leftrightarrow\sqrt{\left(2-x\right)\left(2x+2\right)}\le m-\frac{x}{2}+4\left(\sqrt{2-x}+\sqrt{2x+2}\right)\)
\(\Leftrightarrow\sqrt{\left(2-x\right)\left(2x+2\right)}+\frac{x}{2}-4\left(\sqrt{2-x}+\sqrt{2x+2}\right)\le m\)
Đặt \(\sqrt{2-x}+\sqrt{2x+2}=t\Rightarrow\sqrt{3}\le t\le3\)
\(t^2=x+4+2\sqrt{\left(2-x\right)\left(2x+2\right)}\Rightarrow\sqrt{\left(2-x\right)\left(2x+2\right)}+\frac{x}{2}=\frac{t^2}{2}-2\)
\(\Rightarrow\frac{t^2}{2}-4t-2\le m\)
Xét hàm \(f\left(t\right)=\frac{t^2}{2}-4t-2\) trên \(\left[\sqrt{3};3\right]\)
\(\Rightarrow f\left(t\right)_{min}=f\left(3\right)=-\frac{19}{2}\Rightarrow m_{min}=-\frac{19}{2}\)

1.
\(y'=3x^2-3=0\Rightarrow\left[{}\begin{matrix}x=0\\x=1\end{matrix}\right.\)
\(y\left(0\right)=5;\) \(y\left(1\right)=3;\) \(y\left(2\right)=7\)
\(\Rightarrow y_{min}=3\)
2.
\(y'=4x^3-8x=0\Rightarrow\left[{}\begin{matrix}x=0\\x=-\sqrt{2}\end{matrix}\right.\)
\(f\left(-2\right)=-3\) ; \(y\left(0\right)=-3\) ; \(y\left(-\sqrt{2}\right)=-7\) ; \(y\left(1\right)=-6\)
\(\Rightarrow y_{max}=-3\)
3.
\(y'=\frac{\left(2x+3\right)\left(x-1\right)-x^2-3x}{\left(x-1\right)^2}=\frac{x^2-2x-3}{\left(x-1\right)^2}=0\Rightarrow x=-1\)
\(y_{max}=y\left(-1\right)=1\)
4.
\(y'=\frac{2\left(x^2+2\right)-2x\left(2x+1\right)}{\left(x^2+2\right)^2}=\frac{-2x^2-2x+4}{\left(x^2+2\right)^2}=0\Rightarrow\left[{}\begin{matrix}x=1\\x=-2\end{matrix}\right.\)
\(y\left(1\right)=1\) ; \(y\left(-2\right)=-\frac{1}{2}\Rightarrow y_{min}+y_{max}=-\frac{1}{2}+1=\frac{1}{2}\)

Bạn xem lại đề nghen, đoạn thỏa mãn đó có vấn đề phải không nhỉ?
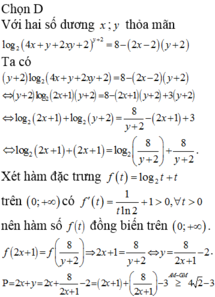
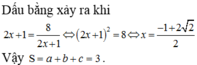
Bài 2: Mình nghĩ điều kiện sửa thành $a,b\in\mathbb{N}$ thôi thì đúng hơn.
ĐKĐB $\Leftrightarrow \log_2[(2x+1)(y+2)]^{y+2}=8-(2x-2)(y+2)$
$\Leftrightarrow (y+2)\log_2[(2x+1)(y+2)]=8-(2x-2)(y+2)$
$\Leftrightarrow (y+2)[\log_2[(2x+1)(y+2)]+(2x-2)]=8$
$\Leftrightarrow \log_2[(2x+1)(y+2)]+(2x-2)]=\frac{8}{y+2}$
$\Leftrightarrow \log_2(2x+1)+\log_2(y+2)+(2x+1)-3=\frac{8}{y+2}$
$\Leftrightarrow \log_2(2x+1)+(2x+1)=\frac{8}{y+2}+3-\log_2(y+2)=\frac{8}{y+2}+\log_2(\frac{8}{y+2})(*)$
Xét hàm $f(t)=\log_2t+t$ với $t>0$
$f'(t)=\frac{1}{t\ln 2}+1>0$ với mọi $t>0$
Do đó hàm số đồng biến trên TXĐ
$\Rightarrow (*)$ xảy ra khi mà $2x+1=\frac{8}{y+2}$
$\Leftrightarrow 8=(2x+1)(y+2)$
Áp dụng BĐT AM-GM:
$8=(2x+1)(y+2)\leq \left(\frac{2x+1+y+2}{2}\right)^2$
$\Rightarrow 2\sqrt{2}\leq \frac{2x+y+3}{2}$
$\Rightarrow 2x+y\geq 4\sqrt{2}-3$
Vậy $P_{\min}=4\sqrt{2}-3$
$\Rightarrow a=4; b=2; c=-3$
$\Rightarrow a+b+c=3$
Đáp án B.
2.
\(\Leftrightarrow\left(y+2\right)log_2\left(2x+1\right)\left(y+2\right)=8-\left(2x-2\right)\left(y+2\right)\)
\(\Leftrightarrow log_2\left(2x+1\right)\left(y+2\right)=\frac{8}{y+2}-2x+2\)
\(\Leftrightarrow log_2\left(2x+1\right)+log_2\left(y+2\right)=\frac{8}{y+2}-2x+2\)
\(\Leftrightarrow log_2\left(2x+1\right)+\left(2x+1\right)=-log_2\left(y+2\right)+3+\frac{8}{y+2}\)
\(\Leftrightarrow log_2\left(2x+1\right)+\left(2x+1\right)=log_2\left(\frac{8}{y+2}\right)+\frac{8}{y+2}\)
Xét hàm \(f\left(t\right)=log_2t+t\Rightarrow f'\left(t\right)=\frac{1}{t.ln2}+1>0;\forall t>0\)
\(\Rightarrow f\left(t\right)\) đồng biến \(\Rightarrow2x+1=\frac{8}{y+2}\)
\(\Rightarrow2x=\frac{8}{y+2}-1=\frac{6-y}{y+2}\)
\(\Rightarrow P=2x+y=y+\frac{6-y}{y+2}=y+\frac{8}{y+2}-1\)
\(\Rightarrow P=y+2+\frac{8}{y+2}-3\ge2\sqrt{\frac{8\left(y+2\right)}{y+2}}-3=4\sqrt{2}-3\)
\(\Rightarrow\left\{{}\begin{matrix}a=4\\b=2\\c=-3\end{matrix}\right.\) \(\Rightarrow a+b+c=3\)