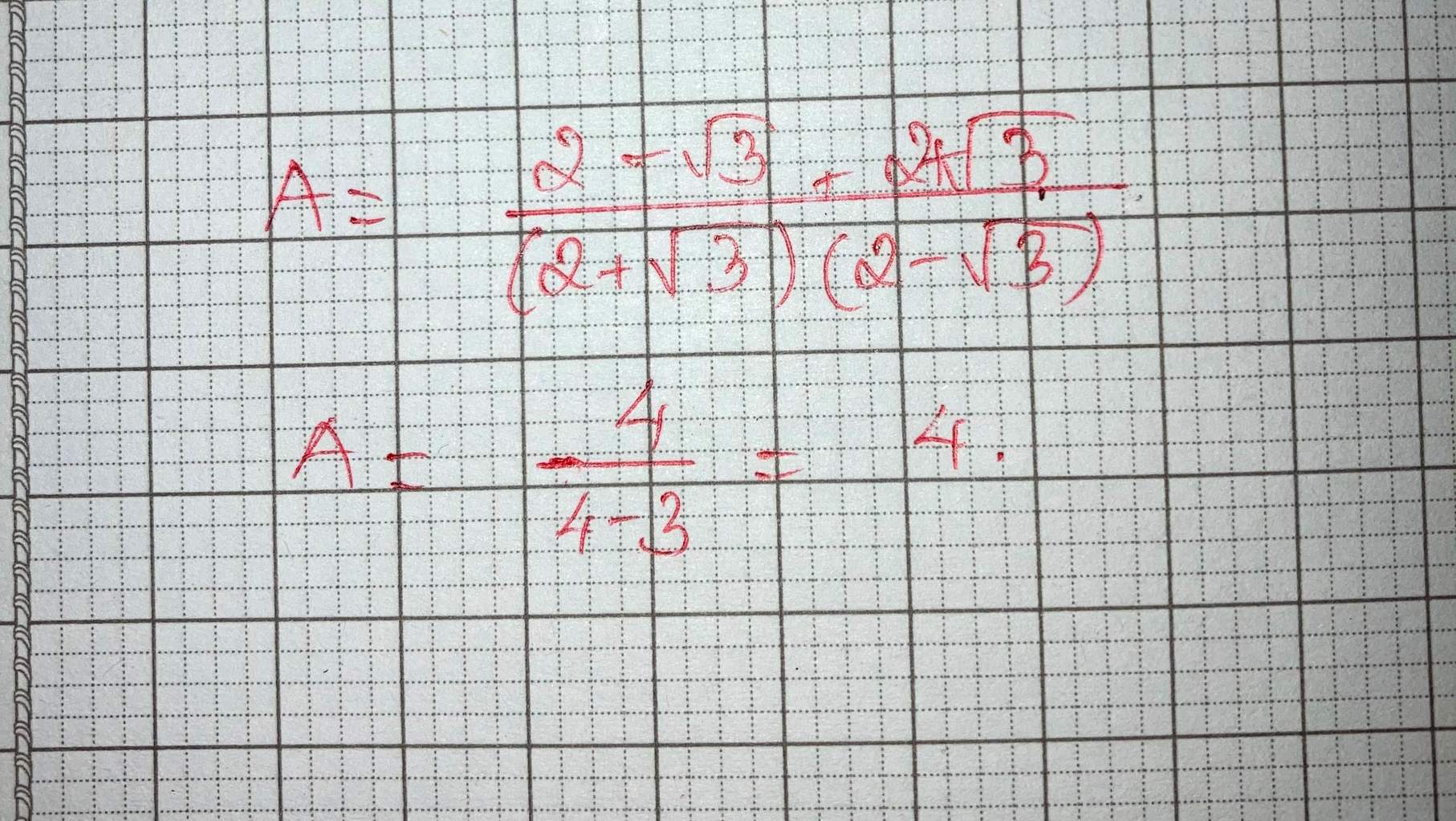rút gọn biểu thức:a= 1-2+2^2-2^3+2^4-...-2^99+2^100
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
5 tháng 6 2021
`A=sqrt{1+1/a^2+1/(a+1)^2}`
`=sqrt{1/a^2+2/a+1-2/a+1/(a+1)^2}`
`=sqrt{(1/a+1)^2-2/a+1/(a+1)^2}`
`=sqrt{(a+1)^2/a^2-2.(a+1)/a.(1/(a+1))+1/(a+1)^2}`
`=sqrt{((a+1)/a-1/(a+1))^2}`
`=|(a+1)/a-1/(a+1)|`
`=|1+1/a-1/(a+1)|`
`a>0=>1/a>1/(a+1)=>1+1/a-1/(a+1)>0`
`=>A=1+1/a-1/(a+1)`
5 tháng 6 2021
Áp dụng công thức ở A ta tính được
`B=1+1/1-1/2+1+1/2-1/3+1-1/3+1/4+.......+1+1/(n-1)-1/n`(ở sau bạn không ghi rõ nên mình đặt số cuối là n)
`=underbrace{1+1+....+1}_{\text{n chữ số 1}}-1/n`
`=n-1/n`
3 tháng 2 2019
a,M=2^0-2^1+2^2-2^3+2^4-2^5+.....+2^2012
2M=2^1-2^2+2^3-2^4+2^5-2^5+......-2^2012+2^2013
3M=2^0+2^2013
M=(2^0+2^2013)÷3
Vậy.......
b,N=3-3^2+3^3-3^4+3^5-3^6+.....+3^2011-3^2012
3N=3^2-3^3+3^4-3^5+3^6-3^7+......+3^2012-3^2013
4N=3-3^2013
N=(3-3^2013)÷4
Vậy........
K tao nhé ko lên lớp tao đánh m😈😈😈
31 tháng 8 2021
Câu 3:
a: \(49^2=2401\)
b: \(51^2=2601\)
c: \(99\cdot100=9900\)
29 tháng 6 2021
`a)2sqrt{48}-4sqrt{27}+sqrt{75}+sqrt{12}`
`=8sqrt3-12sqrt3+5sqrt3+2sqrt3`
`=3sqrt3`
`b)sqrt{(3-sqrt5)^2}-sqrt{20}`
`=3-sqrt5-2sqrt5`
`=3-3sqrt5`
2 câu cuối không rõ đề :v
TT
4
11 tháng 2 2022
đk : x >= 0 ; x khác 4
\(A=\dfrac{2\sqrt{x}-4-\sqrt{x}-2+4}{x-4}=\dfrac{\sqrt{x}-2}{x-4}=\dfrac{1}{\sqrt{x}+2}\)
TG
11 tháng 2 2022
ĐKXĐ: x khác 4; x ≥ 0
\(A=\dfrac{2\sqrt{x}-4-\sqrt{x}-2+4}{x-4}=\dfrac{\sqrt{x}-2}{\left(\sqrt{x}-2\right)\left(\sqrt{x}+2\right)}=\dfrac{1}{\sqrt{x}+2}\)
VP
1
11 tháng 6 2021
Với n\(\in N\)* có: \(\dfrac{1}{\left(n+1\right)\sqrt{n}+n\sqrt{n+1}}=\dfrac{1}{\sqrt{n\left(n+1\right)}\left(\sqrt{n+1}+\sqrt{n}\right)}\)\(=\dfrac{\sqrt{n+1}-\sqrt{n}}{\sqrt{n\left(n+1\right)}\left(n+1-n\right)}=\dfrac{\sqrt{n+1}-\sqrt{n}}{\sqrt{n\left(n+1\right)}}\)\(=\dfrac{1}{\sqrt{n}}-\dfrac{1}{\sqrt{n+1}}\)
\(\Rightarrow\)\(\dfrac{1}{\left(n+1\right)\sqrt{n}+n\sqrt{n+1}}=\dfrac{1}{\sqrt{n}}-\dfrac{1}{\sqrt{n+1}}\) (*)
a) Áp dụng (*) vào T
\(\Rightarrow T=1-\dfrac{1}{\sqrt{2}}+\dfrac{1}{\sqrt{2}}-\dfrac{1}{\sqrt{3}}+...+\dfrac{1}{\sqrt{99}}-\dfrac{1}{\sqrt{100}}\)\(=1-\dfrac{1}{10}=\dfrac{9}{10}\)
b) Có \(VT=1-\dfrac{1}{\sqrt{2}}+\dfrac{1}{\sqrt{2}}-\dfrac{1}{\sqrt{3}}+...+\dfrac{1}{\sqrt{n}}-\dfrac{1}{\sqrt{n+1}}\)\(=1-\dfrac{1}{\sqrt{n+1}}=\dfrac{4}{5}\)
\(\Leftrightarrow\sqrt{n+1}=5\Leftrightarrow n=24\) (tm)
Vậy n=24.
